Let ABCD be a traezium with AD parallel to BC. Assume there is a point M in the interior of the segement BC such that AB=AM and DC=DM . Then the ratio of the area of the tapezium to the area of triabgle AMD is -
2
3
4
Not determinable from data
The correct Answer is:B
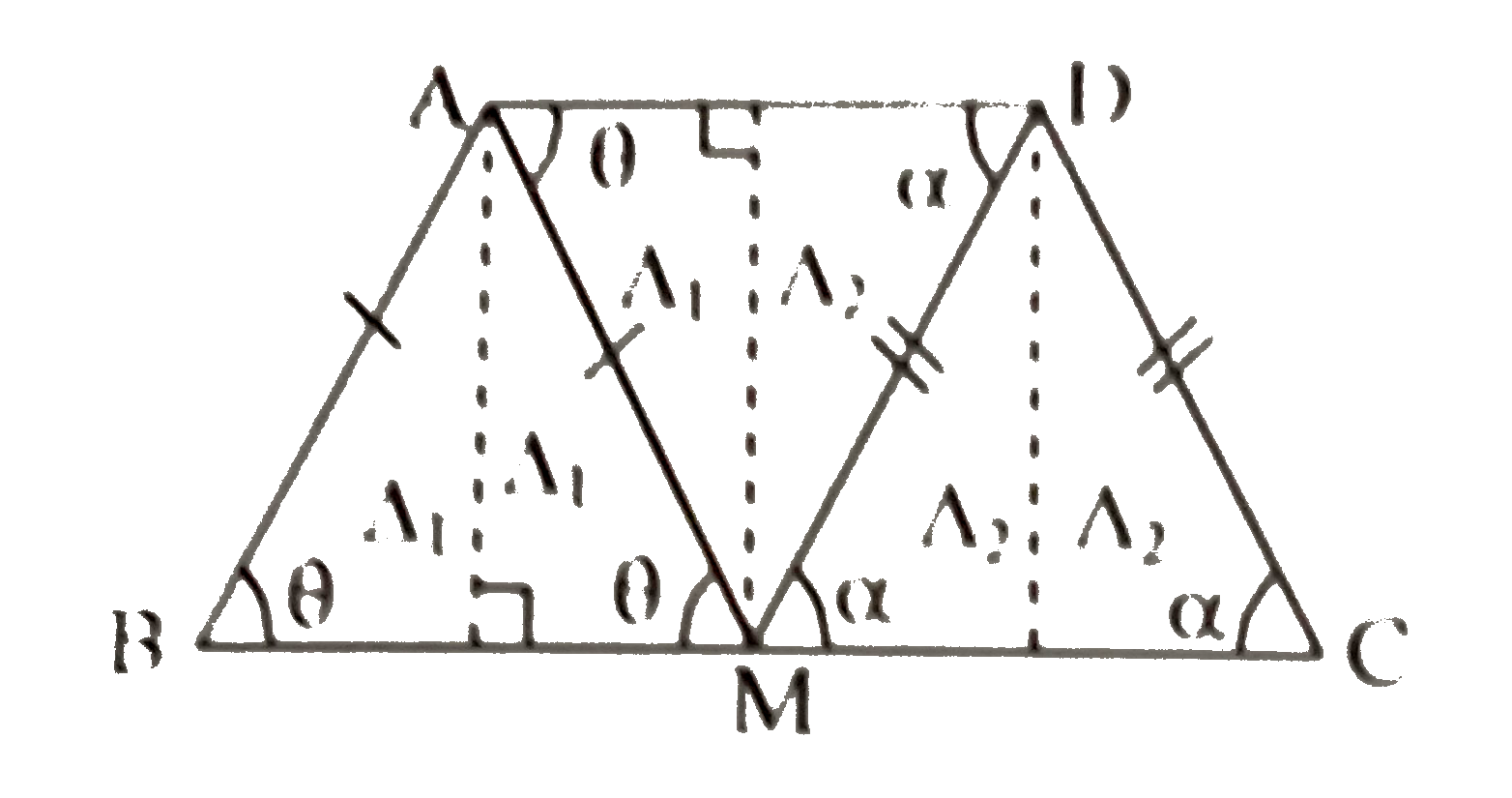
ar(ABCD)ar(AMD)=3Δ+3Δ2Δ1+Δ2=31
Similar Questions
ABCD is a parallelogram. E is a point on BC such that BE : EC = m:n. If AE and DB intersect in F, then what is the ratio of the area of triangle FEB to the area of triangle AFD?
View SolutionIn the adjoining figure, DE is parallel to BC and AD=1cm, BD=2cm. What is the ratio of the area of ABC to the area of ADE?
View Solution
Knowledge Check
ABCD is a trapezium in which AB∣∣CD and AB=2CD. It its diagonals intersect each other at O then ratio of the area of triangle AOB and COD is
A1:2B2:1C4:1D1:4In a trapezium ABCD, AB is a parallel to CD. BD is perpendicular to AD. AC is perpendicular to BC. If AD=BC=15cmandAB=25cm, then the area of the trapezium is :
A192cm2B232cm2C172cm2Dnone of theseLet ABCD be a trapezium in which AB||DC and E be the mid-point of AD. If F be a point on BC such that EF||AB. Then EF, where F is the mid-point of BC, is equal to
 AAB + DCB12(AB+DC)C13(AB+DC)DNone of these
AAB + DCB12(AB+DC)C13(AB+DC)DNone of these
ABCD is a trapezium, where AB is parallel to DC. If AB = 4cm, BC = 3 cm, CD = 7 cm and DA = 2cm, then what is the area of the trapezium?
View SolutionABCD is a trapezium with AB //DC. A line parallel to AC intersects AB at point M and BC at point N. Prove that: areaof△ADM=areaof△ACN.
View SolutionABCD is a kite in which AB=AD and BC= DC.
The kite is symmetrical about

View SolutionABCD is a kite in which AB=AD and BC= DC.
The kite is symmetrical about

View SolutionLet ABC be a triangle and M be a point on side AC closer to vertex C than A. Let N be a point on side AB such that MN is parallel to BC and let P be a point on side BC such that MP is parallel to AB. If the area of the quadrilateral BNMP is equal to 518th of the area of triangle ABC, then the ratio AM/MC equals.
View Solution
