Let ABCD be a square of side length 1, and I− a circle passing through B and C, and touching AD. The readius of I− is -
38
12
1√2
58
The correct Answer is:D
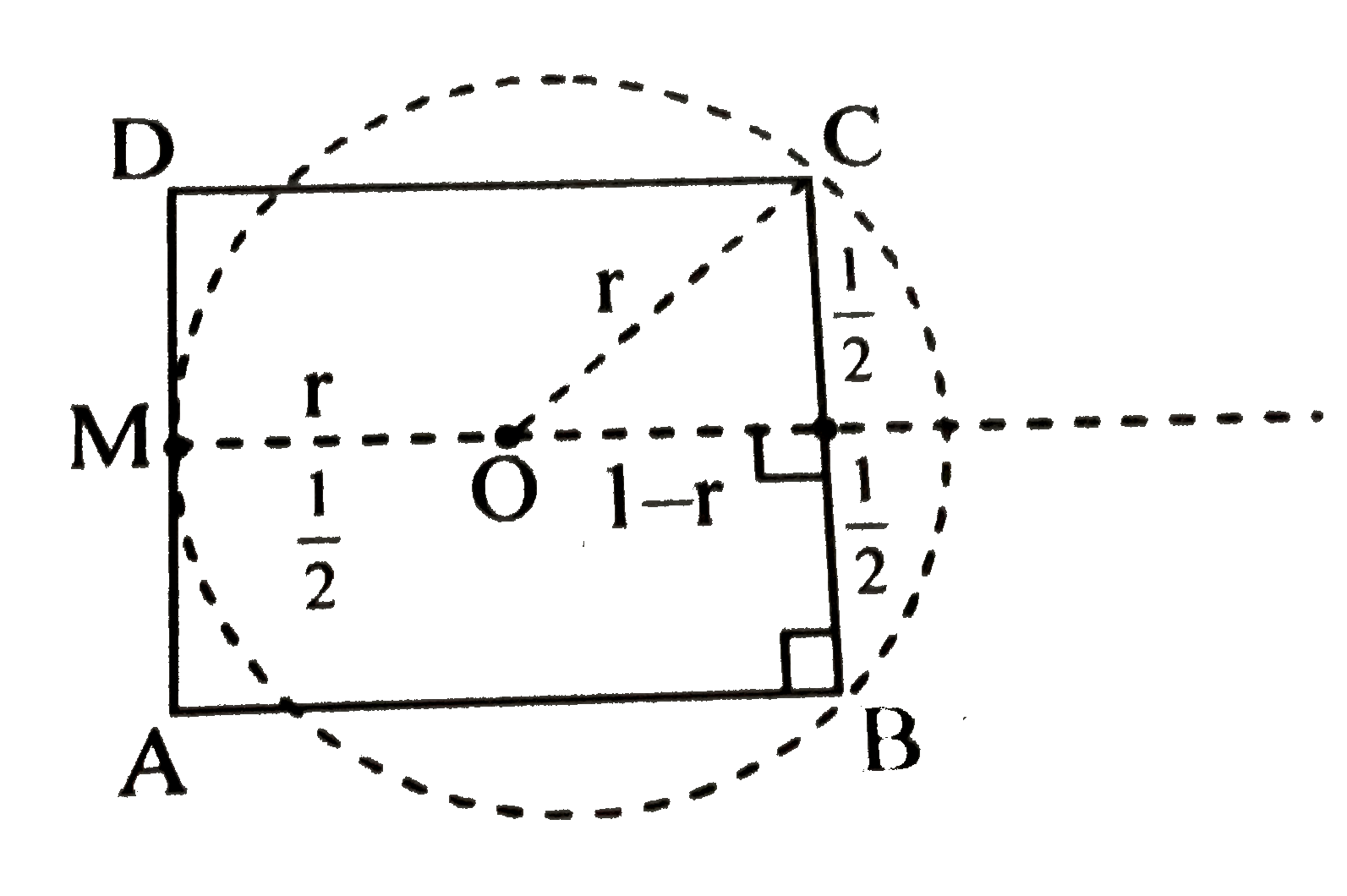
Let O be centre of circle .
OM = radius =r
∴r2(1−r)2+(12)2
⇒2r−1=14
⇒2r−1=14
⇒2r=54
r=58
Similar Questions
Let ABCD be a square of side length 2 units. C2 is the circle through vertices A, B, C, D and C1 is the circle touching all the sides of the square ABCD. L is a line through A
A line M through A is drawn parallel to BD. Point S moves such that its distances from the line BD and the vertex A are equal. If locus of S cuts. M at T2 and T3 and AC at T1, then area of ΔT1T2T3 is
View SolutionLet ABCD be a square of side length 2 units. C2 is the circle through vertices A, B, C, D and C1 is the circle touching all the sides of the square ABCD. L is a line through A
A line M through A is drawn parallel to BD. Point S moves such that its distances from the line BD and the vertex A are equal. If locus of S cuts. M at T2 and T3 and AC at T1, then area of ΔT1T2T3 is
View Solution
Knowledge Check
The equation of circle passing through (-1,-2) and touching both the axes is
Ax2+y2−2x−2y−1=0Bx2+y2+2x+2y+1=0Cx2+y2+10x+10y+25=0Dx2+y2−2x−2y+1=0Let ABCD be square of side length 2 unit. C2 is the circle through verticles A,B,C,D and C1 is the circle touching all the sides of square ABCD. L is the line through A. A circle touches the line L and the circle C1 exrternally such that both the circles are on the same side of the line, then the locus of centre of the circle is
AellipseBhyperbolaCparabolaDparts of straight lineLet ABCD be a square of side length 2 units. C2 is the fircle through the vertices A, B, C, D and C1 is the circle touching all the of the square ABCD. L is a lien through vertex A. A circle touches the line L and the circle C1 externally such that both the circles are on the same side of the line L. The locus of the centre of the circle is
AellipseBhyperbolaCparabolaDpair of straight lines
Let ABCD be a square of side length 2 units. C2 is the circle through vertices A,B,C,DandC1 is the circle touching all the sides of the square ABCD. L is a line through A. If P is a point on C1andQ in another point on C2, then PA2+PB2+PC2+PD2QA2+QB2+QC2+QD2 is equal to
View SolutionLet ABCD be a square of side length 2 units. C2 is the circle through vertices A, B, C, D and C1 is the circle touching all the sides of the square ABCD. L is a line through A. 27. If P is a point on C1 and Q in another point on C2, then 2222 2222 PA PB PC PD QA QB QC QD +++ +++ is equal to (A) 0.75 (B) 1.25 (C) 1 (D) 0.5
View SolutionABCD is a square with side length 10. A circle is drawn through A and D so that it is tangent to BC.

What is the radius of circle?
View SolutionThe equation of the circle passing through (2, 1) and touching co-ordinate axes is
View SolutionLet ABCD be a square of side length 2 units. C2 is the fircle through the vertices A, B, C, D and C1 is the circle touching all the of the square ABCD. L is a lien through vertex A. A circle touches the line L and the circle C1 externally such that both the circles are on the same side of the line L. The locus of the centre of the circle is
View Solution
