in a triangle ABC , points X and Y are on AB And AC , respectively , such that XY is parallel to BC . Which of the two following equalities always hold? (here [PQR] denotes the area of triangle PQR).
(i) [BCX]=[BCY]
(ii) [ACX].[ABY]=[AXY].[ABC]
Neither (i) nor (ii)
(i) only
(ii) only
Both (i) and (ii)
The correct Answer is:D
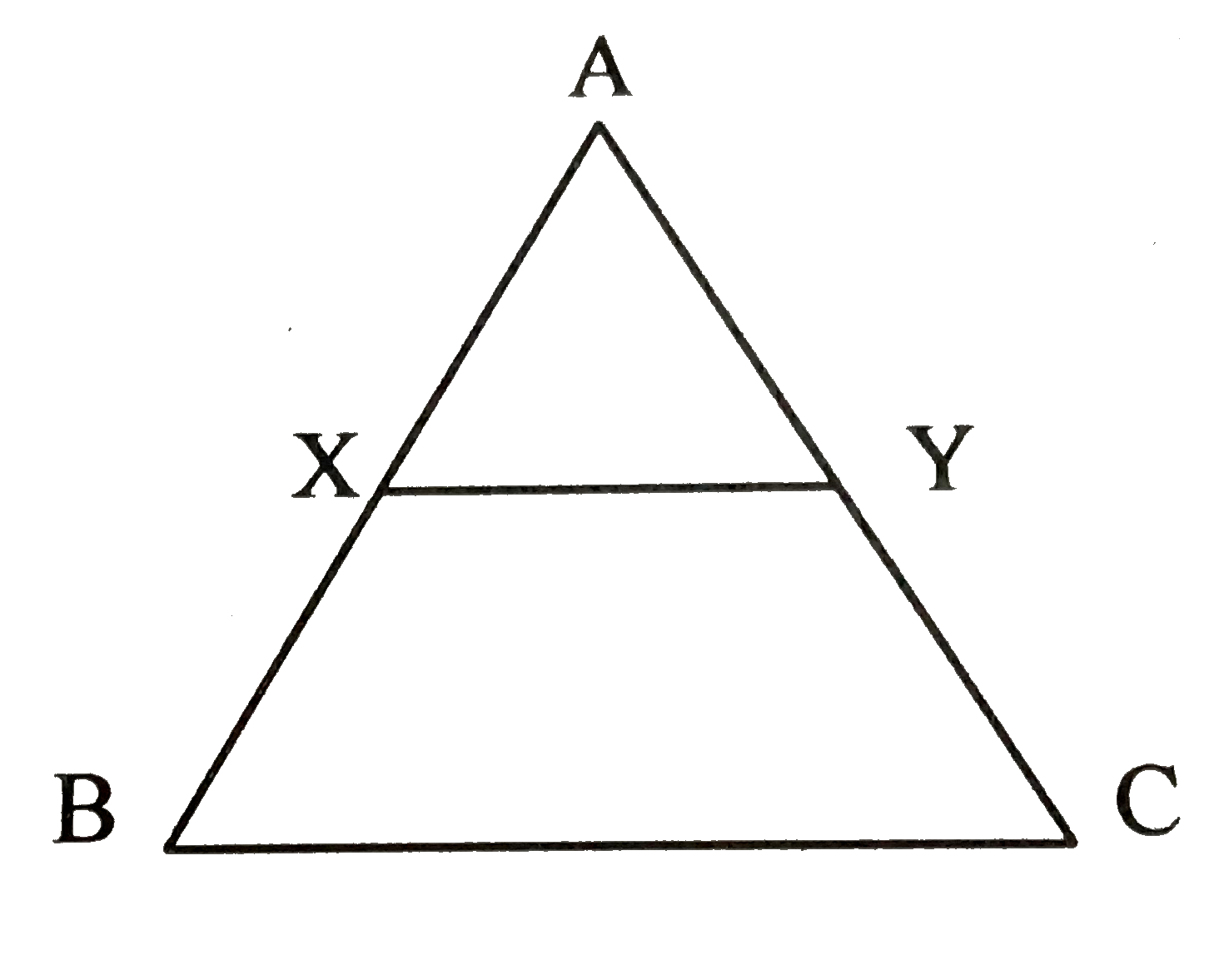
Clearly ar (BCX)=ar(BCY) {Δs between parallel lines & same base }
⇒[BCY]=[BCY]
(i) is true
check
(ii) ar (ΔACX)=12Ac.AXsinA
ar(ΔABY)=12AB.AYsinA
ar(ΔAXY)=12AX.AYsinA.
ar(ΔABC)=12AB.ACsinA.
Clearly [ACX].[ABY]=[AXY].[ABC]
true.
Similar Questions
In a triangle ABC, D and E are points on AB, AC respectively such that DE is parallel to BC. Suppose BE, CD intersect at O. If the areas of the triangles ADE and ODE are 3 and 1 respectively, find the area of the triangle ABC, with justification
View SolutionIn a triangle ABC, X and Y are points on the segments AB and AC, respectively, such that AX:XB=1:2 and AY:YC=2:1. If the area of triangle AXY is 10 then what is the area of triangle ABC?
View Solution
Knowledge Check
Area of ΔABC=30cm2. D and E are the mid - points of BC and AB respectively. Find A(ΔBDE) :
A10cm2B7.5cm2C15cm2Dnone of theseIn a triangle ABC, points P, Q and R are the mid-points of the sides AB, BC and CA respectively. If the area of the triangle ABC is 20 sq. units, find the area of the triangle PQR
A10 sq. unitsB5.3 sq. unitsC5 sq. unitsDNone of thesePoints P and Q lie on sides AB and AC of triangle ABC respectively such that segment PQ is parallel to side BC. If the ratio of areas of triangle APQ: triangle ABC is 25 : 36, then the ratio PA : PB is
A5:6B1:5C6:5D5:1
Suppose ABC is a triangle with AB of unit length. D and E are the points lying on AB and AC respectively such that BC and DE are parallel. If the area of triangle ABC is twice the area of triangle ADE, then the length of AD is
View SolutionIn a triangle ABC,XandY are the points on ABandBC respectively. If BX=12ABandBY=12BCandAB=BC. Show that BX=BY.
View SolutionD and E are points on sides AB and AC respectively of ΔABC such that ar(ΔDBC) = ar(ΔEBC). Prove that DE I I BC.
View SolutionIf a line intersects sides AB and AC of a ΔABCat D and E respectively and is parallel to BC, prove that ADAB=AEAC
View SolutionIn a triangle ABC, let G denote its centroid and let M, N be points in the interiors of the segments AB, AC, respectively, such that M, G, N are collinear. If r denotes the ratio of the area of triangle AMN to the area of ABC then
View Solution
