Among all cyclic quadrilaterals inscribed in a circle of radius R with one of its angles equal to 120∘. Consider the one with maximum possible area. Its area is-
A
√2R2
B
√3R2
C
2R2
D
2√3R2
Video Solution
Text Solution
The correct Answer is:B
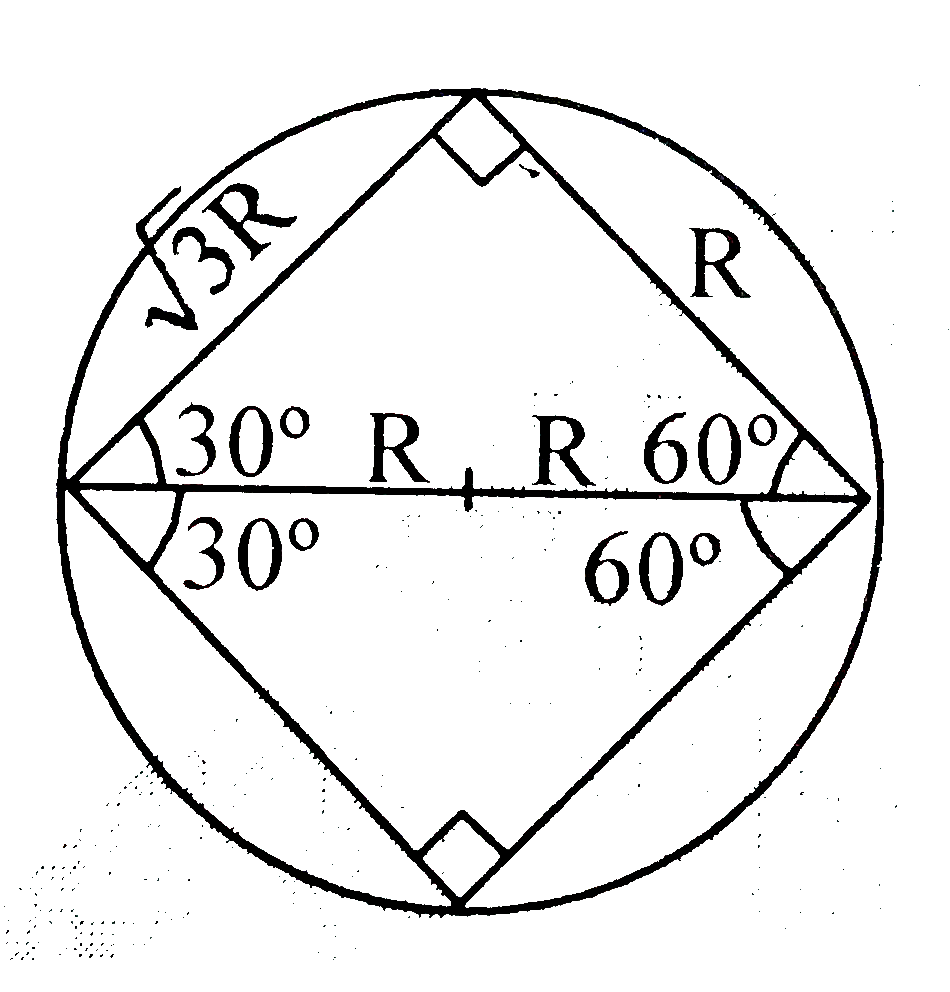
A=2×12×√3R×R=√3R2
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
If a regular hexagon is inscribed in a circle of radius r , then its perimeter is :
A6√3rB6rC3rD12rThe maximum area of rectangle, inscribed in a circle of radius 'r', is :
Aπr2Br2C2r2D14πr2If a triangle of maximum area is inscribed within a circle of radius R, then
 AS=2R2B1r1+1r2+1r3=√2+1RCr=(√2−1)RDs=(1+√2).2R
AS=2R2B1r1+1r2+1r3=√2+1RCr=(√2−1)RDs=(1+√2).2R
An equilateral triangle is inscribed in a circle of radius 'r' units. The area of shaded region is
View SolutionIf radius of circle if 4r, the area of circle is equal to :
View SolutionIf a triangle of maximum area is inscribed within a circle of radius R, then

View SolutionIf a regular hexagon circumscribes a circle of radius r , then its perimeter is :
View SolutionIf a regular hexagon circumscribes a circle of radius r , then its perimeter is :
View Solution