At the Point of Inflexion :

Total Product is maximum

Average Product is maximum

Marginal Product is maximum

Marginal Product is zero

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

Answer

Step by step video, text & image solution for At the Point of Inflexion : by Economics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 ECONOMICS PRODUCTION FUNCTION

Topper's Solved these Questions

PRODUCTION FUNCTIONBOOK - SANDEEP GARGCHAPTER - PRODUCTION FUNCTIONEXERCISE - Short Answer Type Questions
15 Videos
PRODUCTION FUNCTIONBOOK - SANDEEP GARGCHAPTER - PRODUCTION FUNCTIONEXERCISE - Long Answer Type Questions
12 Videos
PRODUCTION FUNCTIONBOOK - SANDEEP GARGCHAPTER - PRODUCTION FUNCTIONEXERCISE - Very Short Answer Type Question
25 Videos
PRODUCER'S EQUILIBRIUMBOOK - SANDEEP GARGCHAPTER - PRODUCER'S EQUILIBRIUMEXERCISE - Unsolved Practicals
12 Videos
REVENUE BOOK - SANDEEP GARGCHAPTER - REVENUE EXERCISE - UNSOLVED PRACTICALS
21 Videos

Knowledge Check

The sum of abscissa of the points of inflexions of curve $y =$
$f (x) = \frac{x^{7}}{42} - \frac{2 x^{6}}{15} + \frac{3 x^{5}}{20} + \frac{x^{4}}{3} - \frac{2 x^{3}}{3} + 99 x + 2010$ is,
A $- 1$
B $0$
C $2$
Dnone of these
Point of Inflexion' is represented by :

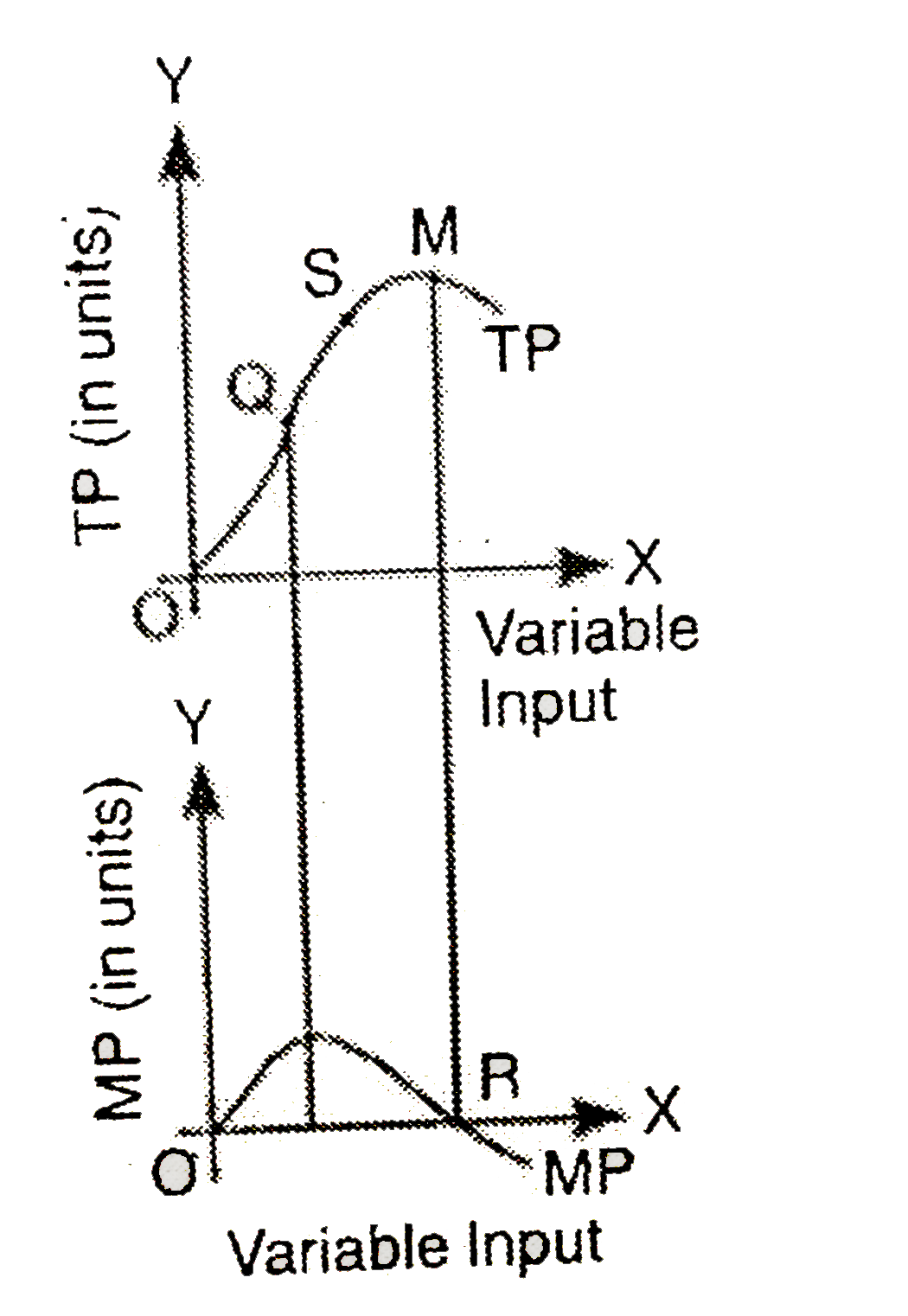APoint M
BPoint Q
CPoint R
DPoint S