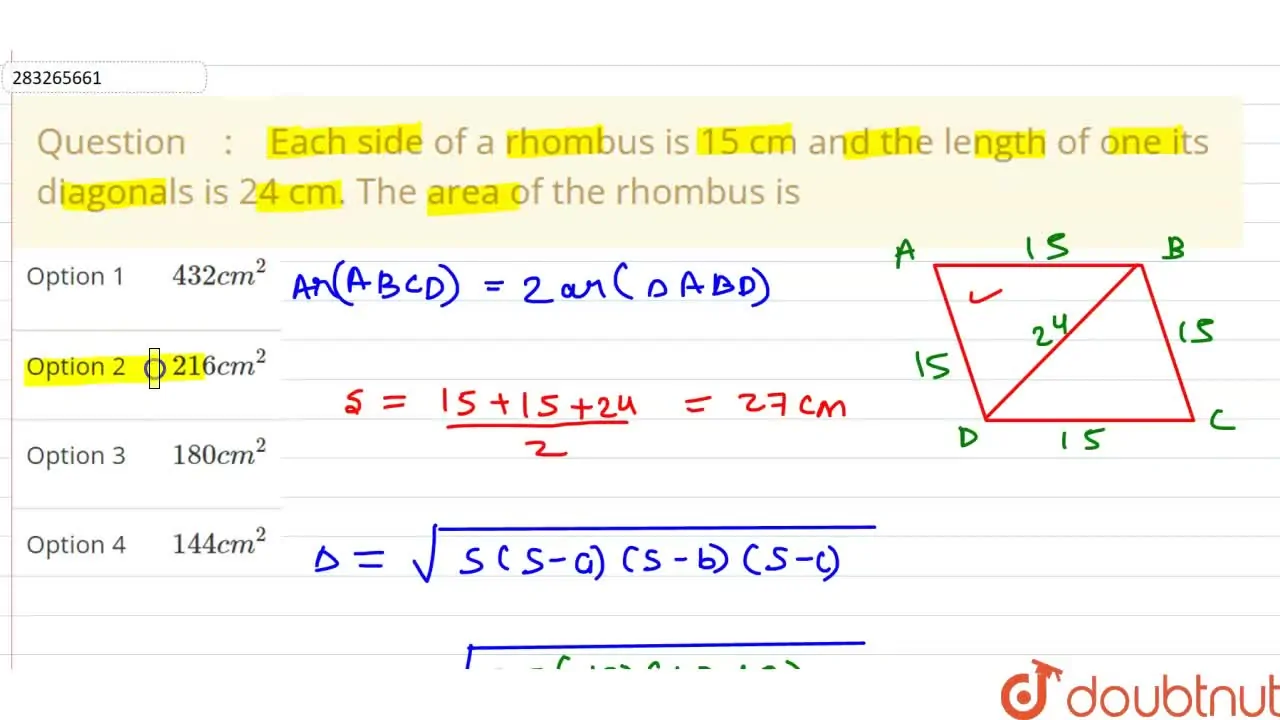
Each side of a rhombus is 15 cm and the length of one its diagonals is 24 cm. The area of the rhombus is
432cm2
216cm2
180cm2
144cm2
The correct Answer is:B
Step by step video, text & image solution for Each side of a rhombus is 15 cm and the length of one its diagonals is 24 cm. The area of the rhombus is by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Related Playlists
- BOOK - RS AGGARWALCHAPTER - AREA OF A TRAPEZIUM AND A POLYGON EXERCISE - TEST PAPER - 18 C (Fill in the blanks)1videos
AREA OF A TRAPEZIUM AND A POLYGON
- BOOK - RS AGGARWALCHAPTER - AREA OF A TRAPEZIUM AND A POLYGON EXERCISE - TEST PAPER - 18 A6videos
AREA OF A TRAPEZIUM AND A POLYGON
- BOOK - RS AGGARWALCHAPTER - COMPOUND INTEREST EXERCISE - TEST PAPER-11 ( Fill in the blanks )4videos
COMPOUND INTEREST
Similar Questions
If the side of a rhombus is 13 cm and the length its one diagonal is 24 cm. Find area of the rhombus.
View SolutionOne side of a rhombus is 10 cms and one of its diagonals is 12 cms . The area of the rhombus is -----
View SolutionOne side of a rhombus is 13 cm and one of its diagonals is 24 cm. What is the area of the rhombus?
View SolutionThe perimeter of a rhombus is 60 cm and one of its diagonal is 24 cm. The area of the rhombus is
View SolutionEach side of a rhombus is 26 cm and one of its diagonals is 48 cm long. The area of the rhombus is (a) 2400 cm2 (b) 3000 cm2 (c) 3600 cm2 (d) 4800 cm2
View SolutionThe side of a rhombus are 10 cm and one of its diagonals is 16 cm . The area of the rhombus is :
View SolutionThe side of a rhombus is 5 cm and one of its diagonal is 8 cm. What is the area of the rhombus ?
View SolutionThe side of a rhombus are 10 cm and one of its diagonals is 16 cm . The area of the rhombus is :
View SolutionOne side of a rhombus is 20 cm long and one of its digonals measures 24 cm. the area of the rhombus is
View SolutionThe area of a rhombus is 36 cm and the length of one of its diagonals is 6 cm. The length of the second diagonal is
View Solution