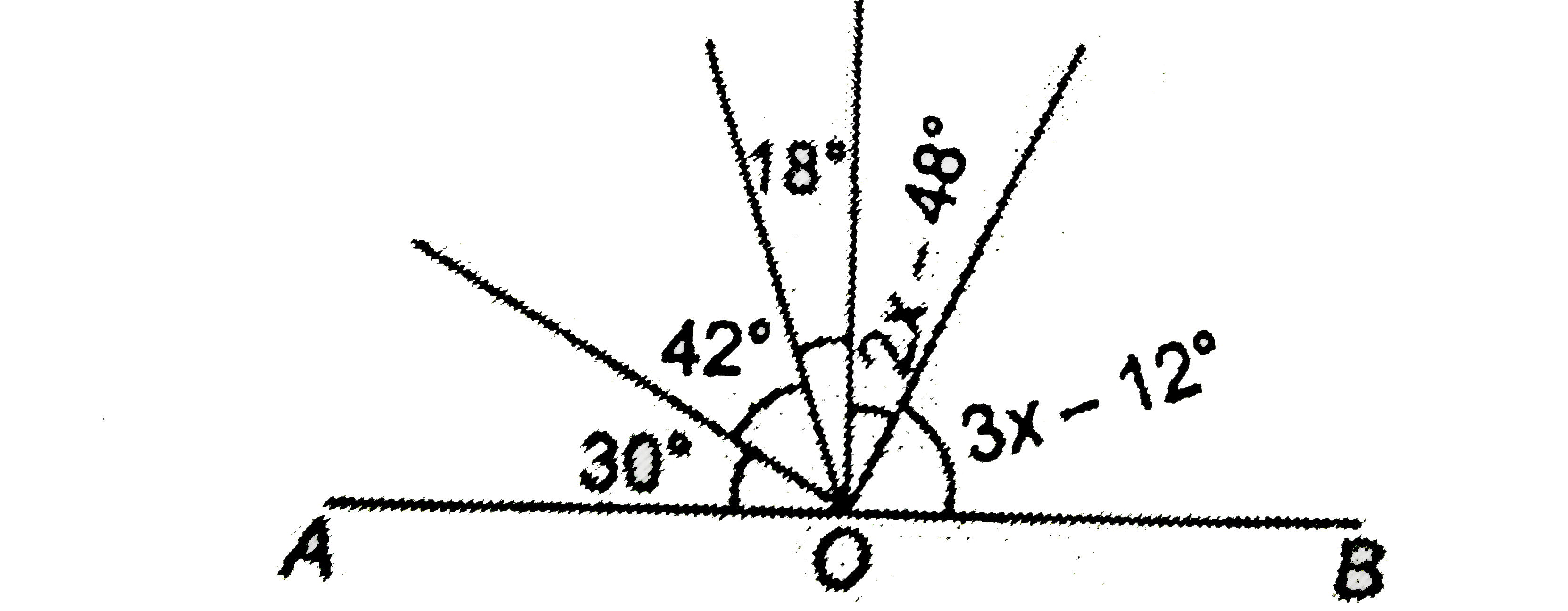
In the given figure, AOB is a straight line, ∠AOC = (3x−8)∘, ∠COD = 50∘ and ∠BOD = (x+10)∘. The value of x is
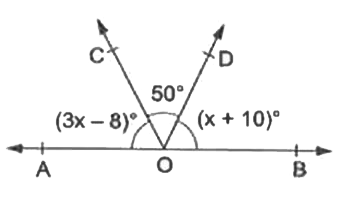
32
42
36
52
The correct Answer is:a
Step by step video, text & image solution for In the given figure, AOB is a straight line, /_AOC = (3x-8)^(@), /_COD = 50^(@) and /_BOD = (x +10)^(@). The value of x is by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - TEST PAPER8videos
CONSTRUCTIONS
- BOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - TEST PAPER (M.C.Q)7videos
CONSTRUCTIONS
- BOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - EXERCISE B10videos
CONSTRUCTIONS
- BOOK - RS AGGARWALCHAPTER - CONGRUENCEEXERCISE - EXERCISE18videos
CONGRUENCE
- BOOK - RS AGGARWALCHAPTER - DECIMALSEXERCISE - TEST PAPER-3(D)5videos
DECIMALS
Similar Questions
Knowledge Check
- Question 1 - Select One
In the given figure, AOB is a straight line, ∠AOC = 68∘ and ∠BOC=X∘ The value of x is
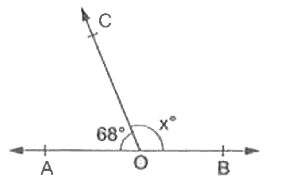A32B22C112D132 - Question 2 - Select One
In the given figure, AOB is a straight line, ∠AOC=56∘ and ∠BOC=x∘. The value of x is
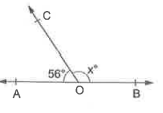A34B44C144D124 - Question 3 - Select One
In the given firgue, AOB is a straight line if ∠AOC=(3x−10)∘∠COD=50∘and∠BOd=(x+20)∘ find ∠AOC
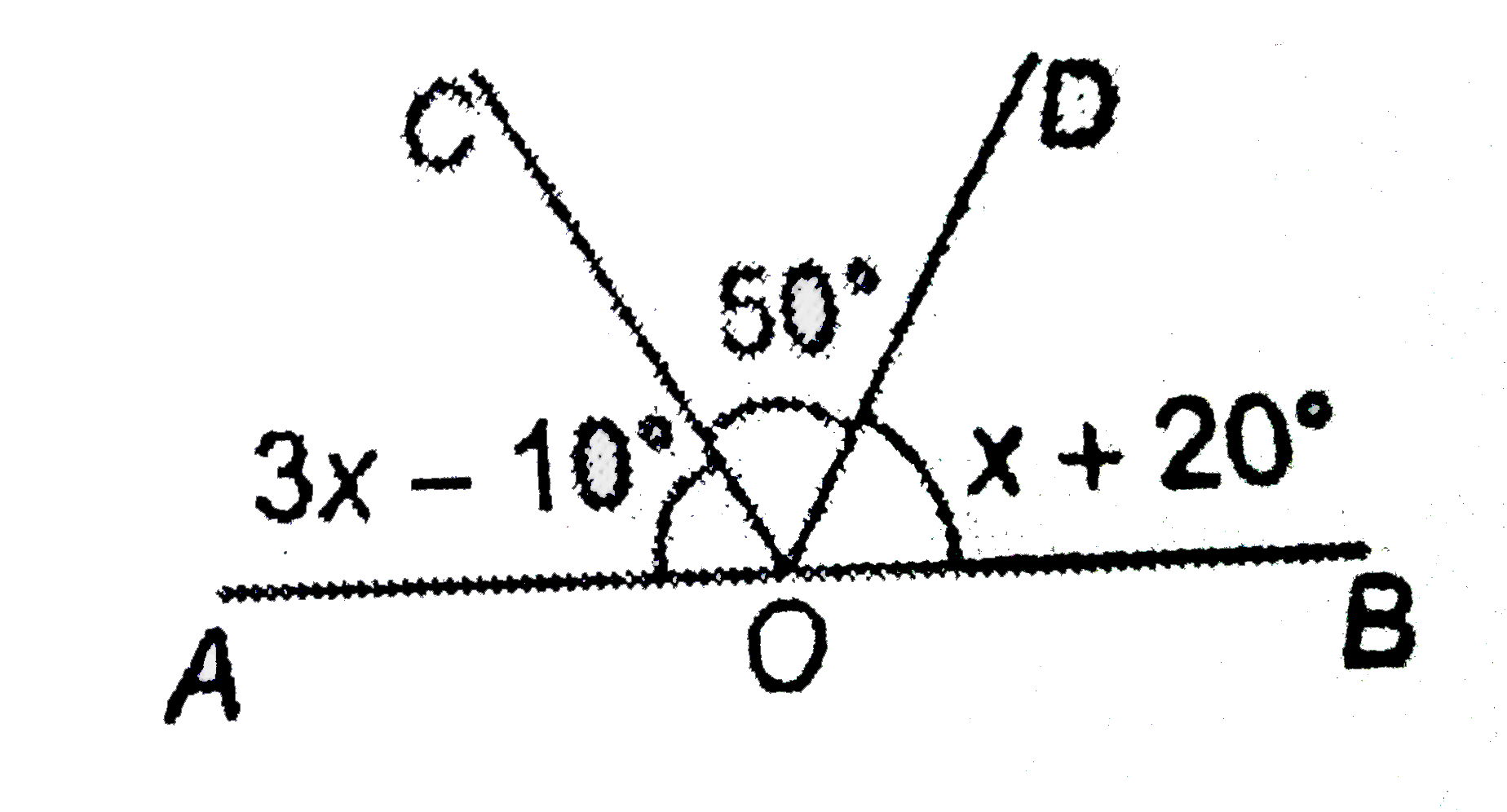A80∘B50∘C70∘D100∘
In the given figure, AOB is a straight line. If ∠AOC=(3x−10)∘,∠COD=50∘ and ∠BOD=(x+20)∘ then ∠AOC=?
View SolutionIn the given figure,XOY is a straight line,find the value of x
View SolutionIn a figure , AB is a straight line , Find (x+y):
View SolutionIn the given figure, AOB is a straight line and OC is a ray such that ∠AOC=(3x+20)∘ and ∠BOC=(2x−10)∘. Find the value of x and hence find (i) ∠AOC
(ii)∠BOC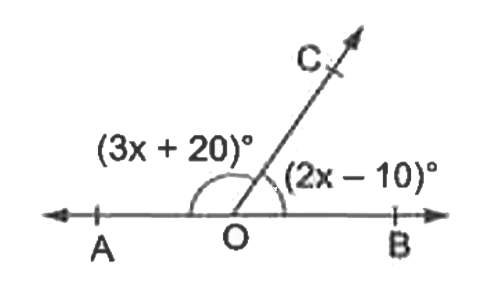
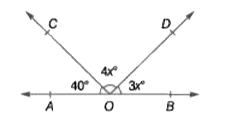
View SolutionIn the given figure, AOB is straight line If ∠AOC=40∘,∠COD=4x∘and∠BOD=3x∘, then ∠COD is equal to
View Solution