Consider a 20W bulb emitting light of wavelength 5000Å and shinning on a metal surface kept at a distance 2m. Assume that the metal surface has work function of 2eV and that each atom on the metal surface can be treated as a circular disk of radius 1.5Å.
(i) Estimate no. of photons emitted by the bulb per second. [Assume no other losses] (ii) Will there be photoelectric emission? (iii) How much time would be required by the atomic disk to receive energy equal to work function 2eV? (iv) How many photons would atomic disk receive within time duration calculated in (iii) above? (v) Can you explain how photoelectric effect was observed instantaneously? [Hint : Time calculated in part (iii) is from classical consideration and you may further take the target of surface area say 1cm2 and estimate what would happen?]
Given, P=200W,λ=5000Å=5000×10−10m
d=2m,ϕ0=2eV,r=1.5A=1.5×10−10m
(i) Number of photon emitted by bulb per second is n' =phc/λ=pλhc
=20×(5000×10−10)(6.62×10−34)×(3×108)
⇒ =5×1019s−1
(ii) Energy of the incident photon
hcλ=(6.62×10−34)(3×108)5000×10−10×1.6×10−19
=2.48 eV
As this energy is greater than e eV (i.e., work function of metal surface), hence photolectric emission takes place.
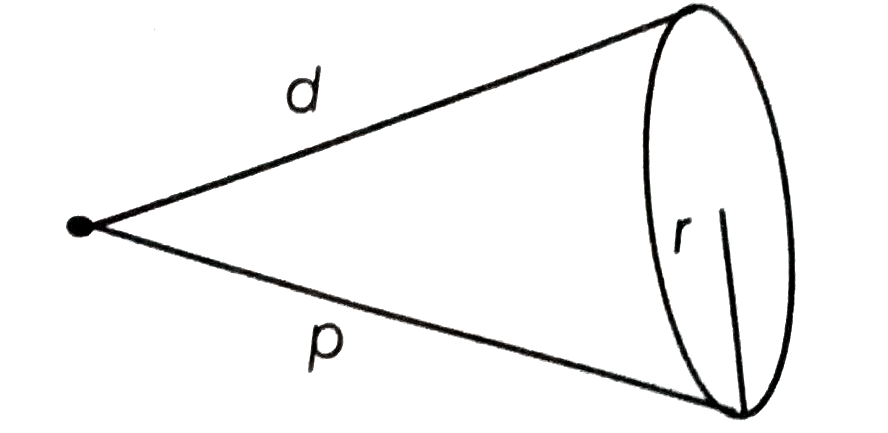
(ii) Let Δt be the time spent in getting the energy ϕ= (work functiojn of metal). Consider the figure,
P4πd2×πr2Δt=ϕ0
⇒ Δt=4ϕ0d2Pr2
=4×(2×1.6×10−19)×2220×(1.5×10−10)2≈28.4s
(iv) Number of photons receivbed by atomic disc in time Δt is
N=n'×πr24πd2×Δt
⇒ =n'r2Δt4d2
=(5×1019)×(1.5×1010)2×28.44×(2)2≈2
(v) As time of emiccion of electrons is 11.04 s.
Hence, the photoelectric emission is not instantaneous in this problem.
In photoelectric emission, there is an collision between incident photon and free electron of the metal surface, which lasts for ever very short interval of time (≈10−9S), hence we say photoelectric emission is instantaneous.
Topper's Solved these Questions
Similar Questions
Light of wavelength 2000 Å falls on a metallic surface whose work function is 4.21 eV. Calculate the stopping potential
View SolutionLight of wavelength 3000 Å falls on a metal surface having work function 2.3 eV. Calculate the maximum velocity of ejected electrons.
(Planck's constant , h=6.63×10−34 J.s. , velocity of light c=3×108m/s , mass of an electron = 9.1×10−31 kg )
View Solution
Knowledge Check
Light of wavelength 4000 Å is incident on a metal surface. The maximum kinetic energy of emitted photoelectron is 2 eV. What is the work function of the metal surface ?
A4 eVB1 eVC2 eVD6 eVLight of wavelength 3000Å is incident on a metal surface whose work function is 1 eV. The maximum velocity of emitted photoelectron will be
A10ms−1B103ms−1C104ms−1D106ms−1Light of wavelength 3000Å is incident on a metal surface whose work function is 1 eV. The maximum velocity of emitted photoelectron will be
A10ms−1B103ms−1C104ms−1D106ms−1
A bulb emits light of 4500Å. The bulb is rated as 150 watt and 9% of the energy is emited as light . How many photons are emitted by the bulb per second ?
View SolutionCalculate no. of photons emitted by 60W bulb in 10 hours that produces light of wavelength 6.626Å
View SolutionLight of wavelenght 5000Å fall on a metal surface of work function 1.9eV Find
a. The energy of photon
b. The kinetic energy of photoelectrons
View SolutionWhen light of wavelength lesser than 6000 Å is incident on a metal, electrons are emitted. The approximate work - function of the metal is :
View SolutionTwo photons, each of energy 2.5 eV are simultaneously incident on the metal surface. If the work function of the metal is 4.5 eV, then from the surface of metal
View Solution