Question

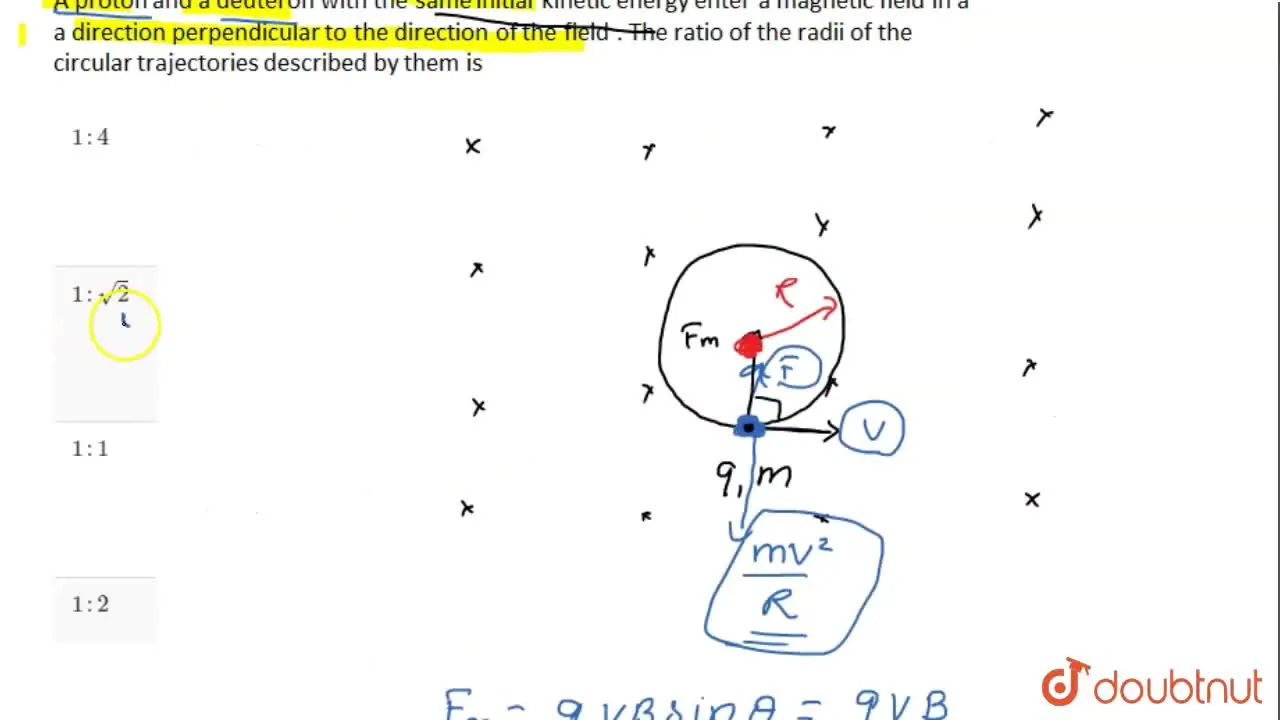
A proton and a deuteron with the same initial kinetic energy enter a magnetic field in a a direction perpendicular to the direction of the field . The ratio of the radii of the circular trajectories described by them is

Options

A. `1:4`
B. `1:sqrt(2)`
C. `1:1`
D. `1:2`

Answer

B

Explanation

To solve the problem of finding the ratio of the radii of the circular trajectories described by a proton and a deuteron entering a magnetic field with the same initial kinetic energy, we can follow these steps:

Step 1: Understand the Motion of Charged Particles in a Magnetic Field
When a charged particle moves in a magnetic field perpendicular to its velocity, it experiences a magnetic force that causes it to move in a circular path. The radius of this circular path can be derived from the balance of the magnetic force and the centripetal force acting on the particle.

Step 2: Write the Expression for the Radius of the Circular Path
The radius \( r \) of the circular path for a charged particle can be expressed as:
\( r = \frac{mv}{qB} \)
where:
- \( m \) is the mass of the particle,
- \( v \) is the velocity of the particle,
- \( q \) is the charge of the particle,
- \( B \) is the magnetic field strength.

Step 3: Relate Velocity to Kinetic Energy
The kinetic energy \( KE \) of a particle is given by:
\( KE = \frac{1}{2} mv^2 \)
From this, we can express \( v \) in terms of kinetic energy:
\( v = \sqrt{\frac{2 \cdot KE}{m}} \)

Step 4: Substitute Velocity in the Radius Formula
Substituting \( v \) into the radius formula gives:
\( r = \frac{m \sqrt{\frac{2 \cdot KE}{m}}}{qB} = \frac{\sqrt{2m \cdot KE}}{qB} \)

Step 5: Calculate the Radius for Proton and Deuteron
1. For Proton:
- Charge \( q_p = 1 \) (in units of elementary charge),
- Mass \( m_p = 1 \) (in atomic mass units).
\( r_p = \frac{\sqrt{2 \cdot 1 \cdot KE}}{1 \cdot B} = \frac{\sqrt{2 \cdot KE}}{B} \)

2. For Deuteron:
- Charge \( q_d = 1 \) (in units of elementary charge),
- Mass \( m_d = 2 \) (in atomic mass units).
\( r_d = \frac{\sqrt{2 \cdot 2 \cdot KE}}{1 \cdot B} = \frac{\sqrt{4 \cdot KE}}{B} = \frac{2 \sqrt{KE}}{B} \)

Step 6: Find the Ratio of the Radii
Now, we can find the ratio of the radii \( \frac{r_p}{r_d} \):
\( \frac{r_p}{r_d} = \frac{\frac{\sqrt{2 \cdot KE}}{B}}{\frac{2 \sqrt{KE}}{B}} = \frac{\sqrt{2 \cdot KE}}{2 \sqrt{KE}} = \frac{\sqrt{2}}{2} = \frac{1}{\sqrt{2}} \)

Step 7: Final Result
Thus, the ratio of the radii of the circular trajectories described by the proton and the deuteron is:
\( \frac{r_p}{r_d} = \frac{1}{\sqrt{2}} \quad \text{or} \quad 1 : \sqrt{2} \)