The ionization energy of hydrogen atom is 13.6 eV. Hydrogen atoms in the ground state are excited by electromagnetic radiation of energy 12.1 eV. How many spectral lines will be emitted by the hydrogen atoms
1
2
3
4
The correct Answer is:C
Step by step video, text & image solution for The ionization energy of hydrogen atom is 13.6 eV. Hydrogen atoms in the ground state are excited by electromagnetic radiation of energy 12.1 eV. How many spectral lines will be emitted by the hydrogen atoms by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Ionization potential of hydrogen atom is 13.6V. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1eV. The spectral lines emitted by hydrogen atoms according to Bohr's theory will be
View SolutionIonization potential of hydrogen atom is 13.6 eV. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1 eV. According to Bohr's theory, the spectral lines emitted by hydrogen will be
View SolutionIonization potential of hydrogen atom is 13.6eV. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1 eV. According to Bohr's theory, the spectral lines emitted by hydrogen will be:-
View SolutionIonisation potential of hydrogen atom is 13.6eV. Hydrogen atom in ground state is excited by monochromatic light of energy 12.1eV. The spectral lines emitted by hydrogen according to Bohr's theory will be
View SolutionHydrogen atoms in the ground state are excited by monochromatic radiation photons of energy 12.1 eV. To which orbit the electron will be lifted, if an hydrogen atom absorbs the photon ?
View SolutionThe ionization potential of H-atom is 13.6 V. The H-atoms in ground state are excited by mono chromatic radiations of photon energy 12.09 ev. Then the number of spectral lines emitted by the excited atoms, will be
View SolutionExcitions energy of hydrogen atom is 13.6eV mathc the following
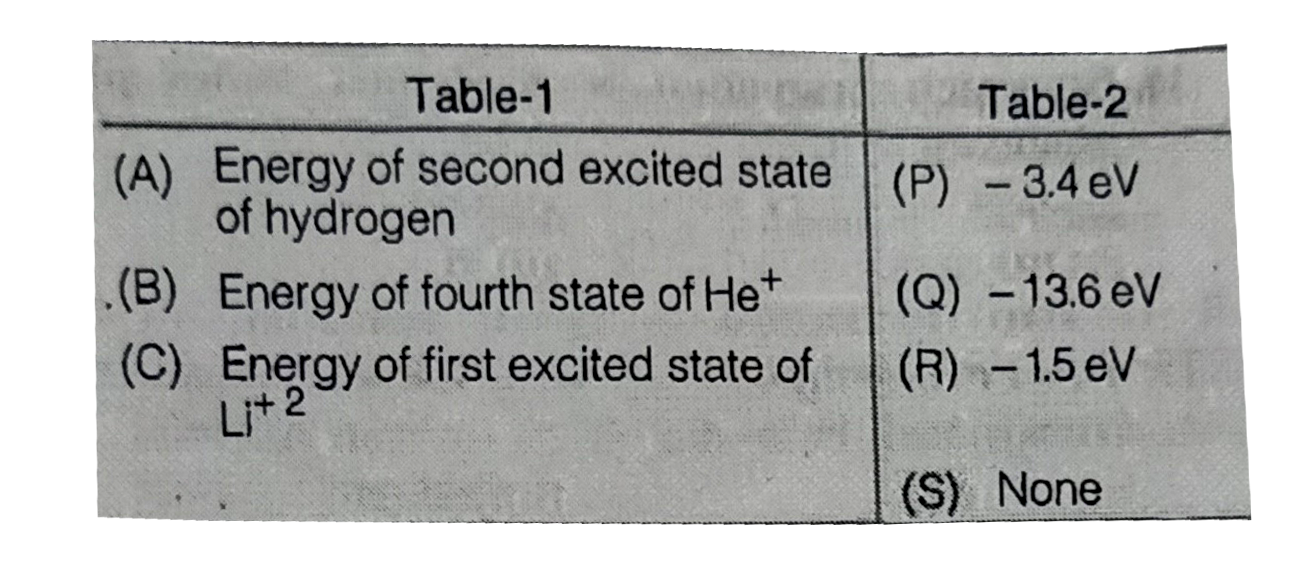 View Solution
View SolutionExcitation energy of hydrogen atom is 13.6eV. Match the following .
 View Solution
View SolutionIonisation potential of hydrogen atom is 13.6eV. Hydrogen atom in ground state is excited by monochromatic light of energy 12.1eV. The spectral lines emitted by hydrogen according to Bohr's theory will be
View SolutionThe ionization potential of H-atom is 13.6 V. The H-atoms in ground state are excited by mono chromatic radiations of photon energy 12.09 ev. Then the number of spectral lines emitted by the excited atoms, will be
View Solution


