Two loops P and Q are made from a uniform wire. The radii of P and Q are R1 and R2, respectively, and their moments of inertia about their axis of rotation are I1 and I2, respectively. If I1I2=4, then R2R1 is
423
413
4−23
4−13
The correct Answer is:B
To solve the problem, we need to find the ratio of the radii R2R1 given the ratio of the moments of inertia I1I2=4.
1. Understanding the Moment of Inertia for a Loop:
The moment of inertia I of a loop (ring) about its axis of rotation is given by the formula:
I=MR2
where M is the mass of the loop and R is its radius.
2. Finding the Mass of Each Loop:
Since both loops are made from a uniform wire, we can express their masses in terms of their radii. The mass M of a loop can be expressed as:
M=μ⋅L
where μ is the mass per unit length and L is the length of the wire used to form the loop. The length of the wire for each loop is:
L=2πR
Thus, the mass of loop P (with radius R1) is:
M1=μ⋅(2πR1)=2πμR1
and for loop Q (with radius R2):
M2=μ⋅(2πR2)=2πμR2
3. Calculating the Moments of Inertia:
Now substituting the masses into the moment of inertia formulas:
I1=M1R21=(2πμR1)R21=2πμR31
I2=M2R22=(2πμR2)R22=2πμR32
4. Finding the Ratio of Moments of Inertia:
Now we can find the ratio of the moments of inertia:
I1I2=2πμR312πμR32=R31R32
Given that I1I2=4, we have:
R31R32=4
5. Finding the Ratio of Radii:
Taking the cube root on both sides gives:
R1R2=41/3
Therefore, we can express R2R1 as:
R2R1=141/3=4−1/3
Final Answer:
R2R1=4−1/3
The moment of inertia I of a loop (ring) about its axis of rotation is given by the formula:
I=MR2
where M is the mass of the loop and R is its radius.
Since both loops are made from a uniform wire, we can express their masses in terms of their radii. The mass M of a loop can be expressed as:
M=μ⋅L
where μ is the mass per unit length and L is the length of the wire used to form the loop. The length of the wire for each loop is:
L=2πR
Thus, the mass of loop P (with radius R1) is:
M1=μ⋅(2πR1)=2πμR1
and for loop Q (with radius R2):
M2=μ⋅(2πR2)=2πμR2
Now substituting the masses into the moment of inertia formulas:
I1=M1R21=(2πμR1)R21=2πμR31
I2=M2R22=(2πμR2)R22=2πμR32
Now we can find the ratio of the moments of inertia:
I1I2=2πμR312πμR32=R31R32
Given that I1I2=4, we have:
R31R32=4
Taking the cube root on both sides gives:
R1R2=41/3
Therefore, we can express R2R1 as:
R2R1=141/3=4−1/3
R2R1=4−1/3
Topper's Solved these Questions
Similar Questions
Two loops P and Q are made from a uniform wire. The redii of P and Q are r1andr2 respectively, and their moments of inertia are I1andI2 respectively, IfI2=4I1,thenr2r1equals-
View SolutionTwo circular loops A and B of radii R and 2R respectively are made of the similar wire. Their moments of inertia about the axis passing through the centre of perpendicular to their plane are IA and IB respectively. The ratioIAIB is :
View Solution
Knowledge Check
Two loops P and Q are made from a uniform wire. The redii of P and Q are r1andr2 respectively, and their moments of inertia are I1andI2 respectively, IfI2=4I1,thenr2r1equals-
A42/3B41/3C4−2/3D4−1/3Two loops P and Q are made from a uniform wire. The radii of P and Q are R1andR2 respectively and their moments of inertia are IPandIQ respectively.
If IPIQ=8 then R1R2 isA5B4C3D2Two loops P and Q are made from a uniform wire. The redii of P and Q are r1andr2 respectively, and their moments of inertia are I1andI2 respectively, IfI2=4I1,thenr2r1equals-
A423B413C4−23D4−13
Two conducting spheres of radii r1 and r2 having charges Q1 and Q2 respectively are connected to each other. There is
View SolutionThe insulated spheres of radii R1 and R2 having charges Q1 and Q2 respectively are connected to each other. There is
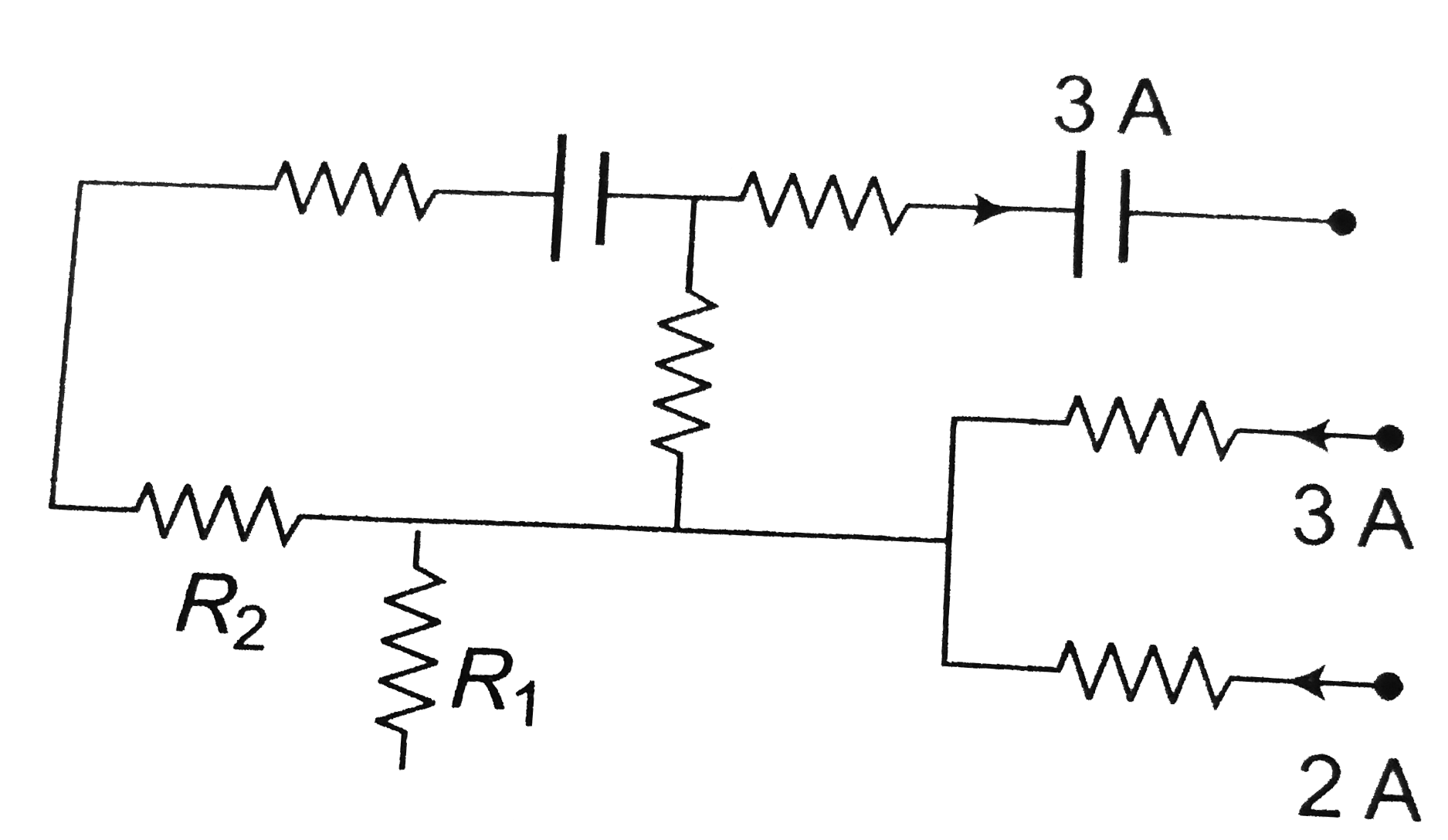
View SolutionIn the given circuit if I1 and I2 be the current in resistance R1 and R2 respectively then:
View SolutionThe insulated spheres of radii R1 and R2 having charges Q1 and Q2 respectively are connected to each other. There is
View Solution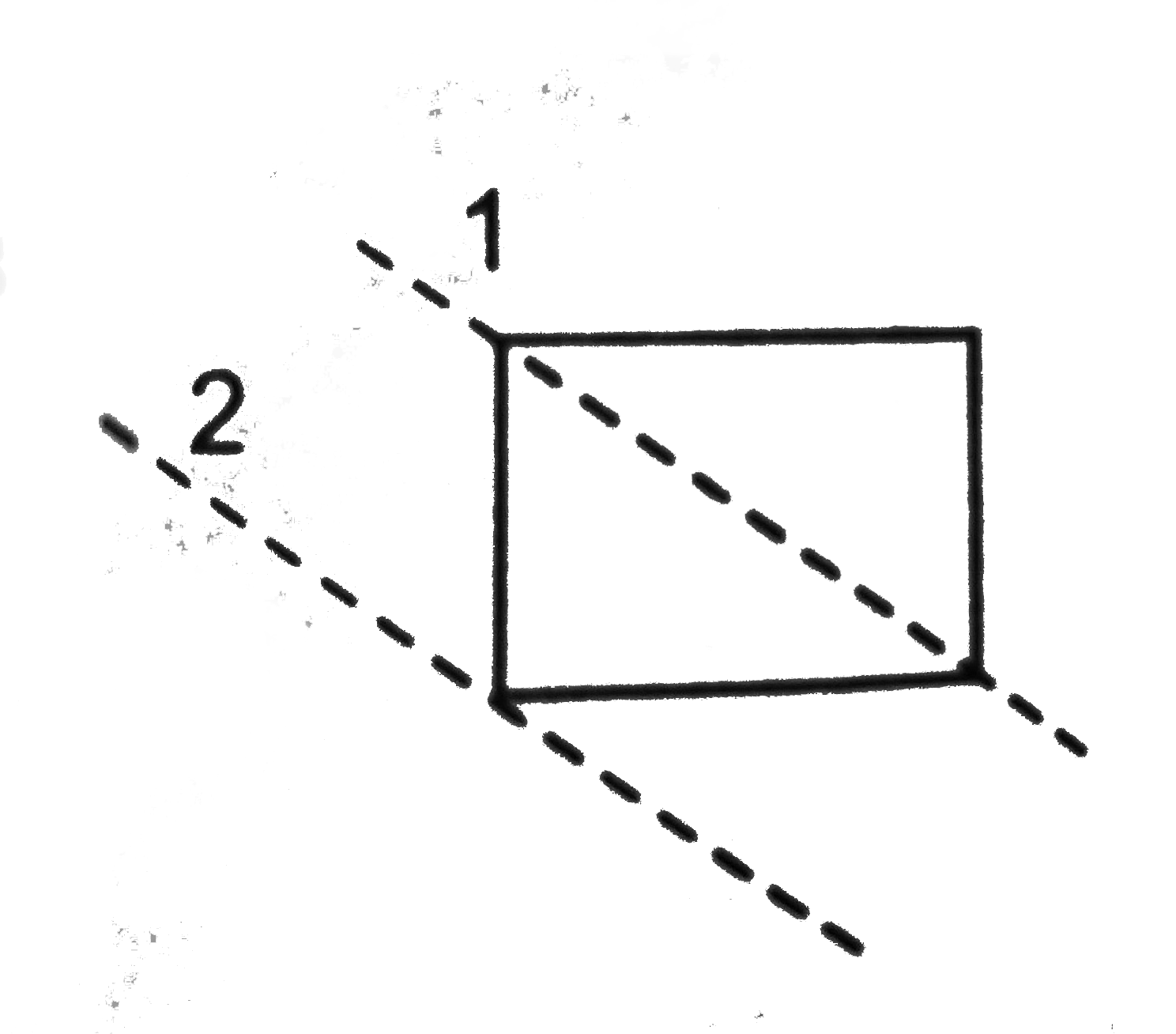
Let I1 and I2 be the moment of inertia of a uniform square plate about axes shown in the figure. Then the ratio I1:I2 is
View Solution
