Acetic acid is partially dimerised to in the vapour phase. At a total pressure of 0.200 atm, acetic acid is dimerized at 298 K. The value of equilibrium constant of dimerization under these conditions is
The correct Answer is:D
To solve the problem of finding the equilibrium constant of dimerization of acetic acid (CH₃COOH) at a total pressure of 0.200 atm, where it is 92.0% dimerized at 298 K, we can follow these steps:
Step 1: Write the Dimerization Reaction
The dimerization of acetic acid can be represented as:
\( 2 \text{CH}_3\text{COOH} \rightleftharpoons \text{(CH}_3\text{COOH)}_2 \)
Step 2: Define Initial and Equilibrium Concentrations
Let the initial amount of acetic acid be 1 mole. At equilibrium, if α is the degree of dimerization, then:
- Moles of acetic acid remaining = \( 1 - \alpha \)
- Moles of dimer formed = \( \frac{\alpha}{2} \)
Given that the dimerization is 92% complete, we have:
\( \alpha = 0.92 \)
Step 3: Calculate Moles at Equilibrium
At equilibrium:
- Moles of acetic acid = \( 1 - 0.92 = 0.08 \)
- Moles of dimer = \( \frac{0.92}{2} = 0.46 \)
Step 4: Calculate Total Moles at Equilibrium
Total moles at equilibrium:
\( \text{Total moles} = 0.08 + 0.46 = 0.54 \)
Step 5: Calculate Partial Pressures
Using the total pressure \( P = 0.200 \, \text{atm} \):
- Partial pressure of dimer \( P_d \):
\( P_d = \frac{0.46}{0.54} \times 0.200 \)
- Partial pressure of acetic acid \( P_a \):
\( P_a = \frac{0.08}{0.54} \times 0.200 \)
Step 6: Substitute Values into the Equilibrium Constant Expression
The equilibrium constant \( K \) for the dimerization reaction is given by:
\( K = \frac{P_d}{(P_a)^2} \)
Step 7: Calculate the Values
1. Calculate \( P_d \):
\( P_d = \frac{0.46}{0.54} \times 0.200 = 0.17037 \, \text{atm} \)
2. Calculate \( P_a \):
\( P_a = \frac{0.08}{0.54} \times 0.200 = 0.02963 \, \text{atm} \)
3. Substitute into the equilibrium constant expression:
\( K = \frac{0.17037}{(0.02963)^2} \)
Step 8: Final Calculation
Calculate \( K \):
\( K = \frac{0.17037}{0.000876} \approx 194.062 \)
Conclusion
The value of the equilibrium constant \( K \) for the dimerization of acetic acid under the given conditions is approximately:
\( K \approx 194.06 \, \text{atm}^{-1} \)
The dimerization of acetic acid can be represented as:
\( 2 \text{CH}_3\text{COOH} \rightleftharpoons \text{(CH}_3\text{COOH)}_2 \)
Let the initial amount of acetic acid be 1 mole. At equilibrium, if α is the degree of dimerization, then:
- Moles of acetic acid remaining = \( 1 - \alpha \)
- Moles of dimer formed = \( \frac{\alpha}{2} \)
\( \alpha = 0.92 \)
At equilibrium:
- Moles of acetic acid = \( 1 - 0.92 = 0.08 \)
- Moles of dimer = \( \frac{0.92}{2} = 0.46 \)
Total moles at equilibrium:
\( \text{Total moles} = 0.08 + 0.46 = 0.54 \)
Using the total pressure \( P = 0.200 \, \text{atm} \):
- Partial pressure of dimer \( P_d \):
\( P_d = \frac{0.46}{0.54} \times 0.200 \)
- Partial pressure of acetic acid \( P_a \):
\( P_a = \frac{0.08}{0.54} \times 0.200 \)
The equilibrium constant \( K \) for the dimerization reaction is given by:
\( K = \frac{P_d}{(P_a)^2} \)
1. Calculate \( P_d \):
\( P_d = \frac{0.46}{0.54} \times 0.200 = 0.17037 \, \text{atm} \)
\( P_a = \frac{0.08}{0.54} \times 0.200 = 0.02963 \, \text{atm} \)
\( K = \frac{0.17037}{(0.02963)^2} \)
Calculate \( K \):
\( K = \frac{0.17037}{0.000876} \approx 194.062 \)
The value of the equilibrium constant \( K \) for the dimerization of acetic acid under the given conditions is approximately:
\( K \approx 194.06 \, \text{atm}^{-1} \)
Topper's Solved these Questions
Similar Questions
Acetoc acid CH3COOH can form a dimer (CH3COOH)2 in the gas phase. The dimer is held togther by two H−bonds with a total strength of 60.0kJper mole of dimer
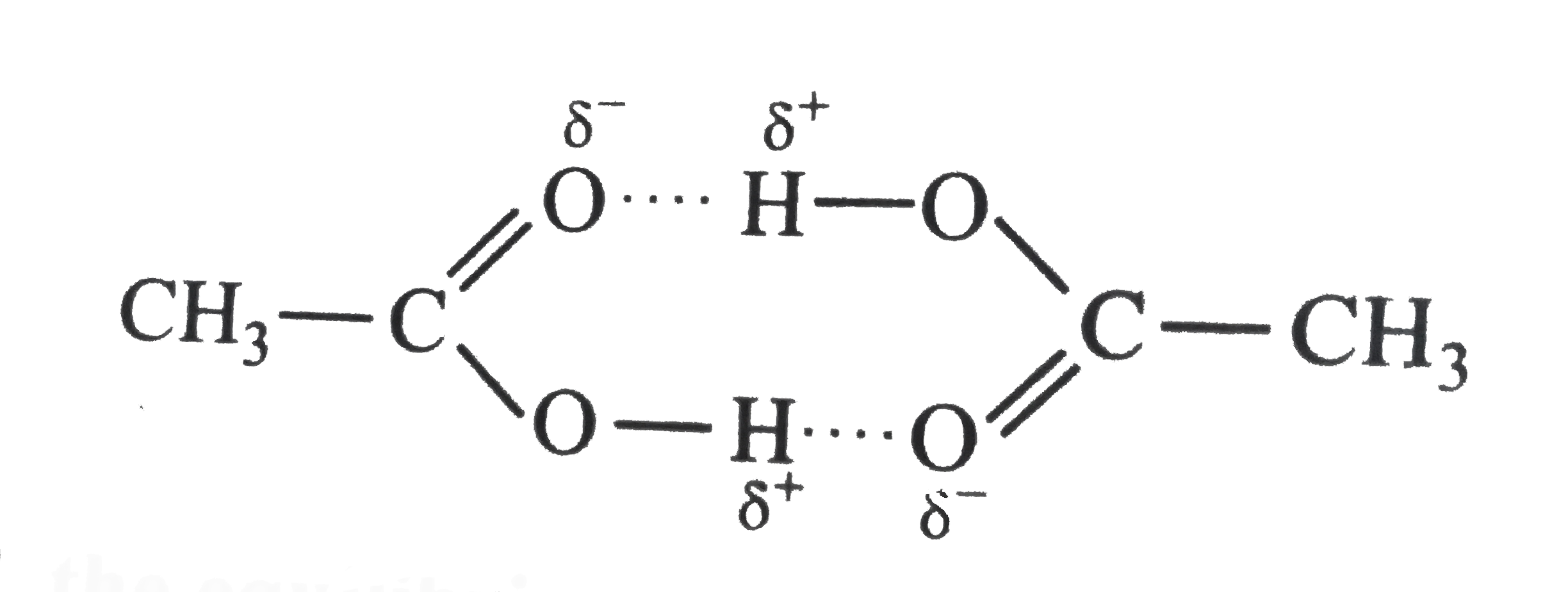
If at 25∘C, the equilibrium constant for the dimerisation is 1.3×103, calculate ΔSΘ for the reaction
2CH3COOH(g)⇔(CH3COOH)2(g)
View SolutionWhy is CH2=CH−COOH a stronger acid than CH3CH2COOH?
View Solution
Knowledge Check
Which gas convert methanol (CH3OH) to acetic acid (CH3COOH) at high temperature and pressure with catalysts?
AN2BCO2CCODO2Freezing point of benzene is 278.4K and heat of fusion of benzene is 10.042 KJ/mol. Acetic acid exists partly as dimer in benzene solution. The freezing point of 0.02mol fraction of acetic acid in benzene is 277.4K.
The equilibrium constant for dimerisation of acetic acid in benzene isA2.4B3.39C2.42D2.56(CH3)3CCH2COOH is more acidic than (CH3)3SiCH2COOH because
ASize of Si is more than that of carbonBElectronegativity of carbon is less than that of siliconCSilicon is more electropositive than carbon due to which (CH3)3SiCH2COO− becomes less stableDNone of the above
Find pH of 0.1 M CH3COOH(aq) solution K (CH3COOH)=10−5
View SolutionAcetic acid forms dimer in vapour phase. The dimer is held together by two hydrogen bonds with a total strength of 66.5 kJ per mole of dimer. If at 25∘C, the equilibrium constant for the dimerisation is 1.3×103. ΔS∘ for the reaction 2CH3COOH⇔(CH3COOH)2 will be (log1.3=0.1139)
View SolutionAcetic acid, (CH3COOH), has a pKa of 4.8. Ethanol, (CH3CH2OH) has a pKa of 16.0 . What are the major species present, when acetic and ethanol are added to water and th e pH is odjusted to 7.0?
View SolutionSame mass of glucose (C6H12O6) and acetic acid (CH3COOH) contain:
View Solution0.1 M CH3COOH solution is 1.0% ionized. In another diluted solution acetic acid is 10% ionised. In other solution concentration of acetic acid is
View Solution