Three identical blocks A, B and C are placed on horizontal frictionless surface. The blocks B and C are at rest. But A is approaching towards B with a speed of 10ms−1
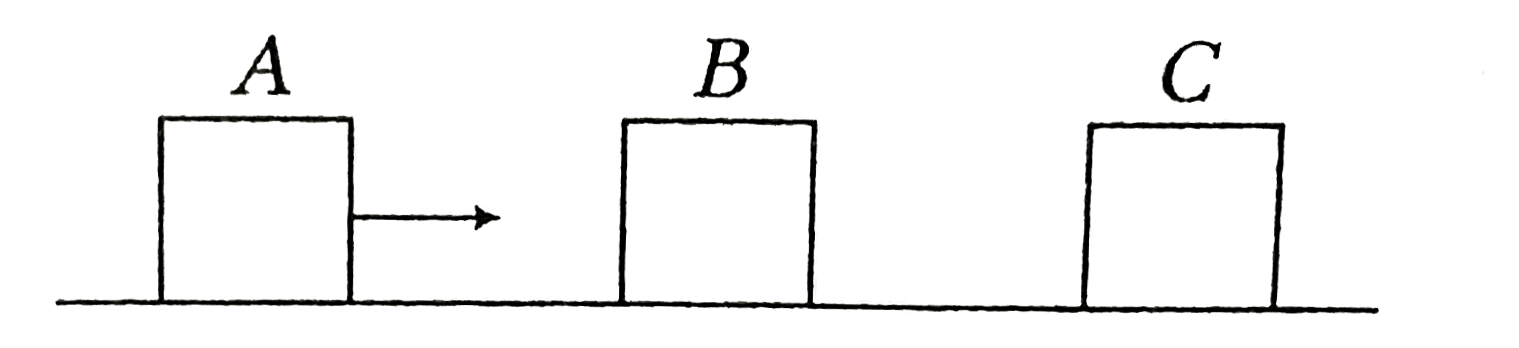
The coefficient of restitution for all collision is 0.5. The speed of the block C just after collision is
5.6ms−1
6ms−1
8ms−1
10ms−1
The correct Answer is:A
Step by step video, text & image solution for Three identical blocks A, B and C are placed on horizontal frictionless surface. The blocks B and C are at rest. But A is approaching towards B with a speed of 10 ms^(-1) The coefficient of restitution for all collision is 0.5. The speed of the block C just after collision is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
A block of mas m moving at a velocity υ collides head on with another block of mass 2m at rest. If the coefficient of restitution is 1/2, find the velocities of te blocks after the collision.
View SolutionBlock of mass m1 moving on a smooth horizontal surface,collides with a stationary block of mass m2 .The coefficient of restitution for the collision is e.The ratio m1m2 for which m1 is at rest after collision is
View Solution
Knowledge Check
- Question 1 - Select One
Three identical spherical balls A, B and C are placed on a table as shown in the figure along a straight line. B and Care at rest initially.

The ball A hits B head on with a speed of 10ms−1. Then after all collisions (assumed to be elastic) A and B are brought to rest and C takes off with a velocity ofA5ms−1B10ms−1C2.5ms−1D7.5ms−1 - Question 2 - Select One
A bullte weighing 5g and moving with a velocity 600m/s strikes a 5kg block of ice resting on a frictionless surface. The speed of the block after the collision is
A6cm/sB60cm/sC6m/sD0.6cm/s - Question 3 - Select One
A sphere A impinges directly on an identical sphere B at rest. If coefficient of restitution is e , the ratio of velocities of A and B after collision is
A1−e1+eB1+e1−eCe1+eD2e1+e
Two blocks A and B of masses m and 2m are placed on a smooth horizontal surface. Block B is given a speed of 3m/s. Find
(i) The maximum speed of A
(ii) The minimum speed of B.
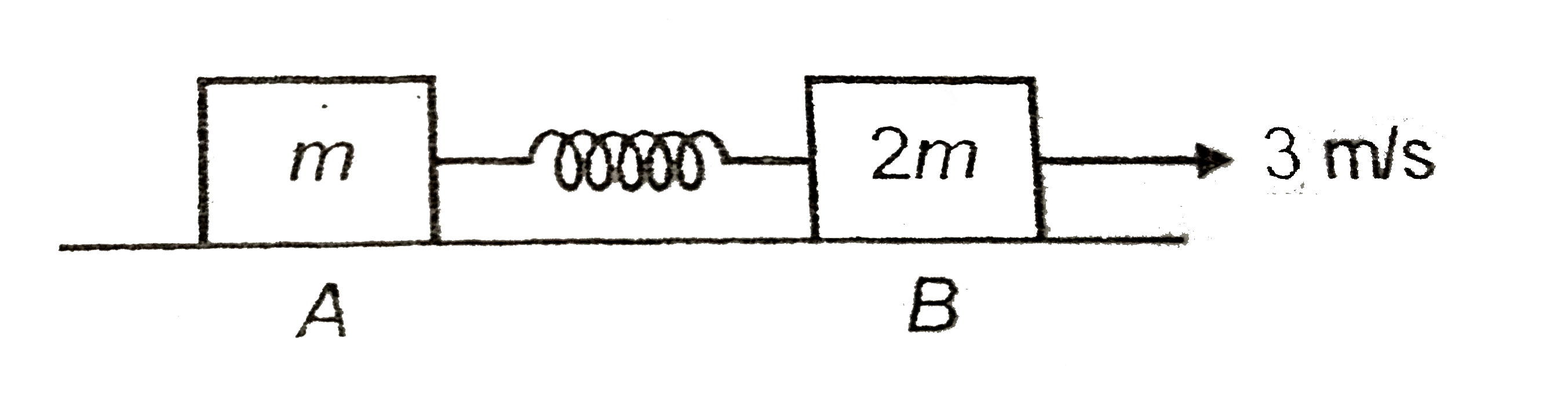 View Solution
View SolutionFour identical balls A,B,C and D are placed in a line on frictionless horizontal surface. A and D are moved with the same speed u towards the middle as shown. Assuming elastic collisions, find the final velocities.
 View Solution
View SolutionThree blocks of identical masses are placed on a frictionless table as shown . The centre block is at rest , whereas the other two blocks are moving directly towards the stationary block with identical speed v . The centre block is initially closer to the left block than the right one . All collisions are elastic . After long time , which of the following is true .
 View Solution
View SolutionThree blocks A, B and C each of mass m are placed on a surface as shown in the figure. Blocks B and C are initialyy at rest. Block A is moving to the right with speed v. It collides with block B and stricks to it. The A-B combination collides elastically with block C. Which of the following statement is (are) true about the velocity, of block B and C.
 View Solution
View SolutionA buttle weighting 10 g and moving with a velocity 800ms−1 strikes a 10 kg block resting on a frictionless surface. The speed of the block after the perfectly inelastic collision is approximately
View Solution

