The reaction X+Y→Z is first order with respect to X and second order with respect to Y, initial rate of formation of Z=Rmol dm3sec−1 when [X] and [Y] are 0.40 mol dm−3 and 0.30 mol dm−3 respectively . If [X] is halved and [Y] is doubled , the value of the initial rate would become
4 R
R4
R
2R
The correct Answer is:D
To solve the problem, we need to determine how the initial rate of formation of Z changes when the concentrations of X and Y are altered. The reaction is given as:
X+Y→Z
The rate law for this reaction is expressed as:
Rate=k(X)m(Y)n
Where:
- m=1 (first order with respect to X)
- n=2 (second order with respect to Y)
Thus, the rate law becomes:
Rate=k(X)1(Y)2
Step 1: Calculate the initial rate with given concentrations
Given:
- (X)=0.40mol dm−3
- (Y)=0.30mol dm−3
- Initial rate of formation of Z = Rmol dm−3sec−1
Substituting the values into the rate equation:
R=k(0.40)1(0.30)2
Calculating (0.30)2:
(0.30)2=0.09
Thus, the equation becomes:
R=k(0.40)(0.09)
Step 2: Modify the concentrations of X and Y
Now, we need to find the new rate when:
- (X) is halved: (X)=0.402=0.20mol dm−3
- (Y) is doubled: (Y)=2×0.30=0.60mol dm−3
Step 3: Calculate the new rate with modified concentrations
Substituting the new concentrations into the rate equation:
Ratenew=k(X)(Y)2
Substituting the new values:
Ratenew=k(0.20)1(0.60)2
Calculating (0.60)2:
(0.60)2=0.36
Thus, the new rate becomes:
Ratenew=k(0.20)(0.36)
Step 4: Relate the new rate to the initial rate
Now, we can express the new rate in terms of the initial rate:
From the initial rate calculation, we had:
R=k(0.40)(0.09)
Now, substituting back into the new rate:
Ratenew=k(0.20)(0.36)
Now, we can express Ratenew in terms of R:
Ratenew=k(0.20)(0.36)=k(0.20)(0.36)=k(0.20)(0.36)
Step 5: Calculate the ratio of new rate to initial rate
Now we can find the ratio of the new rate to the initial rate:
RatenewR=k(0.20)(0.36)k(0.40)(0.09)=(0.20)(0.36)(0.40)(0.09)
Calculating the right side:
=0.20×0.360.40×0.09=0.0720.036=2
Thus, we find:
Ratenew=2R
Final Answer
The new initial rate of formation of Z will be 2Rmol dm−3sec−1.
---
- m=1 (first order with respect to X)
- n=2 (second order with respect to Y)
- (X)=0.40mol dm−3
- (Y)=0.30mol dm−3
- Initial rate of formation of Z = Rmol dm−3sec−1
- (X) is halved: (X)=0.402=0.20mol dm−3
- (Y) is doubled: (Y)=2×0.30=0.60mol dm−3
Topper's Solved these Questions
Similar Questions
Knowledge Check
A reaction that is of the first order with respect to reactant A has a rate constant 6min−1. If we start with [A]=0.05mol1−1, when would [A] reach the value 0.05mol1−1
A0.384minB0.15minC3minD3.84minA reaction that is of the first order with respect to reactant A has a rate constant 0.6min−1. If we state with [A] = 0.5 mol.l−1, when would.A reach the value 0.05mol.l−1 ?
A0.384 minB0.15 minC3 minD3.84 minTotal order of reaction X+Y→XY is 3. the order of reaction with respect to X is 2. State the different rate equation for the reaction .
A−d[x]dt=k[X]3[y]0B−d[X]dt=k[x]0[y]3C−d[X]dt=k[x]2[Y]D−d[x]dt=k[x][y]2
Calculate critical temperature and critical pressure of oxygen if the values of the van der Waal's constants a and b are 1.32dm6barmol−2 and 0.0312dm3mol−1 respectively.
View SolutionFor a chemical reaction A→B,the rate of the reaction is 2.0×10−3sec−1, when the initial concentration is 0.05moldm−3. The rate of the same reaction is 1.6×10−2moldm−3sec−1. When the initial concentration is 0.1 mol dm3, find the order of reaction.
View SolutionConsider the kinetic data given in the following table for the reaction A+B+C→ Product.
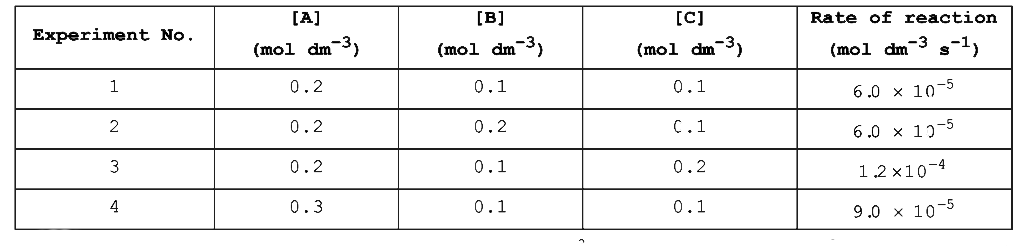
The rate of the reaction for [A]=0.15 mol dm−3,[B]=0.25 mol dm−3and[C]=0.15 mol dm−3, is found to be Y×10−5 mol dm−3s−1. The value of Y is ____.
View SolutionA first order reaction with respect to reactant A , has a rate constant 6 min−1 . If we start with [A]=0.5mol L−1 , when would [A] reach the value 0.05 mol L−1
View SolutionThe rate constant of a zero order reaction is0.2molL−3h−1. If the concentration of the reactant after 30 min is 0.05 mol dm−3, then its initial concentration would be
View Solution