The initial concentration of X and Y were 2 and 4 mole / L respectively . For the following equilibrium X+2Y⇔Z which of the following relationship among equilibrium concentrations of x , y and z is not feasible ?
[X]<[Z]
[X]<[Y]
[X]>[Y]
[Y]>[Z]
The correct Answer is:C
To solve the problem, we need to analyze the equilibrium reaction and the initial concentrations of the reactants. The reaction is:
X+2Y⇌Z
Step 1: Identify Initial Concentrations
- Initial concentration of X = 2 M
- Initial concentration of Y = 4 M
Step 2: Set Up Change in Concentration
Let a be the amount of X that reacts at equilibrium. According to the stoichiometry of the reaction:
- Change in concentration of X = −a
- Change in concentration of Y = −2a (since 2 moles of Y react for every mole of X)
- Change in concentration of Z = +a
Step 3: Write Equilibrium Concentrations
At equilibrium, the concentrations will be:
- Concentration of X = 2−a
- Concentration of Y = 4−2a
- Concentration of Z = a
Step 4: Analyze Each Relationship
Now, we will analyze the given relationships among the equilibrium concentrations of X, Y, and Z.
1. Relationship 1: (X)<(Z)
- 2−a<a
- Rearranging gives 2<2a or a>1
2. Relationship 2: (X)<(Y)
- 2−a<4−2a
- Rearranging gives 2a<2 or a<1
3. Relationship 3: (X)>(Y)
- 2−a>4−2a
- Rearranging gives a>2
4. Relationship 4: (Y)>(Z)
- 4−2a>a
- Rearranging gives 4>3a or a<43
Step 5: Determine Feasibility
Now we need to check which of these relationships can coexist:
- From Relationship 1, we have a>1.
- From Relationship 2, we have a<1 (not feasible with Relationship 1).
- From Relationship 3, we have a>2 (not feasible with Relationship 1).
- From Relationship 4, we have a<43 (not feasible with Relationship 3).
Conclusion:
The relationship that is not feasible is Relationship 3: (X)>(Y) because it requires a>2, which contradicts the limits established by the initial concentrations of X and Y.
---
- Initial concentration of X = 2 M
- Initial concentration of Y = 4 M
Let a be the amount of X that reacts at equilibrium. According to the stoichiometry of the reaction:
- Change in concentration of X = −a
- Change in concentration of Y = −2a (since 2 moles of Y react for every mole of X)
- Change in concentration of Z = +a
At equilibrium, the concentrations will be:
- Concentration of X = 2−a
- Concentration of Y = 4−2a
- Concentration of Z = a
Now, we will analyze the given relationships among the equilibrium concentrations of X, Y, and Z.
- 2−a<a
- Rearranging gives 2<2a or a>1
- 2−a<4−2a
- Rearranging gives 2a<2 or a<1
- 2−a>4−2a
- Rearranging gives a>2
- 4−2a>a
- Rearranging gives 4>3a or a<43
Now we need to check which of these relationships can coexist:
- From Relationship 1, we have a>1.
- From Relationship 2, we have a<1 (not feasible with Relationship 1).
- From Relationship 3, we have a>2 (not feasible with Relationship 1).
- From Relationship 4, we have a<43 (not feasible with Relationship 3).
The relationship that is not feasible is Relationship 3: (X)>(Y) because it requires a>2, which contradicts the limits established by the initial concentrations of X and Y.
Topper's Solved these Questions
Similar Questions
The initial molar concentration of the reactants A and B were 0.1 M and 0.2 M respectively in the following reaction
A+B⇔2C
When equilibrium was attained the concentration of A in the reaction mixture was found to be 0.06M . Calculate the equilibrium constant.
View SolutionThe equilibrium concentration of x, y and z are 4,2 and 2 mol L−1, respectively, at equilibrium of the reaction 2x+y⇔z. The value of Kc is ……………..
View Solution
Knowledge Check
The initial concentration of X and Y were 2 and 4 mole / L respectively . For the following equilibrium X+2Y⇔Z which of the following relationship among equilibrium concentrations of x , y and z is not feasible ?
A[X] = [Z]B[X]<[Y]C[X]>[Y]D[Y]>=<[Z]The equilibrium concentration of x, y and z are 4,2 and 2 mol L−1, respectively, at equilibrium of the reaction 2x+y⇔z. The value of Kc is ……………..
A0.625B2.0625C6.25D0.00625The equilibrium concentration of X, Y and YX2 are 4, 2 and 2 moles respectively for the equilibrium 2X(g)+Y(g)⇔YX2_((g)).The value of KC is -
A0.625B0.0625C6.25D0.00625
The equilibrium concentrations of A, B and C for the reaction A⇔B+C are 46, 2.3 and 2.3 moles/L respectively at 25∘C. If 2 moles per litre of A are removed, calculate the equilibrium concentrations of A, B and C at the same temperature.
View SolutionFor a reversible reaction: X+2Y→2Z, the equilibrium concentrations of X,Y and Z are 0.32,0.40 and 0.35 moles L−1 respectively at 25∘C.
a. If unitially the system contained only X and Y and then reached the state of equilibrium, what were the initial concentrations of X and Y.
b. If at the start only X and Z were present, what were the initial concentrations?
View SolutionFor the reaction:
N2+3H2⇔2NH3
If the initial concentration of N2 and H2 are a and b moles per litre and x moles per litre of ammonia is formed at equilibrium, what is the equilibrium concentration of hydrogen?
View SolutionX, Y and Z react in the 1:1:1 stoichiometric ratio.
The concentration of X, Y and Z we are found to vary with time as shown in the figure below:
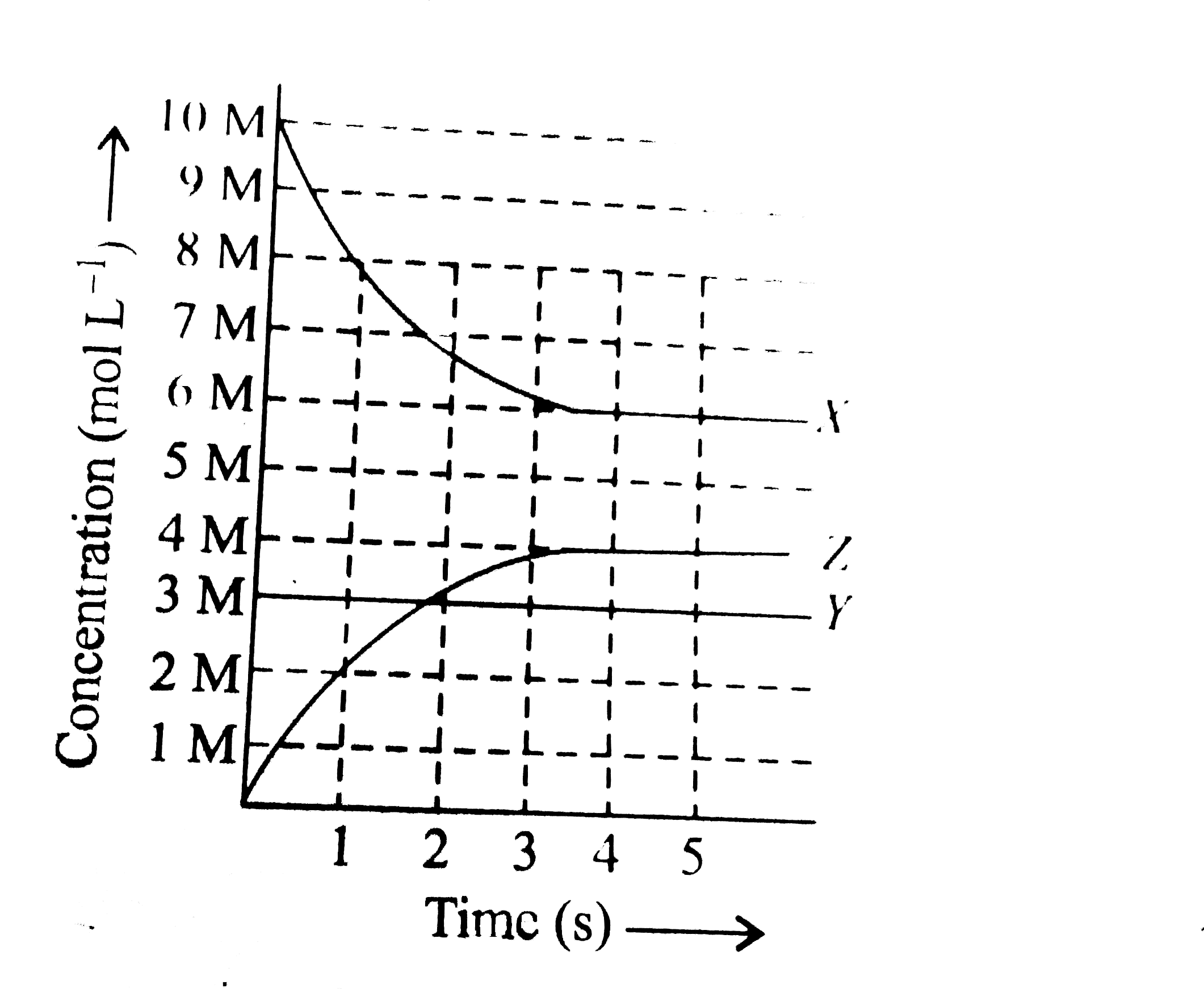
Which of the following equilibrium reaction represents the correct variation of concentration with time?
View SolutionOne mole of X and Y are allowed to react in a 2L container when equilinrium is reached the following reaction occurs 2X+Y⇔Z. If the concentration of Z is 0.2M calculate the equilibrium constant for this reaction
View Solution
