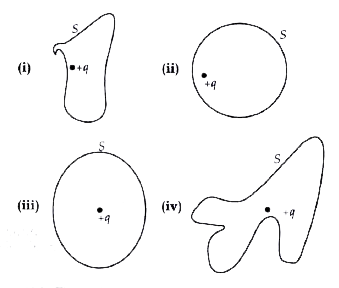What is the electric flux through a cube of side 1 cm which encloses an electric dipole ?
To solve the problem of finding the electric flux through a cube of side 1 cm that encloses an electric dipole, we can follow these steps:
1. Understanding the Problem:
- We have a cube with a side length of 1 cm.
- Inside this cube, there is an electric dipole, which consists of two equal and opposite charges (a positive charge +q and a negative charge -q).
2. Applying Gauss's Law:
- Gauss's Law states that the electric flux (Φ) through a closed surface is equal to the net charge (Q) enclosed by that surface divided by the permittivity of free space (ε₀):
Φ=Qencϵ0
3. Calculating the Net Charge:
- In the case of an electric dipole, the total charge enclosed by the cube is:
Qenc=+q+(−q)=0
- Therefore, the net charge inside the cube is zero.
4. Finding the Electric Flux:
- Substituting the net charge into Gauss's Law:
Φ=0ϵ0=0
- This means that the electric flux through the cube is zero.
5. Conclusion:
- The electric flux through the cube that encloses the electric dipole is:
Φ=0N m2/C
- We have a cube with a side length of 1 cm.
- Inside this cube, there is an electric dipole, which consists of two equal and opposite charges (a positive charge +q and a negative charge -q).
- Gauss's Law states that the electric flux (Φ) through a closed surface is equal to the net charge (Q) enclosed by that surface divided by the permittivity of free space (ε₀):
Φ=Qencϵ0
- In the case of an electric dipole, the total charge enclosed by the cube is:
Qenc=+q+(−q)=0
- Therefore, the net charge inside the cube is zero.
- Substituting the net charge into Gauss's Law:
Φ=0ϵ0=0
- This means that the electric flux through the cube is zero.
- The electric flux through the cube that encloses the electric dipole is:
Φ=0N m2/C
Topper's Solved these Questions
- CBSE EXAMINATION PAPER, DELHI REGION - 2015 (CODE NO. 55/1/1/D)Book:CBSE COMPLEMENTARY MATERIALChapter:CBSE EXAMINATION PAPER, DELHI REGION - 2015 (CODE NO. 55/1/1/D)Exercise:SECTION B
Explore 6 Videos
- CBSE EXAMINATION PAPER, DELHI REGION - 2015 (CODE NO. 55/1/1/D)Book:CBSE COMPLEMENTARY MATERIALChapter:CBSE EXAMINATION PAPER, DELHI REGION - 2015 (CODE NO. 55/1/1/D)Exercise:SECTION C
Explore 15 Videos
- CBSE Examination Paper Delhi–2014Book:CBSE COMPLEMENTARY MATERIALChapter:CBSE Examination Paper Delhi–2014Exercise:SET-III (Questions Uncommon to set-I and II)
Explore 9 Videos
- DUAL NATURE OF MATTER AND RADIATION & ATOMS AND NUCLEIBook:CBSE COMPLEMENTARY MATERIALChapter:DUAL NATURE OF MATTER AND RADIATION & ATOMS AND NUCLEIExercise:Long Answer Question
Explore 22 Videos
Similar Questions
Knowledge Check
The electric flux through the surface
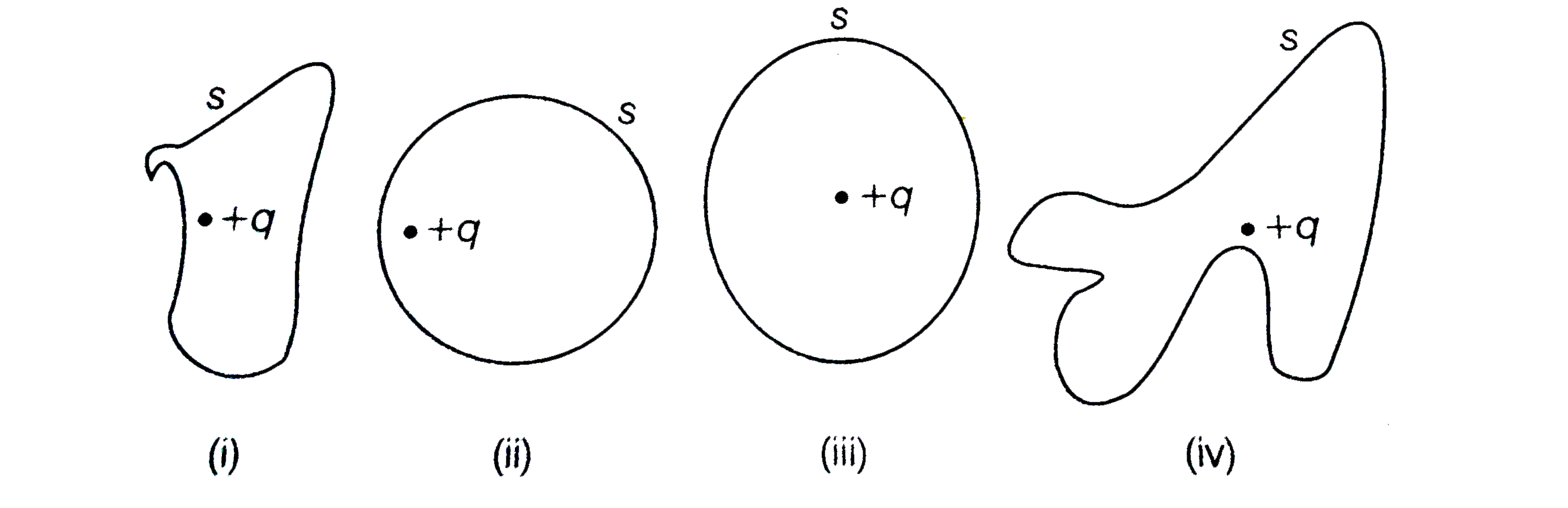 Ain fig. (iv) is the largestBin fig. (iii) is the leastCin fig. (ii) is same as fig.(iii) but is smaller than fig.(iv)DIs the same for all the figures.
Ain fig. (iv) is the largestBin fig. (iii) is the leastCin fig. (ii) is same as fig.(iii) but is smaller than fig.(iv)DIs the same for all the figures.The electric flux through the surface
 Ain fig, (iv) is the largestBin fig, (iii) is the leastCin fig (ii) is same as a fig (iii) but is smaller than that in Fig (iv).Dis the same for all the figures.
Ain fig, (iv) is the largestBin fig, (iii) is the leastCin fig (ii) is same as a fig (iii) but is smaller than that in Fig (iv).Dis the same for all the figures.Electric flux through a cube with charge q inside is
Aqε0Bq6ε0Cq3ε0D0
A closed surface encloses a net charge of −3.60μC. What is the net electric flux through the surface?
(b) The electric flux through a closed surface is found to be 780N−m2/C. What quantity of charge is enclosed by the surface?
(c) The closed surface in part (b) is a cube with sides of length 2.50 cm. From the information given in part (b), is it possible to tell where within the cube the charge is located? Explain.
View SolutionElectric flux through a closed surface in free space enclosing a charge q is
View SolutionS1andS2 are two bellow concentric spheres enclosing Qand3Q respectively as shown in Figure. What is the ration of electric flux through S1andS2? What would be electric flux through S1 ? If air inside S1 is replaced by a medium of dielectric constant 3 ?
View SolutionWhat is the work done in moving a test charge q through a distance of 1 cm along the equatorial axis of an electric dipole ?
View SolutionWhich of the following law gives a relation between the electric flux through any closed surface and the charge enclosed by the surface ?
View Solution