If the mass of moon is M81, where M is the mass of earth, find the distance of the point where gravitational field due to earth and moon cancel each other, from the centre of moon. Given the distance between centres of earth and moon is 60 R where R is the radius of earth
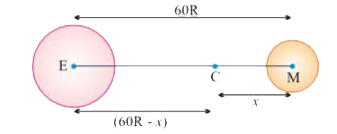
Gravitational field at C due to earth = Gravitational field at C due to earth moon
GM(60R−x)2=GM/81x2
81x2=(60R−x)2
9x=60R−x
x=6R.
Topper's Solved these Questions
- GRAVITATIONBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - GRAVITATIONEXERCISE - SHORT ANSWER TYPE QUESTIONS (3 MARKS)
8 Videos
- GRAVITATIONBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - GRAVITATIONEXERCISE - LONG ANSWER TYPE QUESTIONS (5 MARKS)
6 Videos
- GRAVITATIONBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - GRAVITATIONEXERCISE - MULTIPLE CHOICE QUESTIONS
19 Videos
- DIMENSIONS AND MEASUREMENT BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - DIMENSIONS AND MEASUREMENT EXERCISE - M.C.Q PHYSICAL WORLD & MEASUREMENT
20 Videos
- KINEMATICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - KINEMATICSEXERCISE - M.C.Q.
20 Videos
Similar Questions
If the mass of moon is M81, where M is the mass of earth, find the distance of the point where gravitational field due to earth and moon cancel each other, from the centre of moon. Given the distance between centres of earth and moon is 60 R where R is the radius of earth
View SolutionRadius of moon is 1/4 times that of earth and mass is 1/81 times that of earth. The point at which gravitational field due to earth becomes equal and opposite to that of moon, is (Distance between centres of earth and moon is 60R, where R is radius of earth
View Solution
Knowledge Check
The distance between the earth and the moon is minimum when the moon is in:
AAphelionBPerigeeCPerihelionDApogeeThe distance between the Earth and the Moon is approximately
A484000 kmB384400 kmC340400 kmD364400 kmThe mass of moon is 1/81 of the mass of earth. If gravitational force of earth on moon is F, then the gravitational force of moon on earth is
AF/81BFC9FD81F
Radius of moon is 1/4 times that of earth and mass is 1/81 times that of earth. The point at which gravitational field times that of earth. The point at which gravitational field due to earth becomes equal and opposite to that of moon, is x R from centre of earth. Find the value of x, (Distance between centres of earth and moon is 60R, where R is radius of earth)
View SolutionAt what distance from the centre of the Moon is the point at which the strength of the resultant of the Earth's and Moon's gravitational fields is equal to zero? The Earth's mass is assumed to be η=81 times that of the Moon, and the distance between the centres of these planets n=60 times greater than the radius of the Earth R.
View SolutionThe mass of moon is nearly 10% of the mass of the earth. What will be the gravitational force of the earth on the moon, in comparison to the gravitational force of the moon on the earth?
View SolutionIf the mass of body is M on the surface of the earth ,then its mass on the surface of the moon will be
View SolutionIf the distance between the earth and moon were doubled, the gravitational force between them will be
View Solution
