If △ABC~△DEF,ar(△DEF)=100cm2andABDE=12, then ar(DABC) is
50cm2
25cm2
4cm2
200cm2
The correct Answer is:B, C
To solve the problem, we will follow these steps:
Step 1: Understand the relationship between the two triangles
Given that triangle ABC is similar to triangle DEF, we know that the ratio of their areas is equal to the square of the ratio of their corresponding sides.
Step 2: Write the formula for the ratio of areas
The formula for the ratio of the areas of two similar triangles is:
ar(triangleABC)ar(triangleDEF)=(ABDE)2
Step 3: Substitute the known values
We know:
- ar(triangleDEF)=100cm2
- ABDE=12
Substituting these values into the formula gives:
ar(triangleABC)100=(12)2
Step 4: Calculate the square of the ratio of sides
Calculating the square of 12:
(12)2=14
Step 5: Set up the equation
Now we can set up the equation:
ar(triangleABC)100=14
Step 6: Solve for ar(triangleABC)
To find ar(triangleABC), multiply both sides by 100:
ar(triangleABC)=100×14=25cm2
Final Answer
Thus, the area of triangle ABC is:
ar(triangleABC)=25cm2
---
Given that triangle ABC is similar to triangle DEF, we know that the ratio of their areas is equal to the square of the ratio of their corresponding sides.
The formula for the ratio of the areas of two similar triangles is:
ar(triangleABC)ar(triangleDEF)=(ABDE)2
We know:
- ar(triangleDEF)=100cm2
- ABDE=12
ar(triangleABC)100=(12)2
Calculating the square of 12:
(12)2=14
Now we can set up the equation:
ar(triangleABC)100=14
To find ar(triangleABC), multiply both sides by 100:
ar(triangleABC)=100×14=25cm2
Thus, the area of triangle ABC is:
ar(triangleABC)=25cm2
Topper's Solved these Questions
- TRIANGLEBook:CBSE COMPLEMENTARY MATERIALChapter:TRIANGLEExercise:Short Answer Type Questions-I
Explore 10 Videos
- TRIANGLEBook:CBSE COMPLEMENTARY MATERIALChapter:TRIANGLEExercise:Short Answer Type Questions-II
Explore 25 Videos
- TRIANGLEBook:CBSE COMPLEMENTARY MATERIALChapter:TRIANGLEExercise:Short Answer Type Questions-II
Explore 25 Videos
- SURFACE AREA AND VOLUME Book:CBSE COMPLEMENTARY MATERIALChapter:SURFACE AREA AND VOLUME Exercise:PRACTICE TEST ( SECTION-D)
Explore 1 Video
Similar Questions
If △ABC~△DEF,BC=3EFandar(DABC)=117cm2 find area (△DEF).
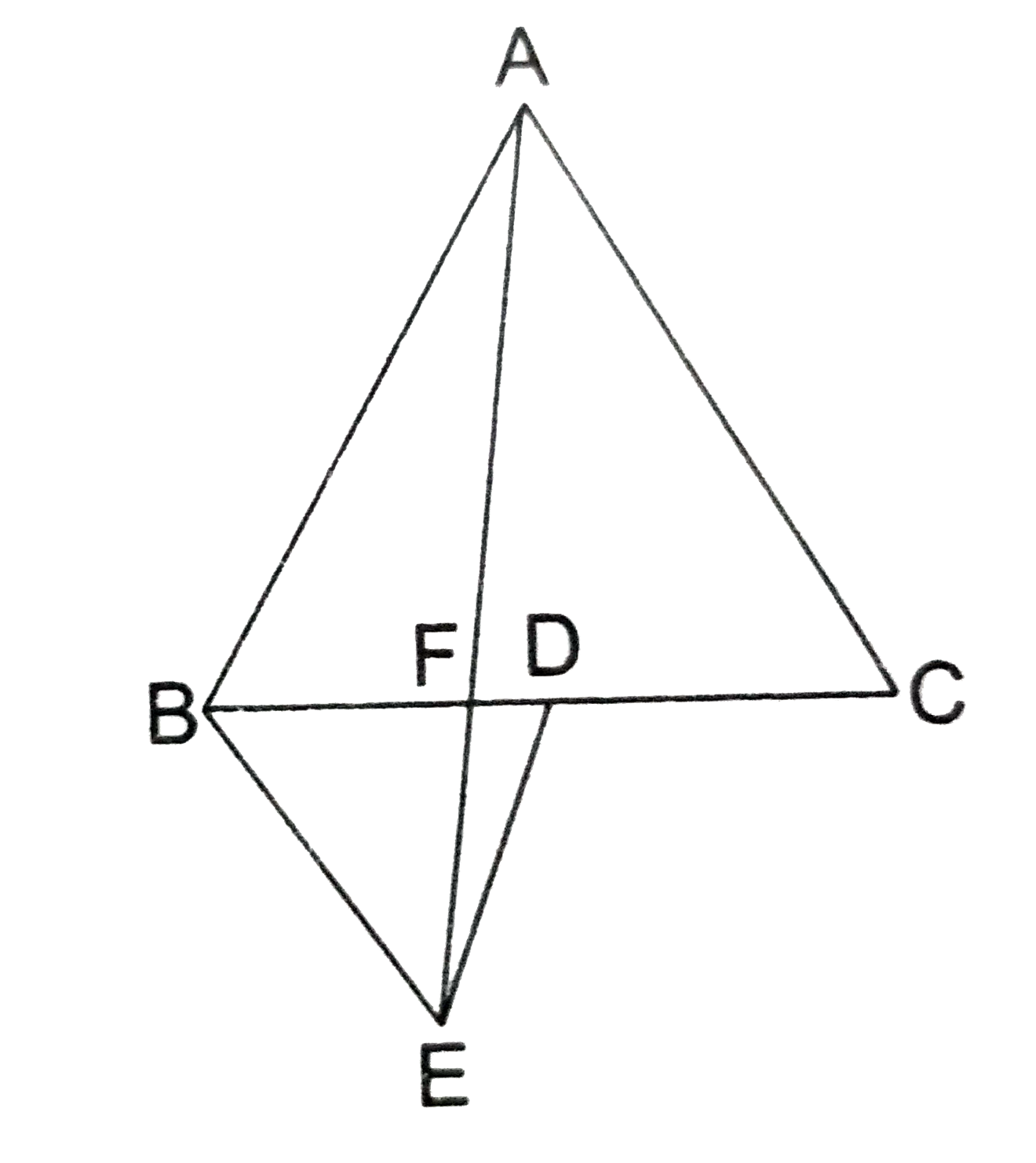
View SolutionIn the adjoining figure, ABC and BDE are two equilateral triangle such that D is the midpoint of BC. If AE intersects BC at F, show that
(i)ar(△BDE)=14ar(△ABC)
(ii)ar(△BDE)=12ar(△BAE)
(iii)ar(△ABC)=2ar(△BEC)
(iv)ar(△BFE)=ar(△AFD)
(v)ar(△BFE)=2ar(△FED)
(vi)ar(△FED)=18ar(△AFC).
View Solution
Knowledge Check
ABCD is a tetrahedron such that each of the △ABC, △ABD and △ACD has a right angle at A. If ar(△ABC)=k1.ar(△ABD)=k2,ar(△BCD)=k3, then ar(△ACD) is
A√k21+k22+k23B√k1k2k3k1+k2+k3C√∣∣k21+k22−k23∣∣D√∣∣k22−k21−k23∣∣If ABCD is a tetrahedron such that each △ABC,△ABDand△ACD has a right angle at A. If ar(△(ABC))=k1,ar(△ABD)=k2,ar(△BCD)=k3, then ar(triangleACD) is
A√k21+k22+k23B√k1k2k3k21+k22+k23C√∣∣(k21+k22−k23)∣∣D√∣∣(k21−k22−k23)∣∣If △ABC - △PQR and BCQR = 14 then ar(△PQR)ar(△ABC) =
A14B41C116D161
If △ABCand△DEF are similar triangles such that ∠A=45∘and∠F=56∘, then find angle C
View Solution△ABC is similar to △DEF. The perimeters of △ABC and △DEF are 40 cm and 30 cm respectively. What is the ratio of (BC+CA) to (EF + FD) equal to?
View SolutionIf △ABC~△EDFand△ABC is not similar to △DEF, then which of the following is not true?
View SolutionIn △ABC, AB=AC and AL is perpendicular to BC at L. In △DEF, DE=DF and DM is perpendicular to EF at M. If (area of △ABC):(area of △DEF)= 9:25 then DM+ALDM−AL is equal to:
त्रिभुज ABC में, AB = AC है तथा AL, BC पर L पर डाला गया लम्ब है| △DEF में, DE = DF है तथा DM, EF पर M पर डाला गया लम्ब है| यदि ( △ABC का क्षेत्रफल ): (△DEF का क्षेत्रफल )= 9 : 25 है, तो DM+ALDM−AL का मान किसके बराबर है ?
View SolutionLet △ABC~△QPR and ar(△ABC)ar(△PQR)=916. If AB=12 cm, BC=6 cm and AC=9 cm then QR is equal to :
View Solution