A boat is moving with a velocity (3ˆi+4ˆj) with respect to ground. The water in river is flowing with a velocity (−2ˆi−4ˆj) with respect to ground.
What is the relative velocity of boat with respect to river ?
To find the relative velocity of the boat with respect to the river, we can follow these steps:
Step 1: Identify the velocities
- The velocity of the boat with respect to the ground is given as:
→Vboat=3^i+4^j
- The velocity of the river (water) with respect to the ground is given as:
→Vriver=−2^i−4^j
Step 2: Use the formula for relative velocity
The formula for the relative velocity of the boat with respect to the river is:
→Vboat, relative=→Vboat−→Vriver
Step 3: Substitute the values
Now, substitute the values of the velocities into the formula:
→Vboat, relative=(3^i+4^j)−(−2^i−4^j)
Step 4: Simplify the expression
When we simplify the expression, we distribute the negative sign:
→Vboat, relative=3^i+4^j+2^i+4^j
Combine like terms:
→Vboat, relative=(3+2)^i+(4+4)^j=5^i+8^j
Step 5: State the final result
The relative velocity of the boat with respect to the river is:
→Vboat, relative=5^i+8^j
---
- The velocity of the boat with respect to the ground is given as:
→Vboat=3^i+4^j
- The velocity of the river (water) with respect to the ground is given as:
→Vriver=−2^i−4^j
The formula for the relative velocity of the boat with respect to the river is:
→Vboat, relative=→Vboat−→Vriver
Now, substitute the values of the velocities into the formula:
→Vboat, relative=(3^i+4^j)−(−2^i−4^j)
When we simplify the expression, we distribute the negative sign:
→Vboat, relative=3^i+4^j+2^i+4^j
Combine like terms:
→Vboat, relative=(3+2)^i+(4+4)^j=5^i+8^j
The relative velocity of the boat with respect to the river is:
→Vboat, relative=5^i+8^j
Topper's Solved these Questions
- KINEMATICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - KINEMATICSEXERCISE - M.C.Q.
20 Videos
- KINEMATICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - KINEMATICSEXERCISE - 5 Marks
2 Videos
- GRAVITATIONBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - GRAVITATIONEXERCISE - MULTIPLE CHOICE QUESTIONS
19 Videos
- KINETIC THEORY OF GASESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Objective question
20 Videos
Similar Questions
A boat is moving with a velocity (3ˆi+ˆj) with respect to ground. The water in the river is moving with a velocity −3ˆi−4ˆj with respect to ground. What is the relative velocity of boat with respect to river ?
View SolutionA boat is moving with a velocity 3ˆi−4ˆj w.r.t ground. The water in the river is moving with a velocity −3ˆi−4ˆj w.r.t ground. What is the relative velocity of boat w.r.t water.
View Solution
Knowledge Check
A boat is moving with a velocity 3ˆi+4ˆj with respect to ground. The water in the river is moving with a velocity −3ˆi−4ˆj with respect to ground. The relative velocity of the boat with respect to water is.
A8ˆjB−6ˆi−8ˆjC6ˆi+8ˆjD5√2)A boat is moving with a velocity 2i + 3j with respect to ground. The water in the river is moving with a velocity -2i - 3j with respect to ground. The relative velocity of the boat with respect to water is
A4jB−4j+6jC4j+6jD6jA boat crossing a river moves with a velocity v relative to still water. The river is flowing with a velocity v/2 with respect to the bank. The angle with respect to the flow direction with which the boat should move to minimize the drift is–
A30∘B60∘C150∘D120∘
A boat stems in a river with velocity 2ˆi+ˆj with respecitve to the ground. The river water flows with a velocity −3ˆi−4ˆj with respect to the ground. What is the relative velocity of boat w.r.t river water ?
View SolutionA boat is traveling upstream in the positive direction of an x axis at 14 km/h with respect to the water of a river. The water is flowing at 8.2 km/h with respect to the ground. What are the (a) magnitude and (b) direction of the boat's velocity with respect to the ground? A child on the boat walks from front to rear at 6.0 km/h with respect to the boat. What are the ( c) magnitude and (d) direction of the child's velocity with respect to the ground?
View SolutionIn a reference frame a man A is moving with velocity (3ˆi−4ˆj)ms−1 and another man B is moving with velocity (ˆi+ˆj)ms−1 relative to A. Find the actual velocity of B.
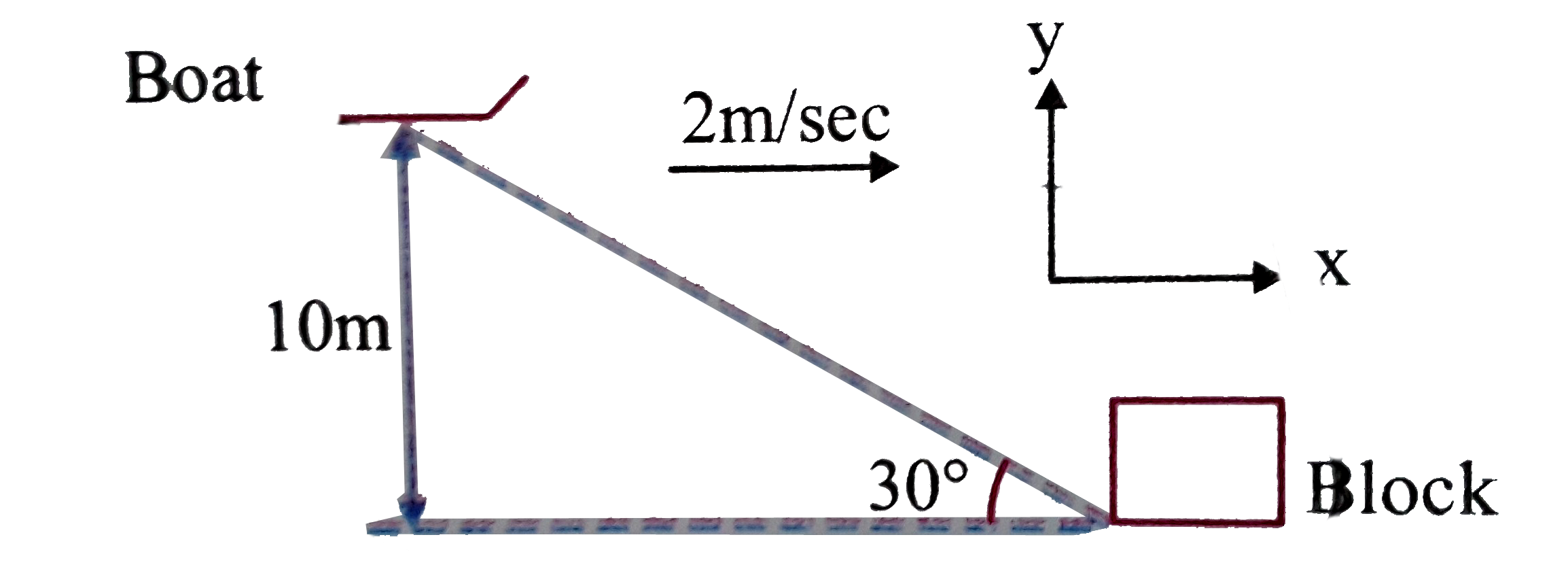
View SolutionA block of mass m in floating in a river flowing with a velocity of 2m/s.A boat is moving behind the block with a velocity of 5m/s with respect to the block as shown.From the boat a stone is thrown with a velocity →v=v1ˆi−vˆj+v3ˆk with respect to the river such that it hits the block.If v1:v2:v3=2√3:2:3 then the velocity of the stone with respect to the ground is (g=10m/s2)
View SolutionA boatman moves his boat with a velocity 'v' (relative to water) in river and finds to his surprise that velocity of river 'u' (with respect to ground) is more than 'v'. He has to reach a point directly opposite to the starting point on another bank by travelling minimum possible distance. Then
View Solution
