Half of the space between parallel plate capacitor is filled with a medium of dielectric constant K parallel to the plates . if initially the capacity is C, then the new capacity will be :
2KC(1+K)
C(K+1)/2
CK/(1+K)
KC
The correct Answer is:A
To solve the problem of finding the new capacitance of a parallel plate capacitor when half of the space is filled with a dielectric medium, we can follow these steps:
Step 1: Understand the Initial Capacitance
The initial capacitance C of a parallel plate capacitor is given by the formula:
C=Aϵ0d
where:
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the distance between the plates.
Step 2: Analyze the New Configuration
In the new configuration, half of the space between the plates is filled with a dielectric material of dielectric constant K, while the other half remains air (or vacuum). This means that the area of the capacitor is now effectively divided into two parts:
- Area with dielectric: A1=A2
- Area without dielectric: A2=A2
Step 3: Calculate the Capacitance of Each Section
1. Capacitance of the section with dielectric C1:
C1=A1⋅Kϵ0d=A2⋅Kϵ0d=KAϵ02d
2. Capacitance of the section without dielectric C2:
C2=A2⋅ϵ0d=A2⋅ϵ0d=Aϵ02d
Step 4: Combine the Capacitances
Since C1 and C2 are in parallel, the total capacitance Ceq can be calculated as:
Ceq=C1+C2
Substituting the values we calculated:
Ceq=KAϵ02d+Aϵ02d
Factoring out the common terms:
Ceq=Aϵ02d(K+1)
Step 5: Relate to the Initial Capacitance
Recall that the initial capacitance C is:
C=Aϵ0d
Thus, we can express Ceq in terms of C:
Ceq=12Aϵ0d(K+1)=C2(K+1)
Final Result
The new capacitance Ceq is:
Ceq=C2(K+1)
The initial capacitance C of a parallel plate capacitor is given by the formula:
C=Aϵ0d
where:
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the distance between the plates.
In the new configuration, half of the space between the plates is filled with a dielectric material of dielectric constant K, while the other half remains air (or vacuum). This means that the area of the capacitor is now effectively divided into two parts:
- Area with dielectric: A1=A2
- Area without dielectric: A2=A2
1. Capacitance of the section with dielectric C1:
C1=A1⋅Kϵ0d=A2⋅Kϵ0d=KAϵ02d
C2=A2⋅ϵ0d=A2⋅ϵ0d=Aϵ02d
Since C1 and C2 are in parallel, the total capacitance Ceq can be calculated as:
Ceq=C1+C2
Substituting the values we calculated:
Ceq=KAϵ02d+Aϵ02d
Factoring out the common terms:
Ceq=Aϵ02d(K+1)
Recall that the initial capacitance C is:
C=Aϵ0d
Thus, we can express Ceq in terms of C:
Ceq=12Aϵ0d(K+1)=C2(K+1)
The new capacitance Ceq is:
Ceq=C2(K+1)
Topper's Solved these Questions
Similar Questions
Two parallel plate capacitors, each of capacitance 40 muF, are connected is series. The space between the plates of one capacitor is filled with a dielectric material of dielectric constant K =4. Find the equivalent capacitacne of the system.
View SolutionIf the distance between the plates of parallel plate capacitor is halved and the dielectric constant of dielectric is doubled, then its capacity will
View Solution
Knowledge Check
If the gap between the plates of a parallel plate capacitor is filled with medium of dielectric constant k=2 , then the field between them
AIncreases by a factor 2BIncreases by a factor √2CDecreased by a factor √2DDecreased by a factor 1/2A parallel plate capacitor has a capacity c. If a medium of dielectric constant K is introduced between plates, the capacity of capacitor becomes
ACKBCK2CK2CDKCHalf of the space between the plates of a parallel plate capacitor is filled with a dielectric material of dielectric constant K . The ramaining half contains air as shown in the figure . The capacitor is now given a charge Q . Then
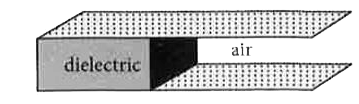 Aelectric field in the dielectric filled region is higher than that in the air - filled region.Bon the two halves of the bottom plate the charge densities are unequal.Ccharge on the half of the top plate above the air filled part is QK+1.Dcapacitance of the capacitor shown above is (1+K)C02, where C0 is the capacitance of the same capacitor with dielectric removed.
Aelectric field in the dielectric filled region is higher than that in the air - filled region.Bon the two halves of the bottom plate the charge densities are unequal.Ccharge on the half of the top plate above the air filled part is QK+1.Dcapacitance of the capacitor shown above is (1+K)C02, where C0 is the capacitance of the same capacitor with dielectric removed.
In figure half of the space between the plates of a parallel plate capacitor is filled with dielectric material of constant K. Then which of the plots are possible?

View SolutionIn a parallel plate capacitor , the capacity increases if
View SolutionIn a parallel plate air capacitor the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity is .
View SolutionWhat will be the capacity of a parallel-plate capacitor when the half of parallel space between the plates is filled by a material of dielectric constant εr ? Assume that the capacity of the capacitor in air is C.
View SolutionA parallel plate capacitor carries a harge Q. If a dielectric slab with dielectric constant K=2 is dipped between the plates, then
View Solution