A 110 V, 60 W lamp is run from a 220 V AC mains using a capacitor in series with the lamp, instead of a resistor then the voltage across the capacitor is about:
110 V
190 V
220 V
311 V
The correct Answer is:B
To solve the problem, we need to find the voltage across the capacitor when a 110 V, 60 W lamp is connected in series with the capacitor to a 220 V AC mains supply.
1. Identify the given values:
- Supply voltage (VS) = 220 V
- Lamp voltage (VR) = 110 V
- Lamp power (P) = 60 W
2. Calculate the current through the lamp:
- The power of the lamp can be expressed as:
P=VR⋅I
- Rearranging gives us:
I=PVR=60W110V≈0.545A
3. Use the voltage relationship in the series circuit:
- In a series circuit, the total voltage is the sum of the voltages across the lamp and the capacitor:
VS=VR+VC
- Rearranging gives:
VC=VS−VR
4. Substituting the known values:
- Substitute VS=220V and VR=110V:
VC=220V−110V=110V
5. Using the Pythagorean theorem for AC circuits:
- In an AC circuit with a capacitor and a resistor, the voltages can be related using:
V2S=V2R+V2C
- Rearranging to find VC:
V2C=V2S−V2R
6. Substituting the values into the equation:
- Substitute VS=220V and VR=110V:
V2C=(220)2−(110)2
V2C=48400−12100=36300
- Taking the square root:
VC=√36300≈190.52V
7. Final result:
- The voltage across the capacitor is approximately:
VC≈190V
Conclusion:
The voltage across the capacitor when the 110 V, 60 W lamp is connected in series with it to a 220 V AC supply is about 190 V.
- Supply voltage (VS) = 220 V
- Lamp voltage (VR) = 110 V
- Lamp power (P) = 60 W
- The power of the lamp can be expressed as:
P=VR⋅I
- Rearranging gives us:
I=PVR=60W110V≈0.545A
- In a series circuit, the total voltage is the sum of the voltages across the lamp and the capacitor:
VS=VR+VC
- Rearranging gives:
VC=VS−VR
- Substitute VS=220V and VR=110V:
VC=220V−110V=110V
- In an AC circuit with a capacitor and a resistor, the voltages can be related using:
V2S=V2R+V2C
- Rearranging to find VC:
V2C=V2S−V2R
- Substitute VS=220V and VR=110V:
V2C=(220)2−(110)2
V2C=48400−12100=36300
- Taking the square root:
VC=√36300≈190.52V
- The voltage across the capacitor is approximately:
VC≈190V
The voltage across the capacitor when the 110 V, 60 W lamp is connected in series with it to a 220 V AC supply is about 190 V.
Similar Questions
Knowledge Check
A 110 V, 60 W lamp is run from a 220 V AC mains using a capacitor in series with the lamp, instead of a resistor then the voltage across the capacitor is about:
A110VB190VC220VD311VA 120V, 620W lamps is run froma 240V, 50Hz mains supply sing a capacitor connected connected in series with the lamp and supply. What is the teoretical value of the capacitor required to operate the lamp at its normal rating?
A3.8μFB6.6μFC0.7μFD13.3μFA diode is connected to 220V(rms) ac in series with a capacitor as shown in fig. The voltage across the capacitor is
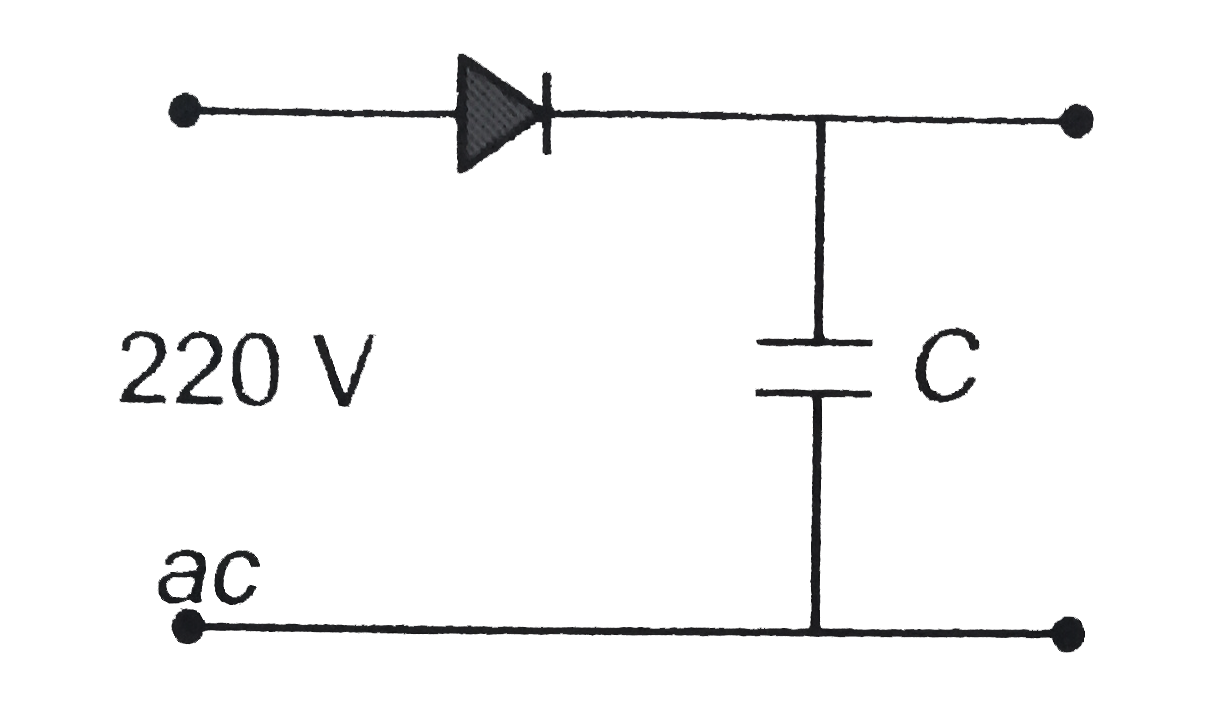 A220VB110VC311.1VD220√2V
A220VB110VC311.1VD220√2V
An ac source of 50 V (r.m.s value) is connected across a series R - C circuit. If the r.m.s voltage across the resistor is 40 V, then the r.m.s voltage across the capacitor is
View SolutionA 60 V -10W lamp is to be run on AC supply of 100 V -50 cycle in series with a suitable capacitor .Find the capactiance of the capacitor that be needed for the purpose.
View SolutionA lamp is connected is series with a a capacitor and an AC source, what happens if the capacity of the capacitor is reduced ?
View SolutionIn a series L−C−R circuit, currenrt in the circuit is 11A when the applied voltage is 220V. voltage across the caspcitor is 200V. If the value of resistor is 20Ω, then the voltage across the unknown inductor is
View SolutionA 20V 5 watt lamp is used in ac main 220V and frequency 50 c.p.s.
Capacitance of capacitor, to be put in series to run the lamp
View Solution
