Two block A and B of masses 5 kg. and 3 kg. respectively rest on smooth horizontal surface with B over A. The coefficient of friction between A and B. 0.5 the maximum horizontal force that can be applied to A so that there will be motion of A and B without separation is :-
15
25
40
50
The correct Answer is:C
To solve the problem, we will follow these steps:
Step 1: Understand the System
We have two blocks, A (5 kg) and B (3 kg), resting on a smooth horizontal surface, with block B on top of block A. The coefficient of friction between the two blocks is 0.5. We need to find the maximum horizontal force F that can be applied to block A so that both blocks move together without separation.
Step 2: Calculate the Normal Force on Block B
The normal force acting on block B is equal to its weight, which is given by:
NB=mB⋅g
where:
- mB=3kg (mass of block B)
- g=10m/s2 (acceleration due to gravity)
Calculating NB:
NB=3kg⋅10m/s2=30N
Step 3: Calculate the Maximum Frictional Force Between A and B
The maximum frictional force Ffriction that can act between blocks A and B is given by:
Ffriction=μ⋅NB
where:
- μ=0.5 (coefficient of friction)
Calculating Ffriction:
Ffriction=0.5⋅30N=15N
Step 4: Determine the Maximum Acceleration of Block B
Using Newton's second law, the maximum acceleration aB of block B can be calculated as:
aB=FfrictionmB
Calculating aB:
aB=15N3kg=5m/s2
Step 5: Calculate the Total Mass of the System
The total mass mtotal of the system (blocks A and B) is:
mtotal=mA+mB=5kg+3kg=8kg
Step 6: Calculate the Maximum Force F Applied to Block A
To ensure that both blocks move together, the force F applied to block A must produce an acceleration equal to aB:
F=mtotal⋅aB
Calculating F:
F=8kg⋅5m/s2=40N
Final Answer
The maximum horizontal force that can be applied to block A so that both blocks A and B move together without separation is:
40N
We have two blocks, A (5 kg) and B (3 kg), resting on a smooth horizontal surface, with block B on top of block A. The coefficient of friction between the two blocks is 0.5. We need to find the maximum horizontal force F that can be applied to block A so that both blocks move together without separation.
The normal force acting on block B is equal to its weight, which is given by:
NB=mB⋅g
where:
- mB=3kg (mass of block B)
- g=10m/s2 (acceleration due to gravity)
NB=3kg⋅10m/s2=30N
The maximum frictional force Ffriction that can act between blocks A and B is given by:
Ffriction=μ⋅NB
where:
- μ=0.5 (coefficient of friction)
Ffriction=0.5⋅30N=15N
Using Newton's second law, the maximum acceleration aB of block B can be calculated as:
aB=FfrictionmB
Calculating aB:
aB=15N3kg=5m/s2
The total mass mtotal of the system (blocks A and B) is:
mtotal=mA+mB=5kg+3kg=8kg
To ensure that both blocks move together, the force F applied to block A must produce an acceleration equal to aB:
F=mtotal⋅aB
Calculating F:
F=8kg⋅5m/s2=40N
The maximum horizontal force that can be applied to block A so that both blocks A and B move together without separation is:
40N
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 2 SECTION-F :- Direction of friction, Pulley Block system on horizontal plane, Pulley Block system on inclined plane, Two Block system, Two Block on Inclined plane
3 Videos
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 3 Section-A
22 Videos
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 2 SECTION-D :- Spring force and spring cutting problems
1 Video
- MODERN PHYSICS -1BOOK - MOTIONCHAPTER - MODERN PHYSICS -1EXERCISE - EXERCISE-4 ( LEVEL- II)
39 Videos
- NLM & FRICTIONBOOK - MOTIONCHAPTER - NLM & FRICTIONEXERCISE - EXERCISE-4 ( LEVEL-II)
15 Videos
Similar Questions
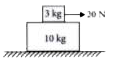
A 3kg block is placed over a 10kg block and both are placed on a smooth horizontal surface. The coefficient of friction between the blocks is 0.2. IF a horizontal force of 20N is applied to 3kg block. Find the acceleration of the two blocks.
View SolutionA block A of mass 2 kg rests on another block B of mass 8 kg which rests on a horizontal floor . The coefficient of friction between A and B is 0.2 while that between B and floor is 0.5 . When horizontal force F = 25 N is applied on the block B , the force of friction between A and B is (in N) .
View Solution
Knowledge Check
Two block A and B of masses 6kg and 3kg rest on a smooth horizontal surface as shown in figure If coefficient of friction between A and Bb is 0.4 the maximum horizontal force which can make them move without separation is
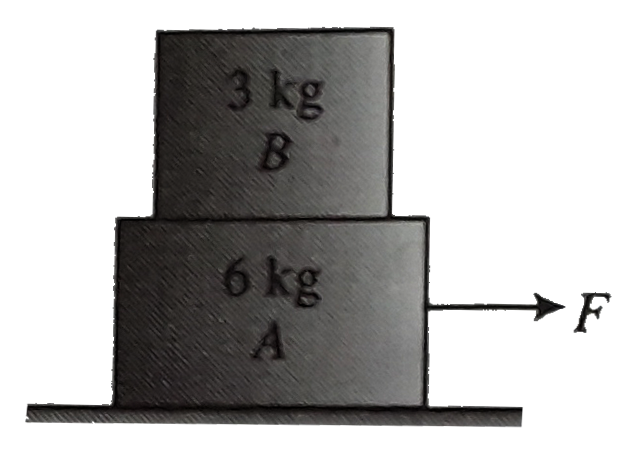 A72NB40NC36ND20N
A72NB40NC36ND20NTwo block (A) 2kg and (B) 5kg rest one over the other on a smooth horizontal plane. The cofficient of static and dynamic friction between (A) and (B) is the sae and equal to 0.60. The maximum horizontal force that can be applied to (B) in order that both (A) and (B) do not have any relative motion:
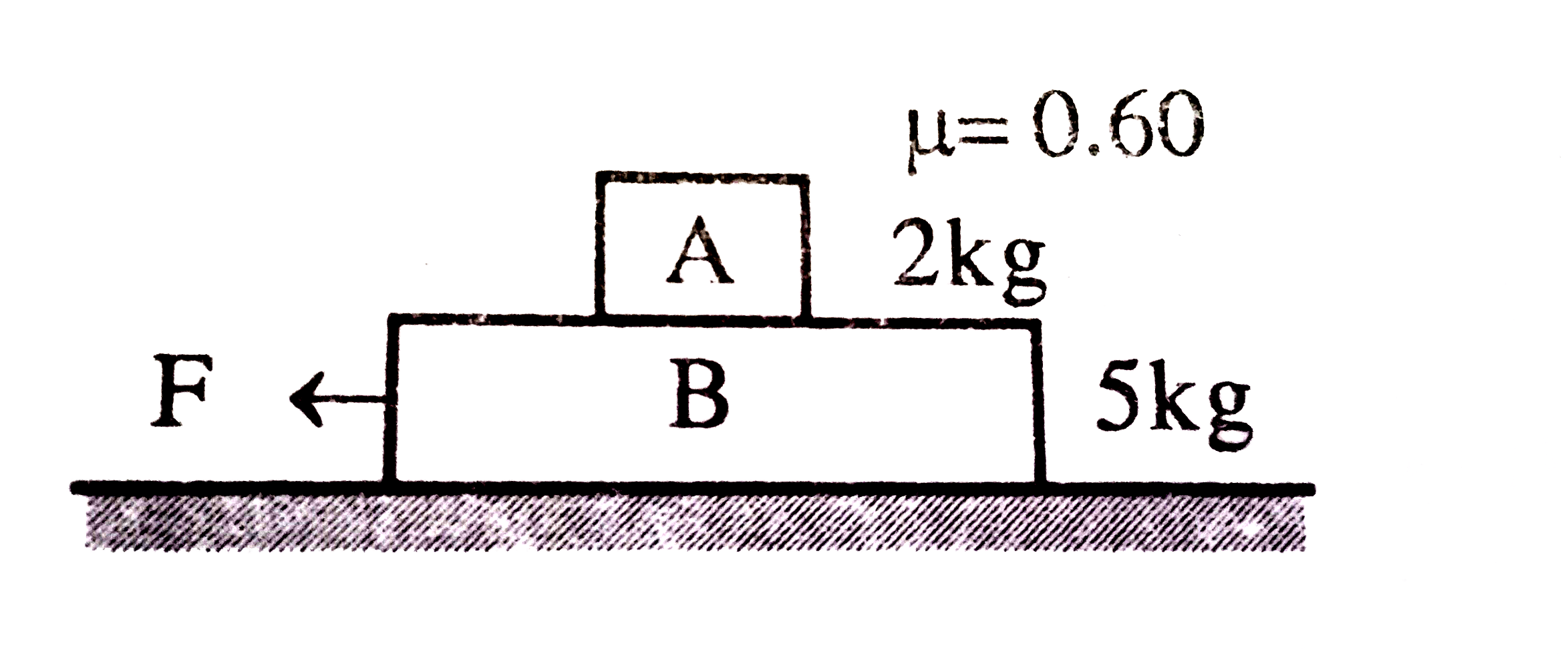 A42NB42kgfC5.4kgfD1.2N
A42NB42kgfC5.4kgfD1.2NIn the arrangement shown in figure, m_A = m_B = 2kg. String is massless and pulley is frictionless. Block B is resting on a smooth horizontal surface, while friction coefficient beteen block A and B is μ=0.5 . the Maximum horizontal force F that can be applied so that block A does not slip over block B is.
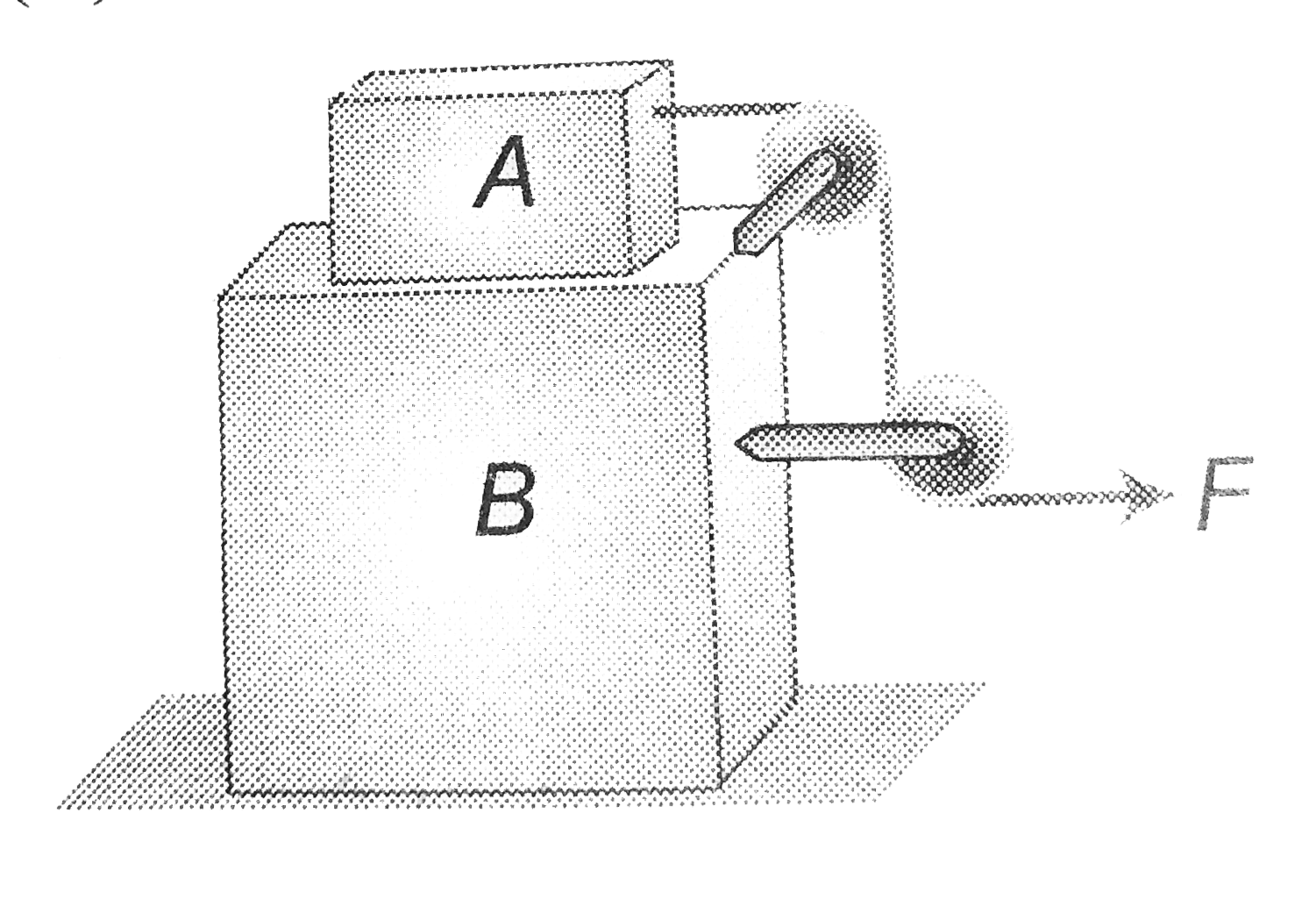 A25NB40NC30ND20N
A25NB40NC30ND20N
Two block "A&B" of mass MA=1kg&MB=3kg are kept on a smooth table as shown in the figure.The coefficient of friction between "A&B" is "0.2" .The maximum force "F" that can be applied on "B" horizontally,so that the block "A" do not slide over the block "B" is Take g=10ms2andk=400Nm)
View SolutionTwo blocks A and B of masses 2kg and 3kg respectively, are placed on a horizontal surface as shown (one on top of other). Find the ratio of magnitude of normal reaction between A and B to that between B and the surface, respectively
View SolutionA block A of mass m kg lies on a block B of mass m kg. B in turn lies on smooth horizontal plane. The coefficient of friction between A and B is μ. Both the blocks are initially at rest. A horizontal force F is applied to lower block B at t = 0 till the lower block B undergoes a displacement of magnitude L. Match the statements in Table-1 with the results in Table-2.

View SolutionA block A of mass 2kg is placed over smooth block B of mass 4kg which is placed over a smooth horizontal floor The coefficient of friction between A and B is 0.4 when a horizontal forces of magnitude 10N is applied on A the acceleration of block of A and B are
View SolutionTwo blocks A and B of masses 10 kg and 15 kg are placed in contact with each other rest on a rough horizontal surface as shown in the figure. The coefficient of friction between the blocks and surface is 0.2. A horizontal force of 200 N is applied to block A. The acceleration of the system is (Take g=10ms−2)
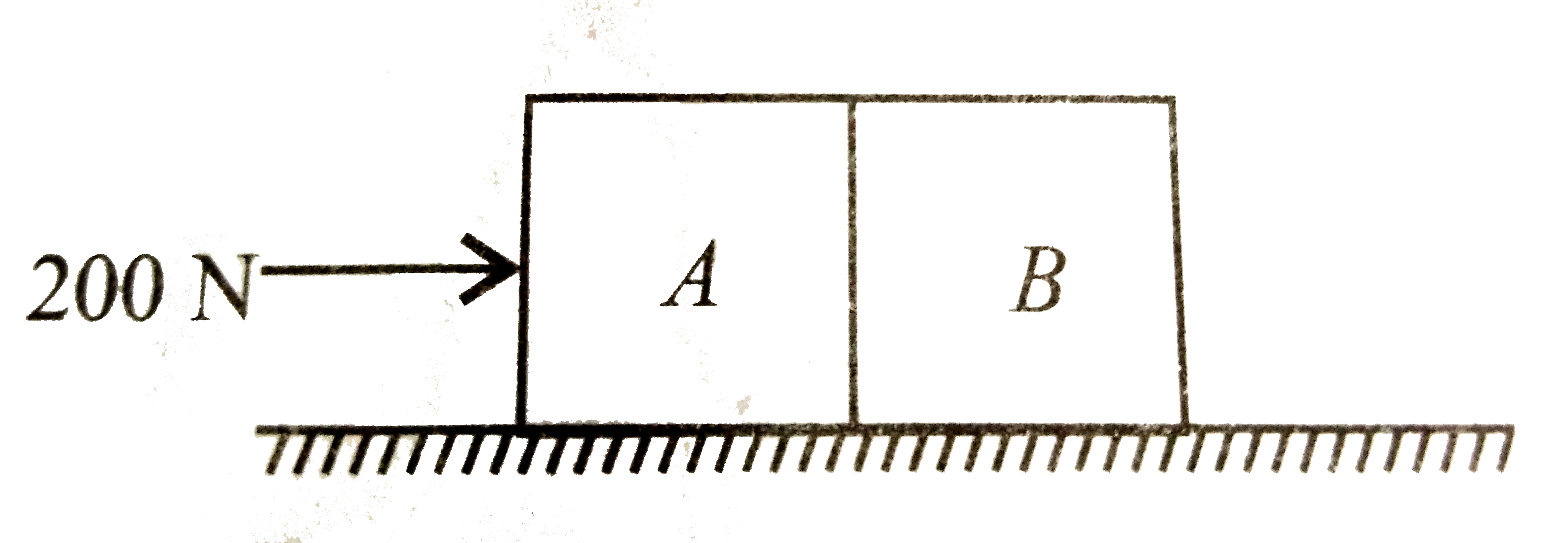
View Solution
