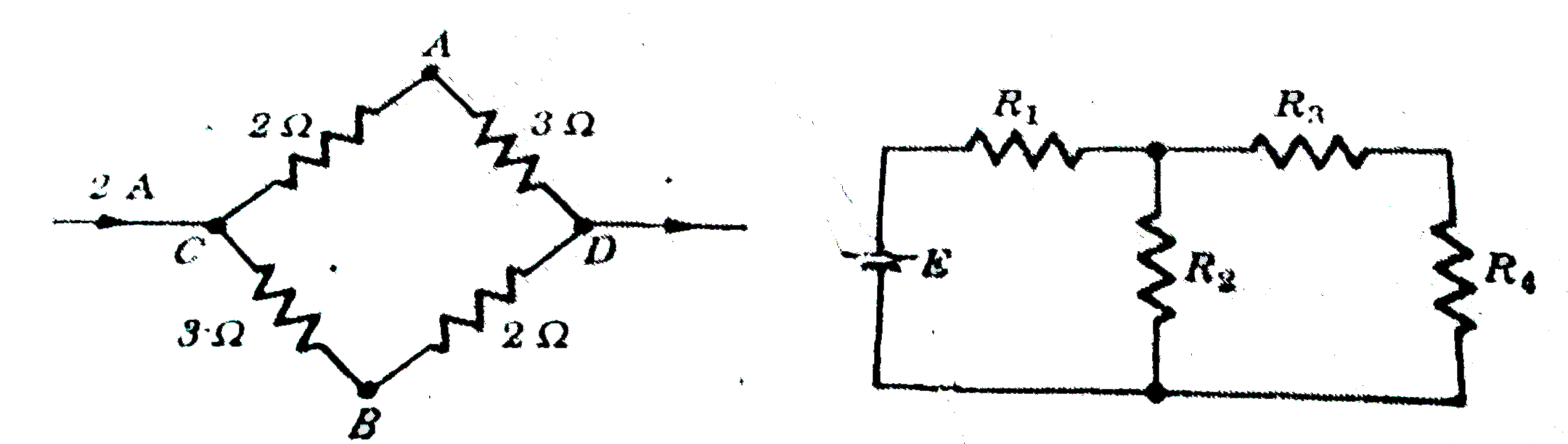Figure shows two rings having charges Q and −√5Q. Find potential difference a and B i.e. (VA−VB).
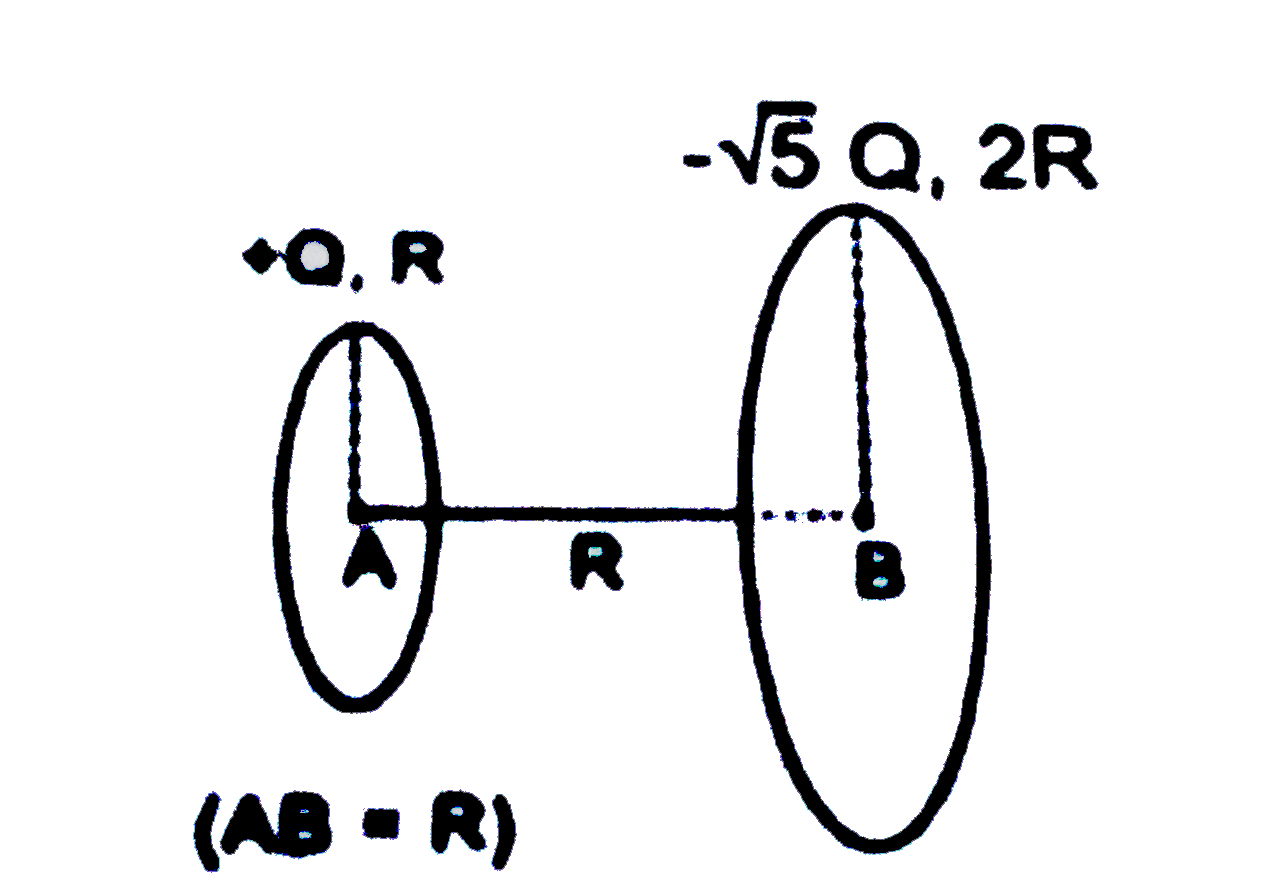
VA=KQR+K(−√5Q)√(2R)2+(R)2
VB=K(−√5Q)2R+K(Q)√(R)2+(R)2
From above we can easily find VA−VB.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - SELF MISCELLANEOUS PROBLEM (Example )
15 Videos
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - SELF PRACTICE PROBLEM
27 Videos
- ELECTRONICS - SEMI CONDUCTORBOOK - MOTIONCHAPTER - ELECTRONICS - SEMI CONDUCTOREXERCISE - EXERCISE - 3
29 Videos
- ELECTROSTATICS - I BOOK - MOTIONCHAPTER - ELECTROSTATICS - I EXERCISE - EXERCISE - 4 (Level -II) PREVIOUS YEAR - JEE ADVANCED
13 Videos
Similar Questions
Knowledge Check
Potential difference between points A and B (i.e. VA−VB) is -----
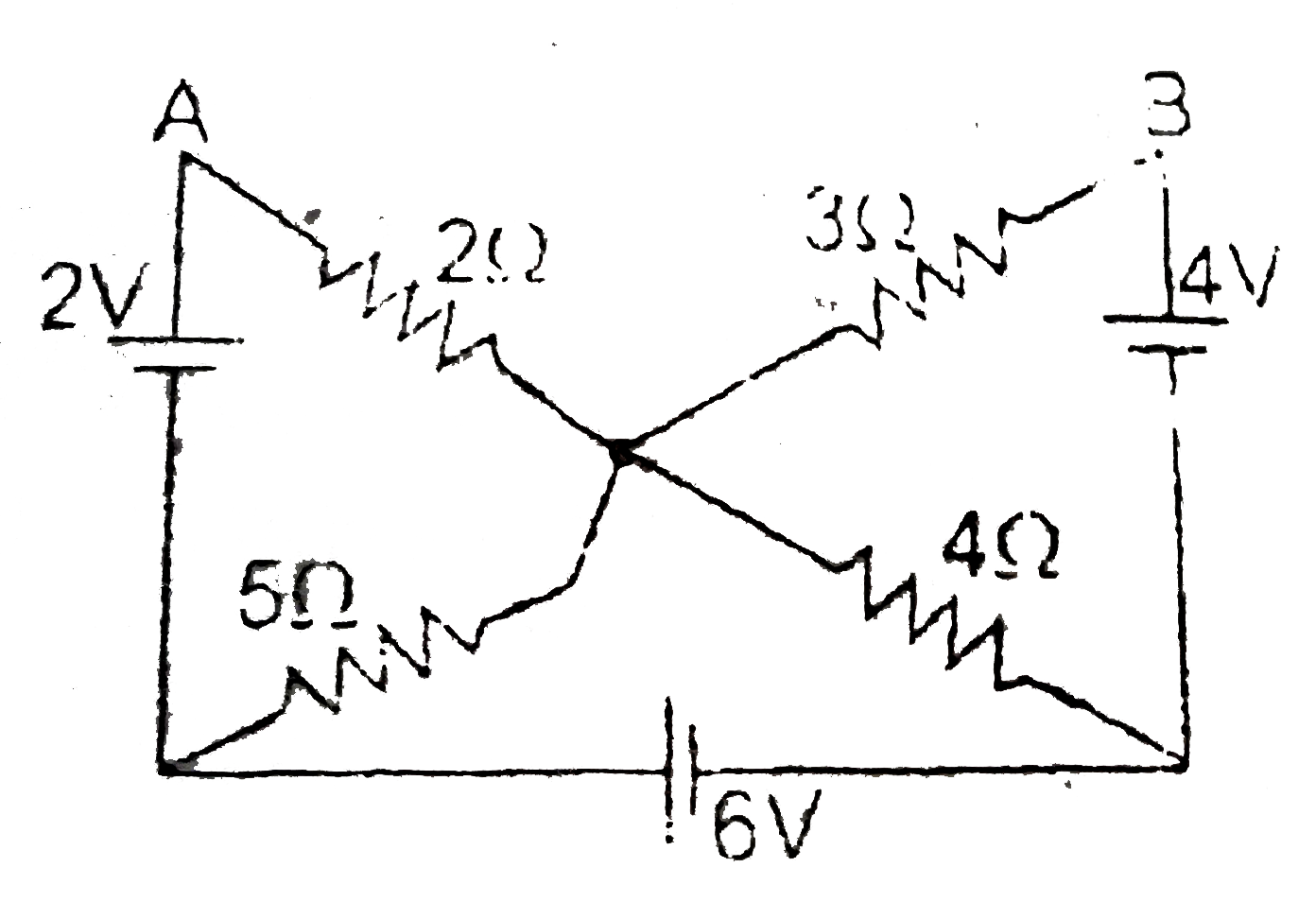 A2VB4VC8VD12 V
A2VB4VC8VD12 VAsseration: A point charge q is placed at centre of spherical cavity inside a spherical conductor as shown. Another point charge Q is placed outside the conductor as shown. Now as the point charge Q is pushed away from conductor, the potential difference (VA−VB) between two points A and B within the cavity of sphere remains constant.

Reason: The electric field due to charges on outer surface of conductor and outside the conductor is zero at all points inside teh conductor.AIf both assertion and reason are true and reson is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf assertion and reason both are false.In figure two points A and B are located in a region of electric field . The potential difference VB−VA is
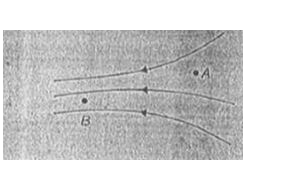 APositiveBNegativeCZeroDNone of these
APositiveBNegativeCZeroDNone of these
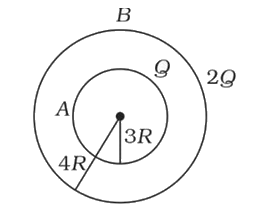
In the figure shown, conducting shells A and B have charges Q and 2Q distributed uniformly over and B. Value of VA−VB k is kQnR. Find n(k=14π∈0
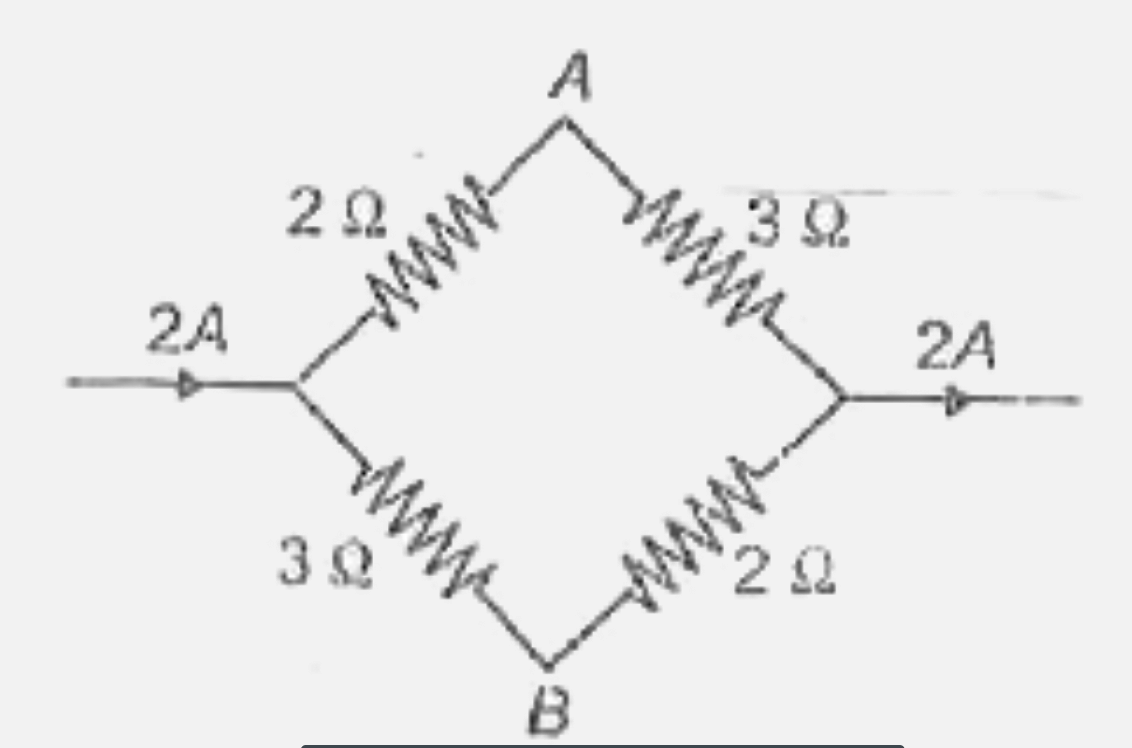
View SolutionPotential difference VA−VB in the network shown in
View SolutionFigures (a) and (b) show the field lines of a positive and negative point charge respectively
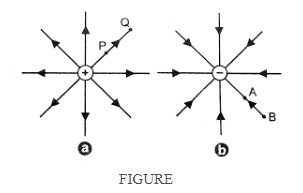
(a) Give the signs of the potential difference VP−VQ,VB–VA.
(b) Give the sign of the potential energy difference of a small negative charge between the points Q and P, A and B.
(c) Give the sign of the work done by the field in moving a small positive charge from Q to P.
(d) Give the sign of the work done by the external agency in moving a small negative charge from B to A.
(e) Does the kinetic energy of a small negative charge increase or decrease in going from B to A?
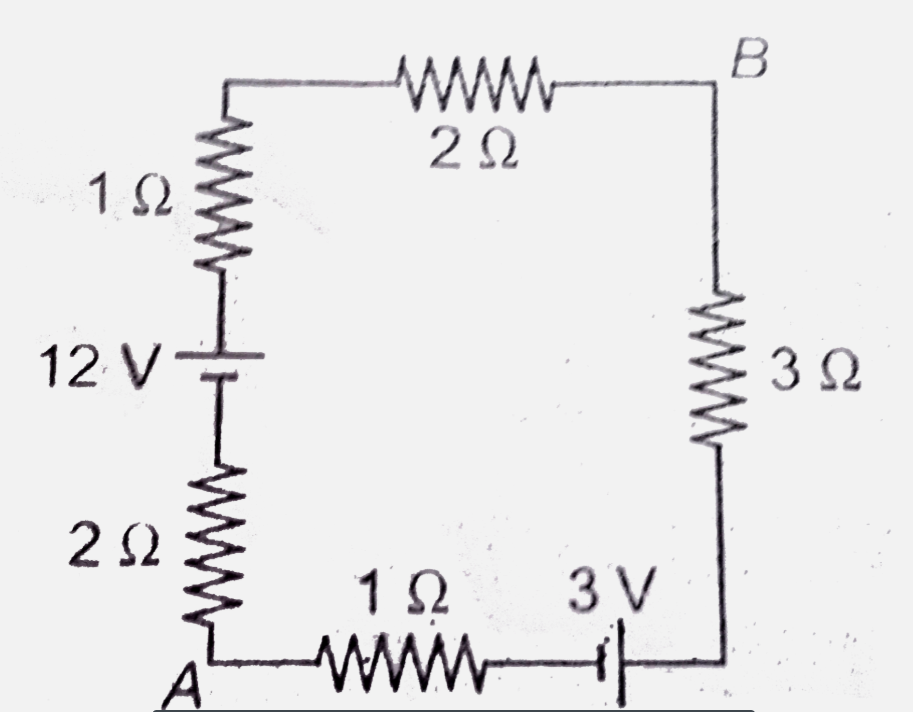
View SolutionPotential difference VB−VA in the network shown is
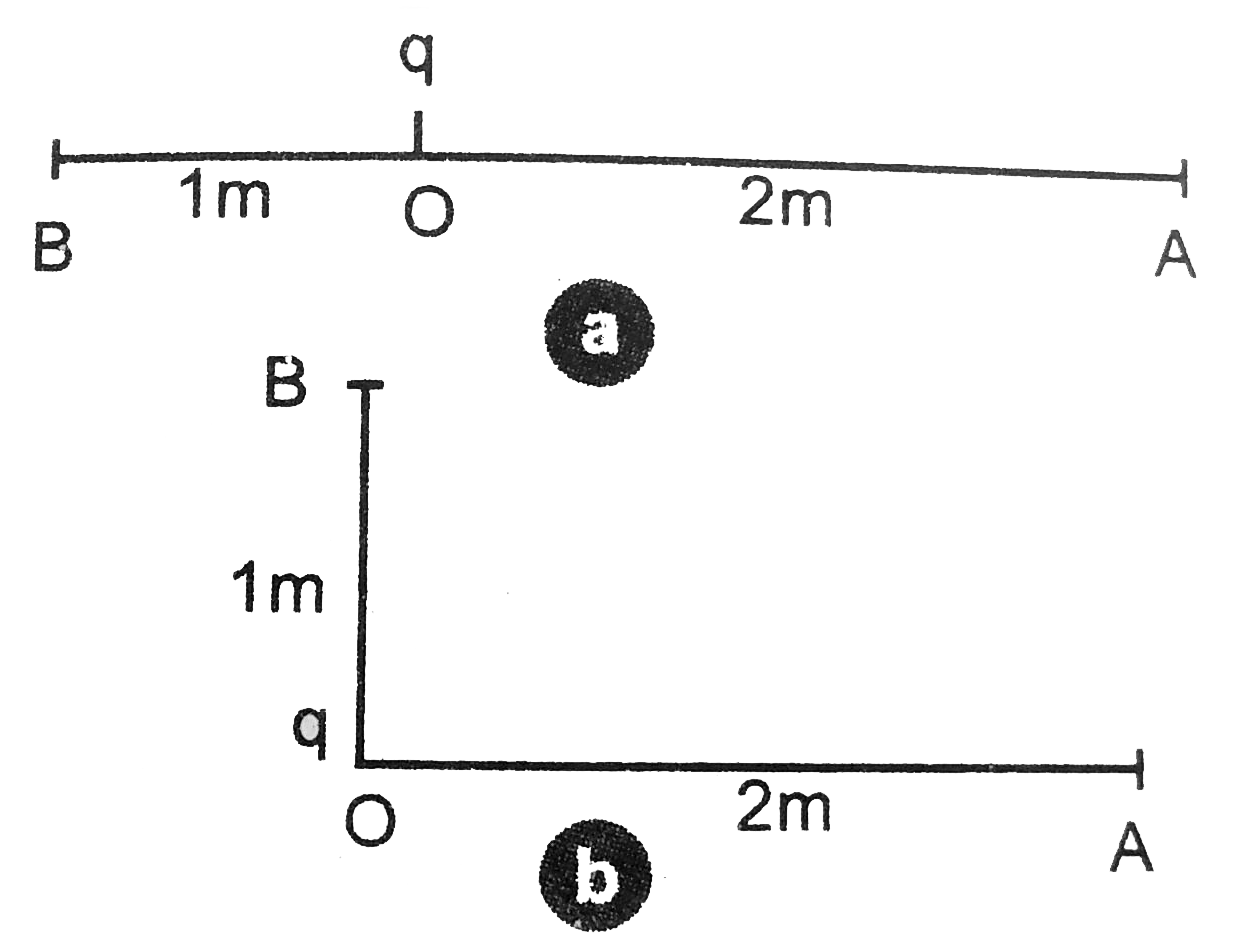
View SolutionA charge q=+1μC is held at 0 between two points A and B such that AO=2mandBO=1m Calculate the value of potential differences (VA−VB). What will be the value of potential differences (VA−VB) if position of B is charged as shown in Fig ?
View Solution