Show that if the flux of magnetic induction through a coil changes from ϕ1 to ϕ2 then the charge q that flows through the circuit of total resistance R is given by q=ϕ2−ϕ1R where R is the resistance of the coil.
Let ϕ be the instantaneous flux. Then dϕdt is the instantaneous rate of change of flux which is equal to the magnitude of the instantaneous emf. So the current in the circuit |i|=1R(dϕdt) , since the current is the rate of flow of charge, that is, i=dqdt
q=∫rq=t=t∫t=0(1R.dϕdt)dt
where τ is the time during which change takes place. but at t=0,ϕ=ϕ1, and at t=t,ϕ=ϕ2
∴:.q=(1)/(R)underset(phi=phi_(1))overset(phi=phi_(2))intdphi=(phi_(2)-phi_(1))/(R)
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - MOTIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-1
25 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MOTIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-2 (LEVEL-I)
16 Videos
- ELECTRO MAGNETIC WAVES BOOK - MOTIONCHAPTER - ELECTRO MAGNETIC WAVES EXERCISE - EXERCISE - 3 (SECTION - B)
8 Videos
- ELECTROMAGNETISMBOOK - MOTIONCHAPTER - ELECTROMAGNETISMEXERCISE - Exercise
136 Videos
Similar Questions
The magnetic flux linked with a coil of N turns and resistance R changes from ϕ1 to ϕ2. Show that the charge that has passed through the coil is
q=−N(ϕ2−ϕ1)R.
View SolutionThere lis a coil of resistance R. Calculate amount of charge that flows through the coil if associated fluc is increased from ϕ1 to ϕ2 .
View Solution
Knowledge Check
The total electric flux ϕ through the entire area of a closed surface is given by
Aϕ=∮→E×→dsBϕ=∮→E⋅→dsCboth 'a' and 'b'Dnone of theseIn Fig. 6, l and m are two parallel tangents to a circle with centre O, touching the circle at A and B respectively. Another tangent at C intersects the line at D and in at E. Prove that ZDOE=90.
Aa3R2bBa3RbCa3R6bDa3R3bA time varying magnetic flux passing through a coil is given by ϕ=xt2, if at t=3s, the emf induced is 9 V, then the value of x is
A0.66Wb/s2B−0.66Wb/s2C1.5Wb/s2D−1.5Wb/s2
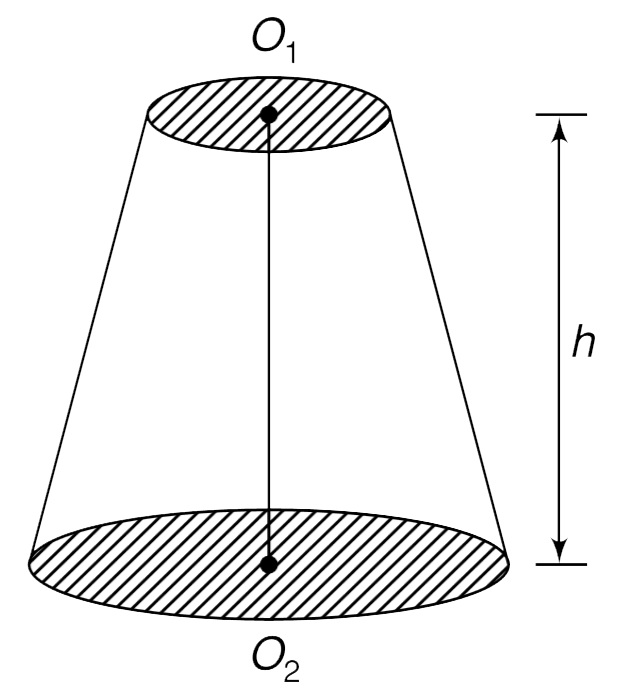
A frustum is cut from a right circular cone. The two circular faces have radii R and 2R and their centers are at O1 and O2 respectively. Height of the frustum is h=3R. When a point charge Q is placed at O1, the flux of electric field through the circular face of radius 2R is ϕ1 and when the charge Q is placed at O2, the flux through the other circular face is ϕ2. find the ratio ph1ϕ2
View SolutionThe charge flowing through a resistance R varies with time t as Q=at−bt2. The total heat produced in R is
View SolutionIf the electric flux entering and leaving an enclosed surface are ϕ1andϕ2 respectively, then the electric charge present within the surface is
View SolutionThe charge flowing through a resistance R varies with time tasQ=at−bt2. The total heat produced in R is
View SolutionThe charge flowing through a resistance R varies with time tasQ=at−bt2. The total heat produced in R is
View Solution
