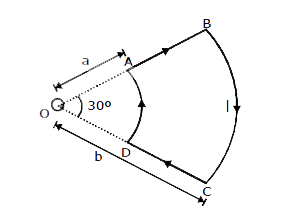
The magnitude of the magnetic field (B) due to loop ABCD at the origin (O) is
zero
μ0(b−a)24ab
μ0I4π[b−aab]
μ0I4π[2(b−a)+π3(a+b)]
The correct Answer is:B
Step by step video, text & image solution for The magnitude of the magnetic field (B) due to loop ABCD at the origin (O) is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MAGNETISM BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-4 (LEVEL-II)
40 Videos
- MAGNETISM BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-3 (Level-II)
23 Videos
- LOGIC GATESBOOK - MOTIONCHAPTER - LOGIC GATESEXERCISE - EXERCISE - 2
10 Videos
- MAGNETISM -1BOOK - MOTIONCHAPTER - MAGNETISM -1EXERCISE - Exercise - 3 SECTION -A
56 Videos
Similar Questions
A circular loop of radius 12 cm carries a current of 7.2 A. A flat coil of radius 0.82 cm, having 50 turns and a current of 1.3 A, is concentric with the loop. The plane of the loop is perpendicular to the plane of the coil. Assume the loop's magnetic field is uniform across the coil. What is the magnitude of (a) the magnetic field produced by the loop at its centre and (b) the torque on the coil due to the loop ?
View SolutionFind the magnitude of the magnetic field at the origin O due to very long conductor carrying current I of the shape as shown in figure.
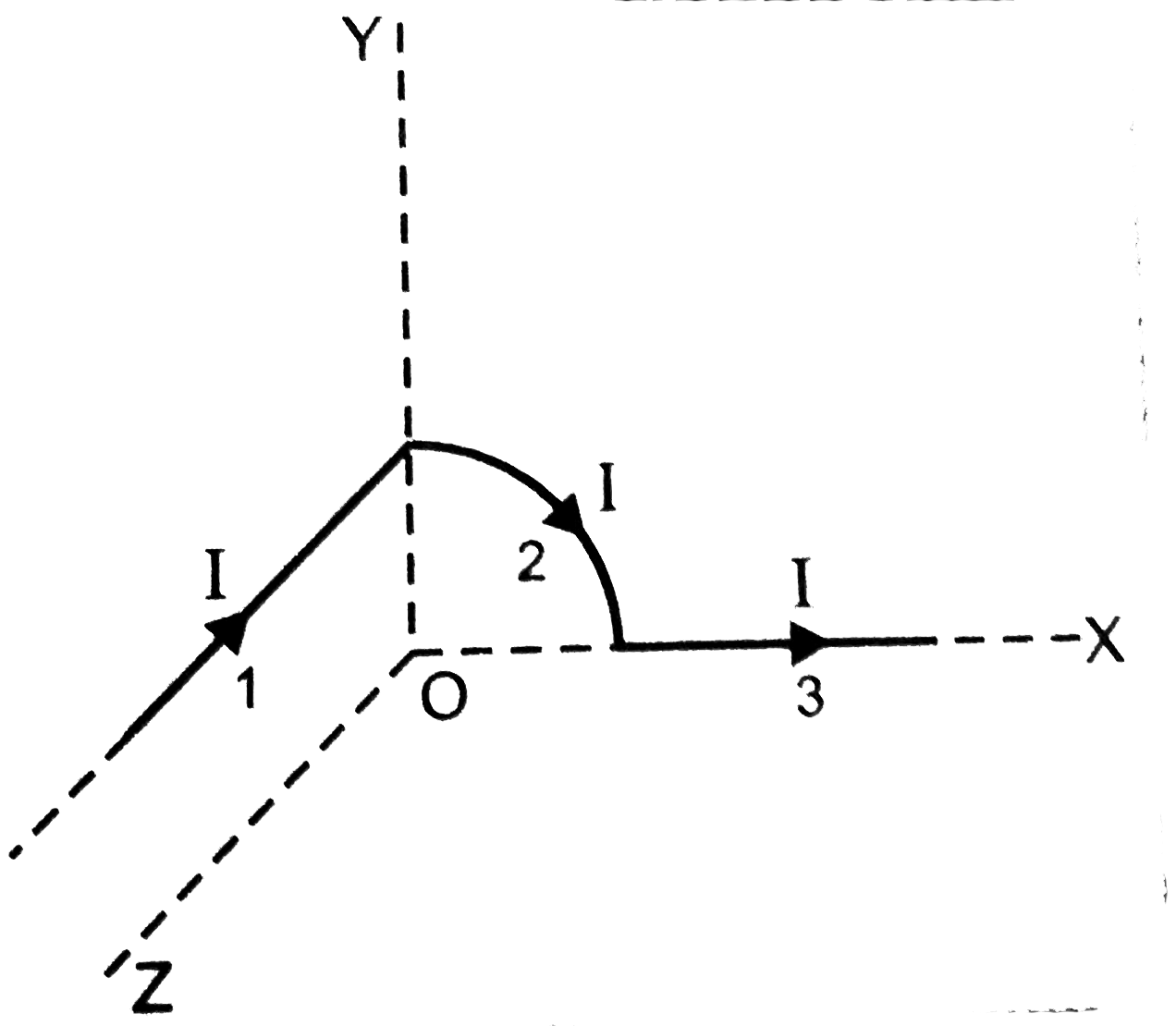
View Solution
Knowledge Check
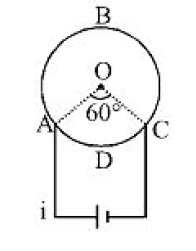
A current loop ABCD is held fixed on the plane of the paper as shown in figure. The arcs BC(radius=b)andDA(radius=a) of the loop are joined by two straight wires ABandCD at the origin O is 30^(@).A¬herstraightth∈wirewithsteadycurrentI_(1)flow∈goutofthepla≠ofthepaperiskeptattheorig∈.<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/JMA_MCM_C14_119_Q01.pngwh=80%mx-autosty≤=background:white;><br>Themagnitudeofthemag≠ticfield(B)due→thel∞pABCD at the origin (o) is :
Aμ0I(b−a)24abBμ0I4π[b−aab]Cμ0I4π[2(b−a)+π/3(a+b)]Dzero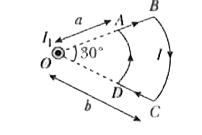
The magnitude of the magnetic filed B due to the loop ABCD at origin (O) isAzeroBμ0I(b−a)24πabCμ0I(b−a)4πabDμ0I4π[2(b−a)+π3(a+b)].A current - carrying loop is shown in the figure. The magnitude of the magnetic field produced at a point O is
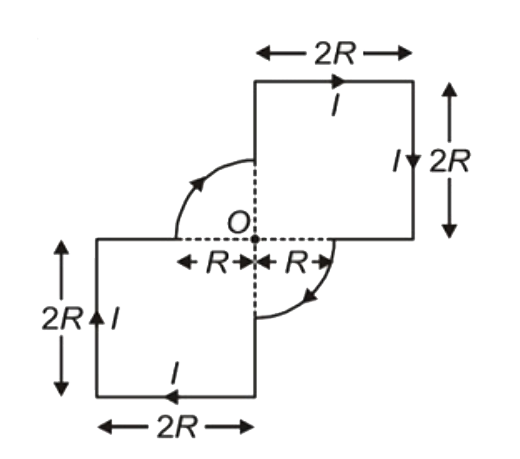 Aμ0I4RBμ0I2R(1+√2π)Cμ0I4R(1+√2π)Dμ04R(1+2√2π)
Aμ0I4RBμ0I2R(1+√2π)Cμ0I4R(1+√2π)Dμ04R(1+2√2π)
A cell is connected between the points A and C of a circular conductor ABCD of centre 'O'.∠AOC=60∘. IfB1andB2 are the magnitudes of the magnetic fields at O due to the currents in ABC and ADC respectively, the ratio B1B2, is
View SolutionA square loop of wire, edge length a, carries a current i. Compute the magnitude of the magnetic field produced at a point on the axis of the loop at a distance x from the centre.
View SolutionMagnetic Field || Wire || Loop
View Solution(a) State Bio-Savart's law in vector form expressing the magnetic field due to an element →dl carrying current I at a distance r from the element.
(b) Write the expression for the magnitude of the magnetic field at the centre of a circular loop of radius 'R' carrying a steady current I. Draw the field lines due to the current loop.
View SolutionThe magnetic moment of a circular current loop is 2.3×10−5Am2. What is the magnitude of the magnetic field on the axis of the loop at a distance of 1 cm from the loop?
View Solution
