An electron is released from the origin at a place where a uniform electric field E and a uniform magnetic field B exist along the negative y-axis and the nagative z-axis respectively. Find the displacement of the electron along the y-axis when its velocitybecomes perpendicular to the electric feld for the first time.

Video Solution

Text Solution

Verified by Experts

Let us take axes as shown in figure. According to the right-handed system, the Z-axis is upward in the figure and hence the magnetic field is shown downwards. At any time, the velocity of the electron may be written as
$\to u = u_{x} \to i + u_{y} ˆ j$
The electric and magentic field may be written as
$\to E = - E \to j and \to B = - B ˆ k$
respectivley. The force on the electron is
$\to F = - e (\to E + \to u \times \to B) = e \to E \to j + e B (u_{y} \to i - u_{x} \to j)$
Thus, $F_{x} = e u_{y} B and F_{y} = e (E - u_{x} B)$ .
The components of the acceleration are
$a_{x} = \frac{d u_{x}}{d t} = \frac{e B}{m} u_{y} ... (i)$
and $a_{y} = \frac{d u_{y}}{d t} = \frac{e}{m} (E - u_{x} B) ... (i i)$
We have,
$\frac{d^{2} u_{y}}{{d t}^{2}} = - \frac{e B}{m} \frac{d u_{x}}{d t} = - \frac{e B}{m} . \frac{e B}{m} u_{y} = - ω^{2} u_{y}$
where $ω = \frac{e B}{m} ... (i i i)$
This equation is similar to that for a simple harmonic motion. Thus,
$u_{y} = A sin (ω t + δ) ... (i v)$
and hence,
$\frac{d u_{y}}{d t} = A ω cos (ω t + δ) ... (v)$
At $t = 0$ , $u_{y} = 0 and \frac{d u_{y}}{d t} = \frac{F_{y}}{d t} = \frac{e E}{m}$
Putting in (iv) and (v)
$δ = 0 and A = \frac{e E}{m ω} \frac{E}{B}$
Thus, $u_{y} = \frac{E}{B} sin ω t$
The path of the electron will be perpendicular to the Y-axis when $u_{y} = 0$ . This will be the case for the first time at t where
$sin ω t = 0$
or, $ω t = π or, t = \frac{π}{ω} = \frac{π m}{e B}$
Also, $u_{y} = \frac{d y}{d t} = \frac{E}{B} sin ω t$
or, $y \int 0 d y = \frac{E}{B} sin ω t d t or, y = \frac{E}{B ω} (1 - cos ω t)$
At $t = \frac{π}{ω}$ ,
$y = \frac{E}{B ω} (1 - cos π) = \frac{2 E}{B ω}$
Thus, the displacement along the Y-axis is
$\frac{2 E}{B ω} = \frac{2 E m}{B e B} = \frac{2 E m}{e B^{2}}$

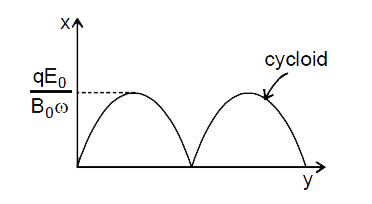
$a_{x} = \frac{q}{m} E_{0} sin ω t$
$v_{x} \int 0 d v_{x} = \frac{q E_{0}}{m} t \int 0 sin ω t d t$
$\Rightarrow v_{x} = \frac{q E_{0}}{m ω} (1 - cos ω t)$
$x \int 0 d x = \frac{q E_{0}}{m ω} t \int 0 (1 - cos ω t) . d t$
$\Rightarrow x = \frac{q E_{0}}{m ω} [t - \frac{sin ω t}{ω}]$

Updated on:21/07/2023

A uniform electric field and a uniform magnetic field are acting along the same direction in a certain region. If an electron is projected in the same direction then the electron
Aspeed will increase
Bwill turn towards left of direction of motion
Cwill turn towards right of direction of motion
Dspeed will decrease
An electron is released from rest in a region of uniform electric and magnetic fields acting parallel to each other. The electron will
Amove in a straight line.
Bmove in a circle.
Cramain stationary.
Dmove in a helical path.
A uniform electric field and a uniform magnetic field are acting along the same direction in a certain region. If an electron is projected along the direction of the fields with a certain velocity then
Aits velocity will increase
Bits velocity will decrease
Cit will turn towards left of direction of motion
Dit will turn towards right of direction of motion