A statue 4 meters high sits on a column 5.6 meters high . How far from the column must a man, whose eye level is 1.6 meters from the ground, stand in order to see the statue at the greatest angle ?
The correct Answer is:4√2 m
To solve the problem of how far from the column a man must stand to see the statue at the greatest angle, we can follow these steps:
Step 1: Understand the Setup
We have a statue that is 4 meters high sitting on a column that is 5.6 meters high. Therefore, the total height of the statue from the ground is:
Total height=Height of column+Height of statue=5.6+4=9.6 meters
The man's eye level is 1.6 meters from the ground.
Step 2: Define the Variables
Let x be the distance from the base of the column to the man. The angles we are interested in are:
- α: the angle of elevation from the man's eye level to the top of the statue.
- θ: the angle of elevation from the man's eye level to the top of the column.
Step 3: Write the Tangent Functions
Using the definition of tangent for the angles:
- For angle α:
tan(α)=Height of statueDistance from man to column=4x
- For angle θ:
tan(θ)=Height of columnDistance from man to column=5.6x
Step 4: Use the Angle Addition Formula
To find the angle θ+α, we can use the tangent addition formula:
tan(θ+α)=tan(θ)+tan(α)1−tan(θ)tan(α)
Substituting the expressions for tan(θ) and tan(α):
tan(θ+α)=5.6x+4x1−(5.6x)(4x)=5.6+4x1−22.4x2=9.6x1−22.4x2
Step 5: Simplify the Expression
This simplifies to:
tan(θ+α)=9.6x⋅x2x2−22.4=9.6xx2−22.4
Step 6: Maximize the Angle
To find the maximum angle, we need to maximize tan(θ+α). We can differentiate:
y=9.6xx2−22.4
Using the quotient rule:
y′=(x2−22.4)(9.6)−9.6x(2x)(x2−22.4)2=9.6(x2−22.4−2x2)(x2−22.4)2=9.6(−x2−22.4)(x2−22.4)2
Setting the numerator to zero for maximization:
−x2−22.4=0⟹x2=22.4⟹x=√22.4=4.73 meters
Step 7: Conclusion
The distance from the column at which the man should stand to see the statue at the greatest angle is approximately:
x≈4.73 meters
We have a statue that is 4 meters high sitting on a column that is 5.6 meters high. Therefore, the total height of the statue from the ground is:
Total height=Height of column+Height of statue=5.6+4=9.6 meters
The man's eye level is 1.6 meters from the ground.
Let x be the distance from the base of the column to the man. The angles we are interested in are:
- α: the angle of elevation from the man's eye level to the top of the statue.
- θ: the angle of elevation from the man's eye level to the top of the column.
Using the definition of tangent for the angles:
- For angle α:
tan(α)=Height of statueDistance from man to column=4x
- For angle θ:
tan(θ)=Height of columnDistance from man to column=5.6x
To find the angle θ+α, we can use the tangent addition formula:
tan(θ+α)=tan(θ)+tan(α)1−tan(θ)tan(α)
Substituting the expressions for tan(θ) and tan(α):
tan(θ+α)=5.6x+4x1−(5.6x)(4x)=5.6+4x1−22.4x2=9.6x1−22.4x2
This simplifies to:
tan(θ+α)=9.6x⋅x2x2−22.4=9.6xx2−22.4
To find the maximum angle, we need to maximize tan(θ+α). We can differentiate:
y=9.6xx2−22.4
Using the quotient rule:
y′=(x2−22.4)(9.6)−9.6x(2x)(x2−22.4)2=9.6(x2−22.4−2x2)(x2−22.4)2=9.6(−x2−22.4)(x2−22.4)2
Setting the numerator to zero for maximization:
−x2−22.4=0⟹x2=22.4⟹x=√22.4=4.73 meters
The distance from the column at which the man should stand to see the statue at the greatest angle is approximately:
x≈4.73 meters
Topper's Solved these Questions
- MAXIMA AND MINIMA Book:MOTIONChapter:MAXIMA AND MINIMA Exercise:EXERCISE -4 (LEVEL - I)
Explore 10 Videos
- MAXIMA AND MINIMA Book:MOTIONChapter:MAXIMA AND MINIMA Exercise:EXERCISE - 4 (LEVEL - II)
Explore 17 Videos
- MAXIMA AND MINIMA Book:MOTIONChapter:MAXIMA AND MINIMA Exercise:EXERCISE - 2 (LEVEL - II)
Explore 15 Videos
- MATRICES Book:MOTIONChapter:MATRICES Exercise:Exercise - 4 (Level-II)
Explore 28 Videos
- METHOD OF DIFFERENTIATIONBook:MOTIONChapter:METHOD OF DIFFERENTIATIONExercise:EXERCISE - 4 LEVEL -II
Explore 5 Videos
Similar Questions
A statue 4 metres high sits on a column 5.6 metres high. How far from the column must a man,whose eye level is 1.6 metres from the ground, stand in order to have the most favourable view of the statue.
View SolutionA statue 8 meters high standing on the top of a tower 64 meters high, on the bank of a river, subtends at a point A on the opposite bank, directly facing the tower, the same angle as subtends at the same point A by man of height 2 meters standing at the base of the tower. Prove that the breadth of the river is 16√6 meters.
View Solution
Knowledge Check
A candle flame 2 cm high is placed at distance of 2 meter from a wall. How far from the wall must a concave mirror to placed in order to form an image of the flame 6 cm high on the wall ?
A225 cmB300cmC450 cmD500 cmA man is moving away from a tower 41.6m high at a rate of 2ms−1. If the eye level of the man is 1.6m above the ground, then the rate at which the angle of elevation of the top of the tower changes, when he is at a distance of 30 m from the foot of the tower, is:
A−4125 radian/sB225 radian/sC−1625 radian/sDNone of theseA pole 25 meters long stands on the top of a tower 225 meters high. It θ is the angle subtended by the pole at a point on the ground which is at distance 2.25 km, from the foot of the tower, then tanθ is equal to
A190B191C110D19
A flag - staff of 10 meters high stands on a building of 50 meters height.For an observer at a height of 60 meters,the flag - staff and the building subtend equal angles. The distance of the observer from the top of the flag - staff is
View SolutionRamesh places a mirror on level ground to determine the height of a pole (with traffic light fired on it) (see the figure). He stands at a certain distance so that he can see the top of the pole reflected from the mirror. Ramesh's eye level is 1.8 m above the ground. The distance of Ramesh and the pole from the mirror are 1.5 m and 2.5 m respectively.
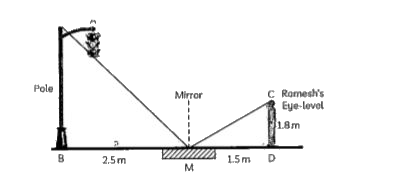
If Ramesh's eye level is 1.2 m above the ground, then the height of the pole is:
View SolutionAnjali places a mirror on level ground to determine the height of a tree (see the diagram) . She stands at a certain distance so that she can see the top of the tree reflected from the mirror . Anjali's eye level is 1.8 m above the ground . The distance of Anjali and the tree from the mirror are 1.5 m and 2.5 m respectively.
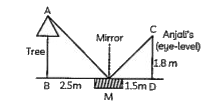
(i) Name the two similar triangles that are formed in the diagram.
ii. State the criterion of similarity that is applicable here.
iii. Find the height of the trees.
View SolutionRamesh places a mirror on level ground to determine the height of a pole (with traffic light fired on it). He stands at a certain distance so that he can see the top of the pole reflected from the mirror. Ramesh's eye level is 1.8 m above the ground. The distance of Ramesh and the pole from the mirror are 1.5 m and 2.5 m respectively.
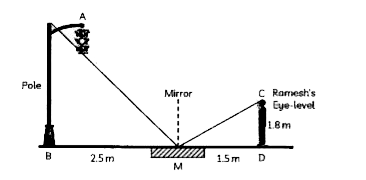
If Ramesh's eye level is 1.2 m above the ground, then the height of the pole is:
View SolutionRamesh places a mirror on level ground to determine the height of a pole (with traffic light fired on it). He stands at a certain distance so that he can see the top of the pole reflected from the mirror. Ramesh's eye level is 1.8 m above the ground. The distance of Ramesh and the pole from the mirror are 1.5 m and 2.5 m respectively.
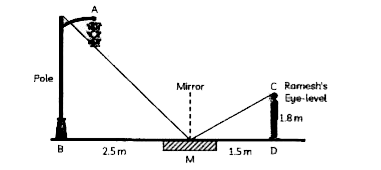
If the distance of Ramesh and the pole from the mirror are 2.5 m and 1.5 m respectively, then the height of the pole is:
View Solution