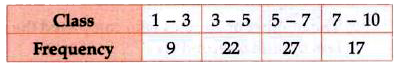
Find the probability distribution of the maximum of two scores obtained when a die is thrown twice. Determine also the mean of the distribution.
To solve the problem of finding the probability distribution of the maximum of two scores obtained when a die is thrown twice, and to determine the mean of that distribution, we can follow these steps:
Step 1: Identify Possible Outcomes
When a die is thrown twice, the possible outcomes for each die are 1, 2, 3, 4, 5, and 6. Therefore, the total number of outcomes when throwing two dice is 6×6=36.
Step 2: Determine the Maximum Values
We need to find the probability distribution of the maximum score, which can take values from 1 to 6. We will calculate the probability for each maximum value.
Step 3: Calculate Probabilities for Each Maximum Value
1. Maximum = 1:
- The only way to achieve a maximum of 1 is if both dice show 1.
- Favorable outcomes: (1,1) → 1 outcome.
- Probability: P(X=1)=136.
2. Maximum = 2:
- The maximum can be 2 if both dice show either 1 or 2.
- Favorable outcomes: (1,1), (1,2), (2,1), (2,2) → 3 outcomes.
- Probability: P(X=2)=336=112.
3. Maximum = 3:
- The maximum can be 3 if at least one die shows 3 and none show higher.
- Favorable outcomes: (1,1), (1,2), (1,3), (2,1), (2,2), (2,3), (3,1), (3,2), (3,3) → 5 outcomes.
- Probability: P(X=3)=536.
4. Maximum = 4:
- The maximum can be 4 if at least one die shows 4 and none show higher.
- Favorable outcomes: (1,1), (1,2), (1,3), (1,4), (2,1), (2,2), (2,3), (2,4), (3,1), (3,2), (3,3), (3,4), (4,1), (4,2), (4,3), (4,4) → 7 outcomes.
- Probability: P(X=4)=736.
5. Maximum = 5:
- The maximum can be 5 if at least one die shows 5 and none show higher.
- Favorable outcomes: (1,1), (1,2), (1,3), (1,4), (1,5), (2,1), (2,2), (2,3), (2,4), (2,5), (3,1), (3,2), (3,3), (3,4), (3,5), (4,1), (4,2), (4,3), (4,4), (4,5), (5,1), (5,2), (5,3), (5,4), (5,5) → 9 outcomes.
- Probability: P(X=5)=936=14.
6. Maximum = 6:
- The maximum can be 6 if at least one die shows 6.
- Favorable outcomes: (1,1), (1,2), (1,3), (1,4), (1,5), (1,6), (2,1), (2,2), (2,3), (2,4), (2,5), (2,6), (3,1), (3,2), (3,3), (3,4), (3,5), (3,6), (4,1), (4,2), (4,3), (4,4), (4,5), (4,6), (5,1), (5,2), (5,3), (5,4), (5,5), (5,6), (6,1), (6,2), (6,3), (6,4), (6,5), (6,6) → 11 outcomes.
- Probability: P(X=6)=1136.
Step 4: Summarize the Probability Distribution
The probability distribution of the maximum score X is as follows:
| X | Probability P(X) |
|-------|------------------------|
| 1 | 136 |
| 2 | 336 |
| 3 | 536 |
| 4 | 736 |
| 5 | 936 |
| 6 | 1136 |
Step 5: Calculate the Mean of the Distribution
To find the mean E(X), we use the formula:
E(X)=∑(x⋅P(X))
Calculating each term:
E(X)=1⋅136+2⋅336+3⋅536+4⋅736+5⋅936+6⋅1136
Calculating each product:
- 1⋅136=136
- 2⋅336=636
- 3⋅536=1536
- 4⋅736=2836
- 5⋅936=4536
- 6⋅1136=6636
Now summing these values:
E(X)=1+6+15+28+45+6636=16136
Final Result
Thus, the mean of the distribution is:
E(X)=16136
When a die is thrown twice, the possible outcomes for each die are 1, 2, 3, 4, 5, and 6. Therefore, the total number of outcomes when throwing two dice is 6×6=36.
We need to find the probability distribution of the maximum score, which can take values from 1 to 6. We will calculate the probability for each maximum value.
- The only way to achieve a maximum of 1 is if both dice show 1.
- Favorable outcomes: (1,1) → 1 outcome.
- Probability: P(X=1)=136.
- The maximum can be 2 if both dice show either 1 or 2.
- Favorable outcomes: (1,1), (1,2), (2,1), (2,2) → 3 outcomes.
- Probability: P(X=2)=336=112.
- The maximum can be 3 if at least one die shows 3 and none show higher.
- Favorable outcomes: (1,1), (1,2), (1,3), (2,1), (2,2), (2,3), (3,1), (3,2), (3,3) → 5 outcomes.
- Probability: P(X=3)=536.
- The maximum can be 4 if at least one die shows 4 and none show higher.
- Favorable outcomes: (1,1), (1,2), (1,3), (1,4), (2,1), (2,2), (2,3), (2,4), (3,1), (3,2), (3,3), (3,4), (4,1), (4,2), (4,3), (4,4) → 7 outcomes.
- Probability: P(X=4)=736.
- The maximum can be 5 if at least one die shows 5 and none show higher.
- Favorable outcomes: (1,1), (1,2), (1,3), (1,4), (1,5), (2,1), (2,2), (2,3), (2,4), (2,5), (3,1), (3,2), (3,3), (3,4), (3,5), (4,1), (4,2), (4,3), (4,4), (4,5), (5,1), (5,2), (5,3), (5,4), (5,5) → 9 outcomes.
- Probability: P(X=5)=936=14.
- The maximum can be 6 if at least one die shows 6.
- Favorable outcomes: (1,1), (1,2), (1,3), (1,4), (1,5), (1,6), (2,1), (2,2), (2,3), (2,4), (2,5), (2,6), (3,1), (3,2), (3,3), (3,4), (3,5), (3,6), (4,1), (4,2), (4,3), (4,4), (4,5), (4,6), (5,1), (5,2), (5,3), (5,4), (5,5), (5,6), (6,1), (6,2), (6,3), (6,4), (6,5), (6,6) → 11 outcomes.
- Probability: P(X=6)=1136.
The probability distribution of the maximum score X is as follows:
|-------|------------------------|
| 1 | 136 |
| 2 | 336 |
| 3 | 536 |
| 4 | 736 |
| 5 | 936 |
| 6 | 1136 |
To find the mean E(X), we use the formula:
- 2⋅336=636
- 3⋅536=1536
- 4⋅736=2836
- 5⋅936=4536
- 6⋅1136=6636
Thus, the mean of the distribution is:
Topper's Solved these Questions
Similar Questions
Knowledge Check
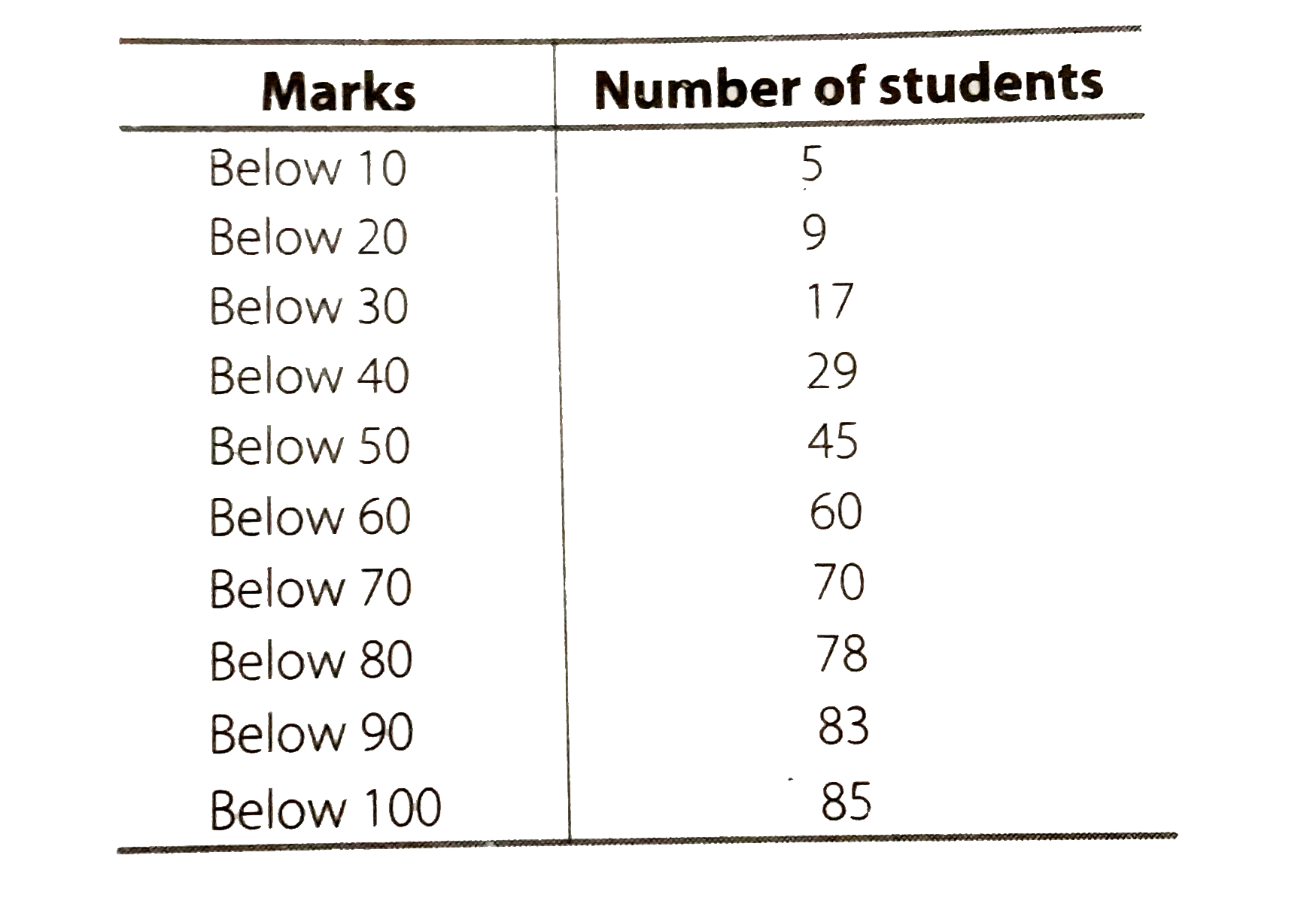
Obtain the mean of the following distribution.
 A7.5B8.05C9DNone
A7.5B8.05C9DNone
Determine the mean of the following distribution:
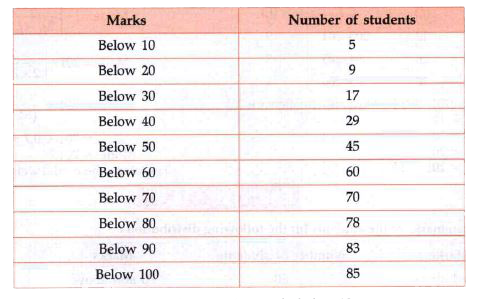
View SolutionFind the mean of the following distribution.

View SolutionFind the mean of the following distribution.

View SolutionFind the probability distribution of the number of sixes in three tosses of a die.
View SolutionFind the mean of following distribution
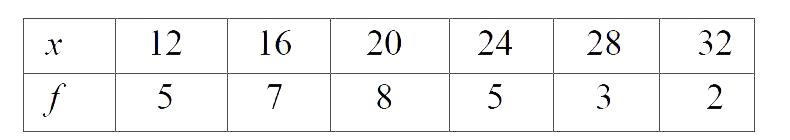
View SolutionFind the mean of the following distribution
View SolutionFind the mean of the following distribution

View Solution