If the whole earth is to be connected by LOS communication using space waves ( no restriction of antenna size or tower height), what is the minimum no of antennaas required ? Calculate the tower height of these antennas in terms of earth's radius?

Video Solution

Text Solution

Verified by Experts

Consider the figure given below to solve this question

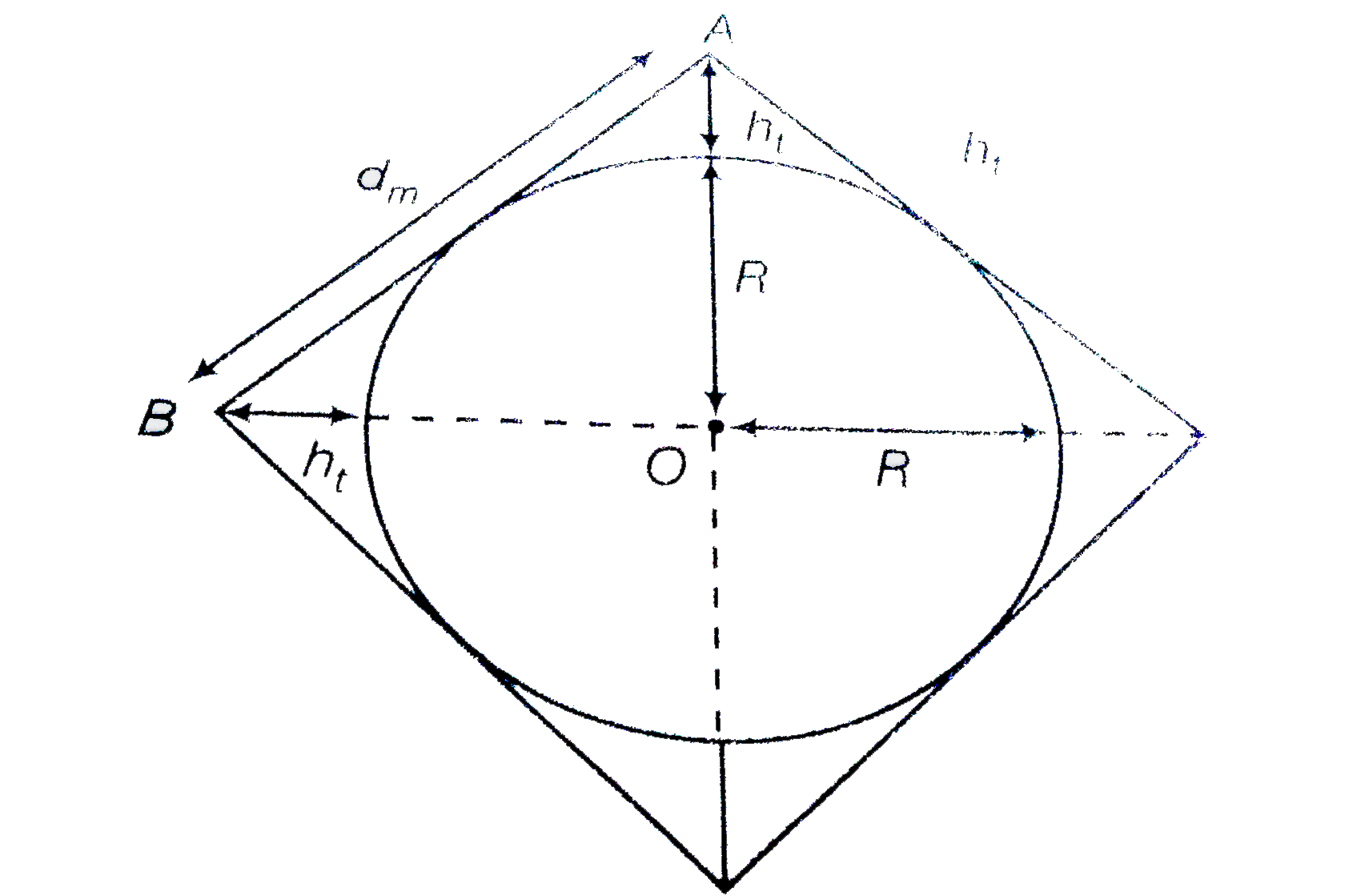
Suppose the height of tansmitting antenna or receiving antenna in order to cover the entire surface of earth through communication is $h_{t}$ and radius of earth is R
Then, maximum distance
$d_{m}^{2} = {(R + h_{t})}^{2} + {(R + h_{t})}^{2}$
$= 2 {(R + h_{t})}^{2}$
$d_{m} = \sqrt{2 h_{t} R} + \sqrt{2 h_{t} R} = 2 \sqrt{2 h_{t} R}$
$∴ 8 h_{t} R = 2 {(R + h_{t})}^{2}$
$\Rightarrow 4 h_{t} R = R^{2} + 2 R h_{t} + h_{t}^{2}$
$\Rightarrow R^{2} - 2 h_{t} R + h_{t}^{2} = 0$
$\Rightarrow {(R - h_{t})}^{2} = 0$
$\Rightarrow R = h_{t}$
Since, space wave frequency is used so $λ < < h_{t}$ , hence only tower height is to be taken into consideration. In three dimensions of earth, 6 antenna towers of each of height $h_{t} = R$ would be used to cover the entire surface of earth with communication programme.

Updated on:21/07/2023

The heights of two antennas above the earth are $h_{T}$ and $h_{R}$ respectively. The maximum line of sight distance $d_{M}$ between the two antennas is (R→Radius of earth)
A $d_{m} = \sqrt{2} R h_{T} - \sqrt{2} R h_{R}$
B $d_{m} = {(\sqrt{2} R h_{T} - \sqrt{2} R h_{R})}_{T}^{2}$
C $d_{m} = \sqrt{2} R h_{T} + \sqrt{2} R h_{R}$
D $d_{m} = {(\sqrt{2} R h_{T} + \sqrt{2} R h_{R})}_{T}^{2}$
The radius of the Earth is 6400 km. If the height of an antenna is 500 m, then its range is
A800 km
B100 km
C80 km
D10 km
In a line of sight radio communication, a distance of about $50$ is Kept between the transmitting and receiving entennas. If the height of the receiving antenna is $70 m$ , then the minimum height fof the transmitting antenna should be:
$(Radius of the Earth = 6.4 \times 10^{6} m)$ .
A $20 m$
B $51 m$
C $32 m$
D $40 m$