Given that the displacement of a particle , where 't' denotes the time. The dimension of K is same as that of :
hertz
metre
radian
second
The correct Answer is:A
To find the dimension of K in the equation x=A2sin2(Kt), we need to ensure that the argument of the sine function, Kt, is dimensionless. Let's break this down step by step.
Step 1: Understand the Argument of the Sine Function
The sine function takes an angle as its argument, and angles are dimensionless quantities. Therefore, for Kt to be dimensionless, the product must not have any units.
Step 2: Analyze the Dimensions
Let’s denote the dimensions of x (displacement) and A (a constant) as follows:
- The dimension of displacement x is (L) (length).
- The dimension of A2 will also be (L) since it is a constant related to displacement.
Step 3: Set Up the Equation
Since Kt must be dimensionless, we can express this as:
(K)⋅(T)=1
where (T) is the dimension of time.
Step 4: Solve for the Dimension of K
Rearranging the equation gives us:
(K)=1(T)
This means that the dimension of K is (T)−1.
Step 5: Identify the Unit of K
The dimension (T)−1 corresponds to frequency, which is measured in Hertz (Hz).
Conclusion
Thus, the dimension of K is (T)−1, which is equivalent to the unit of frequency.
Final Answer
The dimension of K is (T)−1, or Hertz (Hz).
---
The sine function takes an angle as its argument, and angles are dimensionless quantities. Therefore, for Kt to be dimensionless, the product must not have any units.
Let’s denote the dimensions of x (displacement) and A (a constant) as follows:
- The dimension of displacement x is (L) (length).
- The dimension of A2 will also be (L) since it is a constant related to displacement.
Since Kt must be dimensionless, we can express this as:
(K)⋅(T)=1
where (T) is the dimension of time.
Rearranging the equation gives us:
(K)=1(T)
This means that the dimension of K is (T)−1.
The dimension (T)−1 corresponds to frequency, which is measured in Hertz (Hz).
Thus, the dimension of K is (T)−1, which is equivalent to the unit of frequency.
The dimension of K is (T)−1, or Hertz (Hz).
Similar Questions
The displacement of a progressive wave is represented by y=Asin(ωt−kx) where x is distance and t is time.The dimensions of ωk are same as those of (A) velocity (B) wave number ?
View SolutionGiven that the displacement of an oscillating particle is given by y=A sin(Bx+Ct+D)" where "x" is position and "t" is time.Find the dimensional formula for (ABCD)
View Solution
Knowledge Check
When a wave transverses in a medium, the displacement of a particle located at distance x at time t is given by y=asin(bt−cx) where a, b and c are constants of the wave. The dimension of b/c are same as that of:
AWave velocityBWavelengthCWave amplitudeDWave frequencyThe displacement of a progressive wave is represented by y=Asin(ωt−kx) where x is distance and t is time. The dimensions of ωk are same as those of
AvelocityBwave numberCwavelengthDfrequencyA force F is given by F=at+bt2 , where t is time . What are the dimensions of aandb?
A[MLT−4]and[MLT]B[MLT−1]and[MLT0]C[MLT−3]and[MLT−4]D[MLT−3]and[MLT0]
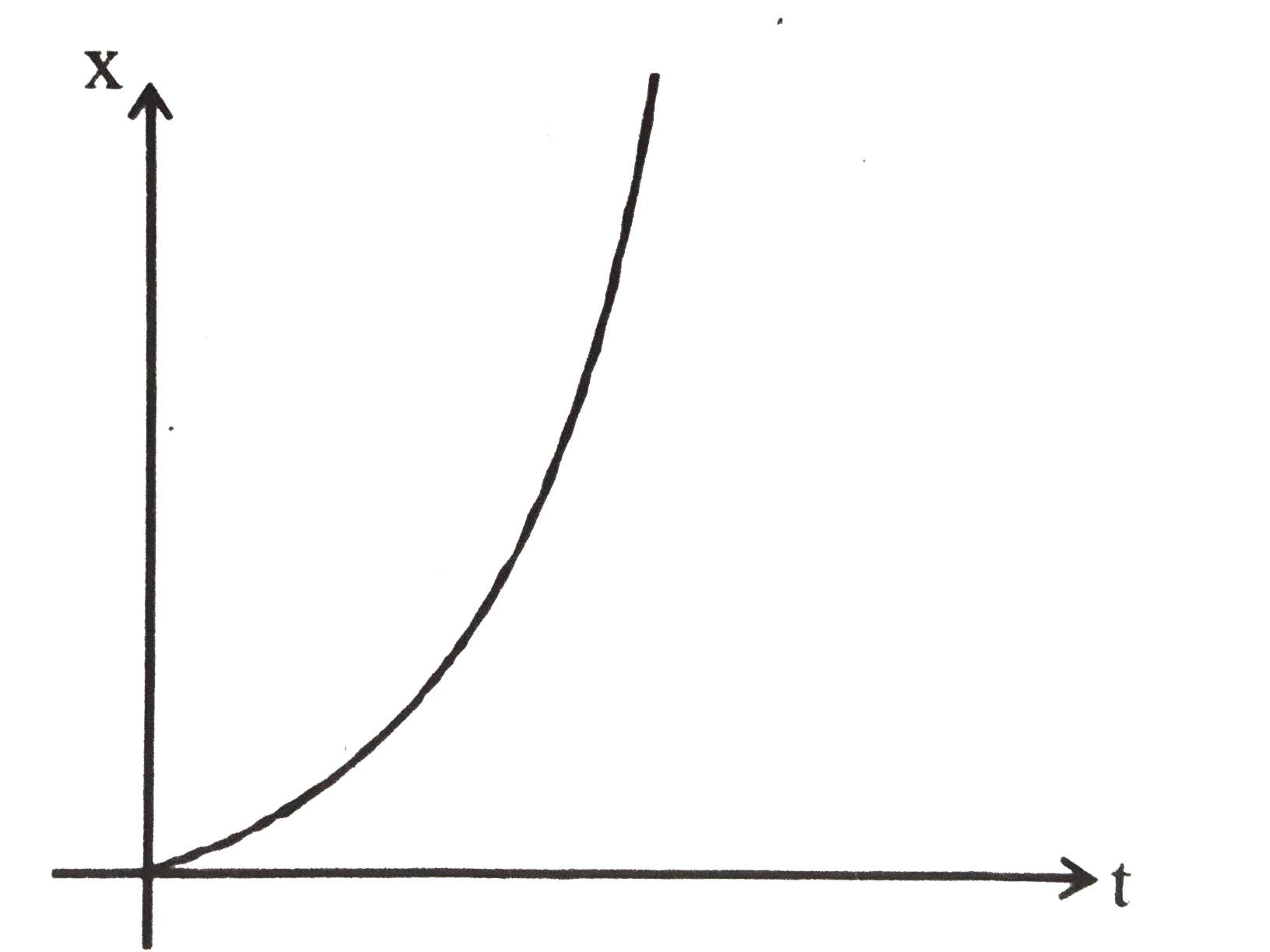
The plot given above represents displacement 'x' of a particle with time 't'. The particle is
View SolutionThe displacement of a particle is moving by x=(t−2)2 where x is in metres and t in second. The distance covered by the particle in first 4 seconds is.
View SolutionThe displacement of a particle in time t is given by s=2t2−3t+1. The acceleration is
View SolutionThe displacement of a particle is given by x=3sin(5πt)+4cos(5πt). The amplitude of particle is
View SolutionThe displacement of a particle is moving by x=(t−2)2 where x is in metres and t in second. The distance covered by the particle in first 4 seconds is.
View Solution