Two masses m and m' are tied with a thread passing over a pulley, m' is on a frictionless horizontal surface and m is hanging freely. If acceleration due to gravity is g, the acceleration of m' in this arrangment will be :
g
mg/(m+m')
mg/m'
mg/(m−m')
The correct Answer is:2
To solve the problem, we need to analyze the forces acting on both masses and apply Newton's second law of motion. Here's a step-by-step solution:
Step 1: Identify the system
We have two masses:
- Mass m′ is on a frictionless horizontal surface.
- Mass m is hanging freely.
Step 2: Draw Free Body Diagrams (FBD)
1. For the hanging mass m:
- The force acting downward is the weight mg.
- The force acting upward is the tension T in the thread.
The net force acting on mass m is:
Fnet=mg−T
2. For the mass m′ on the surface:
- The only horizontal force acting on it is the tension T.
The net force acting on mass m′ is:
Fnet=T
Step 3: Apply Newton's Second Law
Using Newton's second law, we can write the equations for both masses:
1. For mass m:
mg−T=ma(Equation 1)
2. For mass m′:
T=m′a(Equation 2)
Step 4: Solve the equations
From Equation 2, we can express tension T in terms of m′ and a:
T=m′a
Substituting this expression for T into Equation 1:
mg−m′a=ma
Rearranging gives:
mg=ma+m′a
Factoring out a:
mg=(m+m′)a
Step 5: Solve for acceleration a
Now, we can solve for a:
a=mgm+m′
Conclusion
The acceleration of mass m′ on the frictionless surface is:
a=mgm+m′
We have two masses:
- Mass m′ is on a frictionless horizontal surface.
- Mass m is hanging freely.
1. For the hanging mass m:
- The force acting downward is the weight mg.
- The force acting upward is the tension T in the thread.
The net force acting on mass m is:
Fnet=mg−T
- The only horizontal force acting on it is the tension T.
The net force acting on mass m′ is:
Fnet=T
Using Newton's second law, we can write the equations for both masses:
mg−T=ma(Equation 1)
T=m′a(Equation 2)
From Equation 2, we can express tension T in terms of m′ and a:
T=m′a
mg−m′a=ma
mg=ma+m′a
mg=(m+m′)a
Now, we can solve for a:
a=mgm+m′
The acceleration of mass m′ on the frictionless surface is:
a=mgm+m′
Similar Questions
Knowledge Check
In the figure given below masses m and m' are tied with a thread passing over a pulley, m' is on a frictionlesss horizontal surface. If acceleration due to gravity is g, the acceleration of m' in this arrangement will be
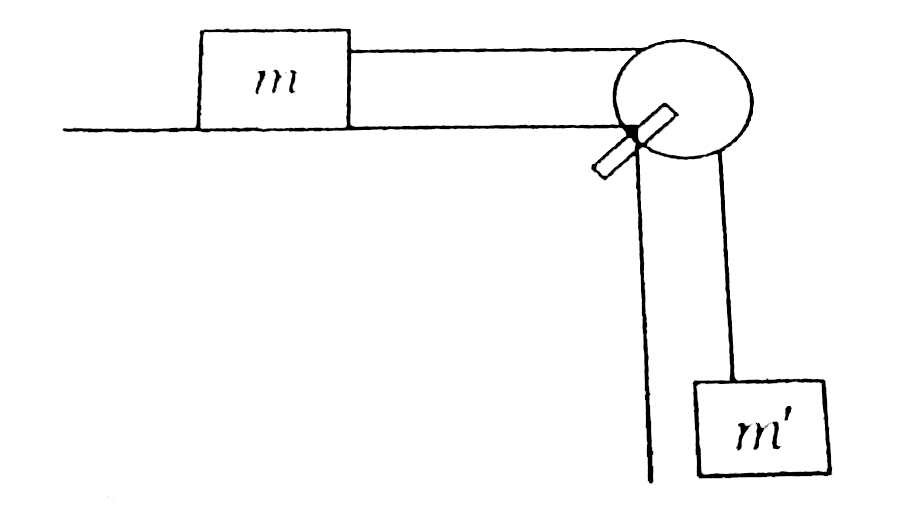 AgBm'g(m+m')Cmgm'Dmg(m−m')
AgBm'g(m+m')Cmgm'Dmg(m−m')In the fig. given masses m and m' are tied with a tread passing over a pulley, m' is on a frictionless horizontal surface. If acceleration due to gravity is g, the acceleration of m' in this arrangement will be:-
 AgBm'g/(m+m)Cmg/m'Dmg/(m-m)
AgBm'g/(m+m)Cmg/m'Dmg/(m-m)A small magnet M is allowed to fall through a fixed horizontal conducting ring R. Let g be the acceleration due to gravity. The acceleration of M will be
A(a) <g when it is above R and moving toward RB(b) >g when it is above R and moving toward RC( c) <g when it is below R and moving away from RD( d) >g when it is below R and moving away from R
Two masses m and M(m<M) are joined by a light string passing over a smooth and light pulley (as shown)

View SolutionTwo masses M and m(M>m) are joined by a light string passing over a smooth light pulley.

View SolutionTwo masses m and M(>m) are joined by a light string passing over a smooth light pulley. The centre of mass of the system moves with an acceleration

View SolutionTwo blocks of masses M and m are connected with massless and frictionless pulleys as shown. Strings are also massless. If the magnitude of acceleration of M is a0 then magnitude of acceleration of m is
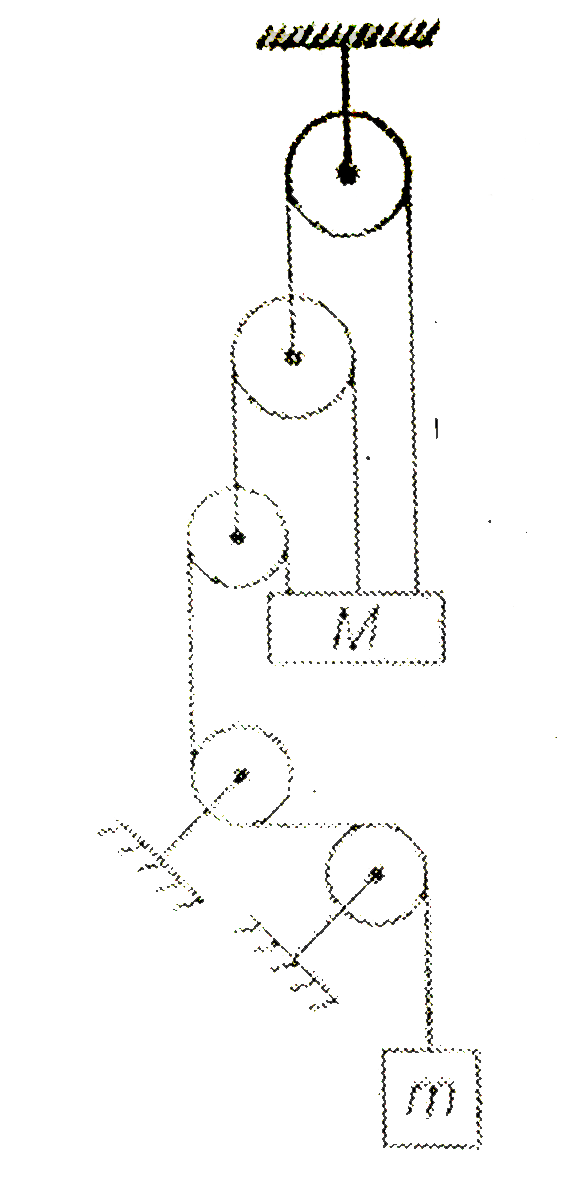
View SolutionIf ge is acceleration due to gravity on earth and gm is acceleration due to gravity on moon, then
View Solution