A pendulum is hanging from the ceiling of a railway carriage making an angle of 30∘ with the vertical direction . The acceleration of the carriage is :
g√3
2g√3
g√3
√3g2
The correct Answer is:1
To solve the problem, we will analyze the forces acting on the pendulum bob and apply the concepts of equilibrium in a non-inertial frame (the accelerating carriage).
1. Identify the Forces Acting on the Pendulum Bob:
- The forces acting on the pendulum bob are:
- The gravitational force (weight) acting downward, mg.
- The tension T in the string acting along the string.
- A pseudo-force Fpseudo acting horizontally in the opposite direction of the acceleration of the carriage, which has a magnitude of ma, where a is the acceleration of the carriage.
2. Set Up the Coordinate System:
- Let’s consider the vertical direction as downward and the horizontal direction as the direction of the carriage's acceleration. The pendulum makes an angle of 30∘ with the vertical.
3. Resolve the Tension into Components:
- The tension T can be resolved into two components:
- Vertical component: Tcos(30∘)
- Horizontal component: Tsin(30∘)
4. Apply Equilibrium Conditions:
- In the vertical direction, the forces must balance:
Tcos(30∘)=mg(1)
- In the horizontal direction, the net force is equal to the pseudo-force:
Tsin(30∘)=ma(2)
5. Divide Equation (2) by Equation (1):
- By dividing the two equations, we eliminate T:
Tsin(30∘)Tcos(30∘)=mamg
- This simplifies to:
tan(30∘)=ag
6. Solve for the Acceleration a:
- We know that tan(30∘)=1√3:
1√3=ag
- Rearranging gives:
a=g⋅1√3=g√3
Final Answer:
The acceleration of the carriage is:
a=g√3
- The forces acting on the pendulum bob are:
- The gravitational force (weight) acting downward, mg.
- The tension T in the string acting along the string.
- A pseudo-force Fpseudo acting horizontally in the opposite direction of the acceleration of the carriage, which has a magnitude of ma, where a is the acceleration of the carriage.
- Let’s consider the vertical direction as downward and the horizontal direction as the direction of the carriage's acceleration. The pendulum makes an angle of 30∘ with the vertical.
- The tension T can be resolved into two components:
- Vertical component: Tcos(30∘)
- Horizontal component: Tsin(30∘)
- In the vertical direction, the forces must balance:
Tcos(30∘)=mg(1)
- In the horizontal direction, the net force is equal to the pseudo-force:
Tsin(30∘)=ma(2)
- By dividing the two equations, we eliminate T:
Tsin(30∘)Tcos(30∘)=mamg
- This simplifies to:
tan(30∘)=ag
- We know that tan(30∘)=1√3:
1√3=ag
- Rearranging gives:
a=g⋅1√3=g√3
The acceleration of the carriage is:
a=g√3
Similar Questions
A car moving on a level rosd. A pendulum suspended from the ceiling makes an angle of 10∘ with the vertical. Find the acceleration of the car. Take g=10ms−2.
View SolutionA pendulum is hanging from the ceiling of a car having an acceleration a0 with to the road. Find the angle made by the string with the verical and tension in the string.
View Solution
Knowledge Check
The bob of a 0.2 m pendulum describes an arc of circle in a vertical plane. If the tension in the cord is √3 times the weight of the bob when the cord makes an angle 30∘ with the vertical, the acceleration of the bob in that position is
AgBg2C√3g2Dg4A bob of mass m=50g is suspended form the ceiling of a trolley by a light inextensible string. If the trolley accelerates horizontally, the string makes an angle tˆa=37∘ with the vertical. Find the acceleration of the trolley.
 A10m/s2B12.5m/s2C7.5m/s2D7.5m/s
A10m/s2B12.5m/s2C7.5m/s2D7.5m/s
A pendulum is hanging from the ceiling of a car having an acceleration a0 with respect to the road. Findthe angle made by the string with the vertical.
View SolutionA train is moving along a horizontal track . A pendulum suspended from the roof makes an angle of 4∘ with the vertical . If g=10m/s2 , what is the acceleration of the train ?
View SolutionA steel ball is suspended from the ceiling of an accelerating carriage by means of cords A and B. Determine the acceleration a of the carriage which cause the tension in A to be twice that in B.
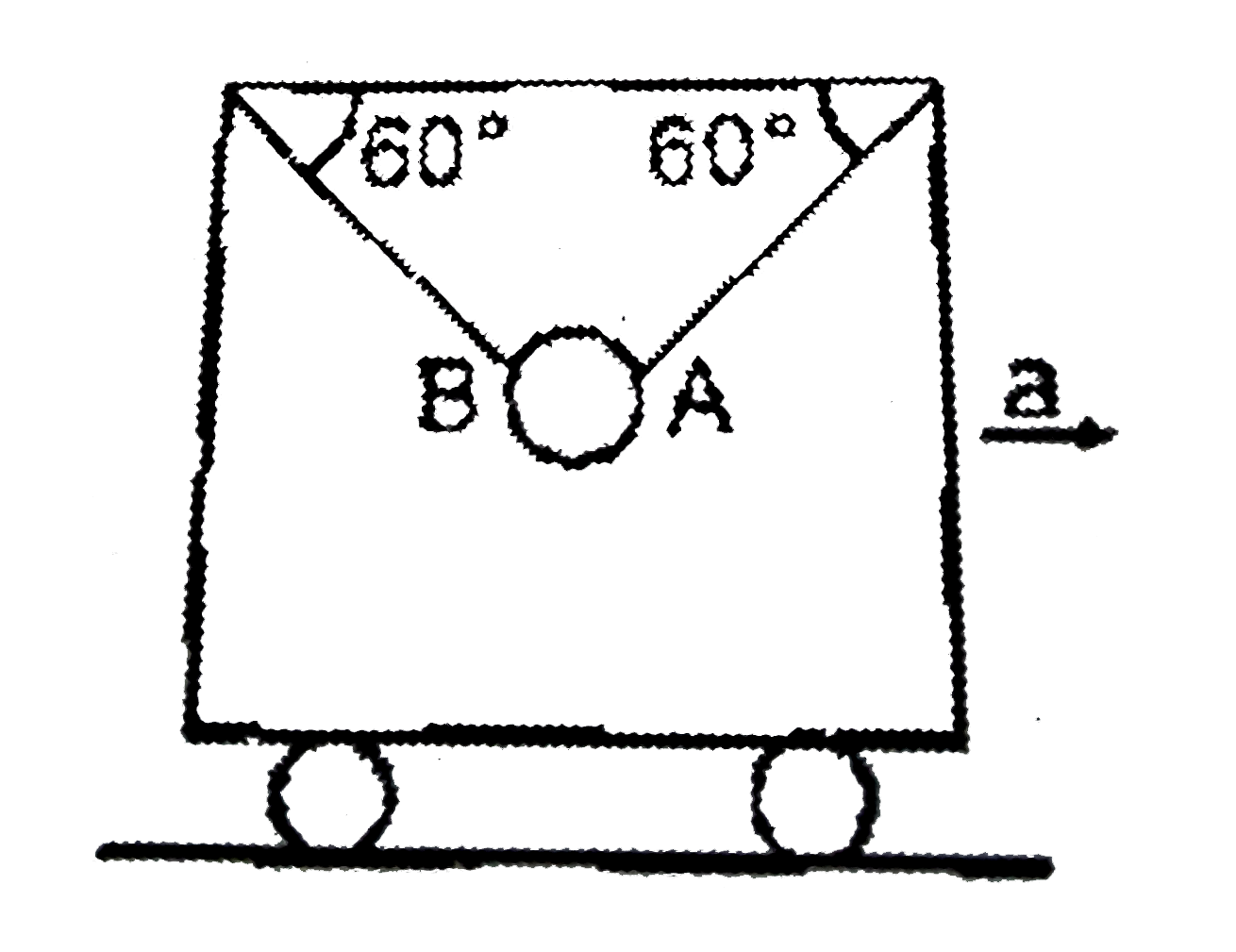
View SolutionA steel ball is suspended from the ceiling of an acceleration carriage by means of two cords A and B. Determine the acceleration a of the carriage which will cause the tension in A to be twice that in B.

View SolutionA pendulum is hanging from the ceiling of a car having an acceleration a0 with respect to the road. Findthe angle made by the string with the vertical.
View SolutionA pedulum is hanging from the ceiling of a car having an acceleration a0 with respect to the road Find the angle made by the string with vertical at equilibrium Also find the tension in the string in this position
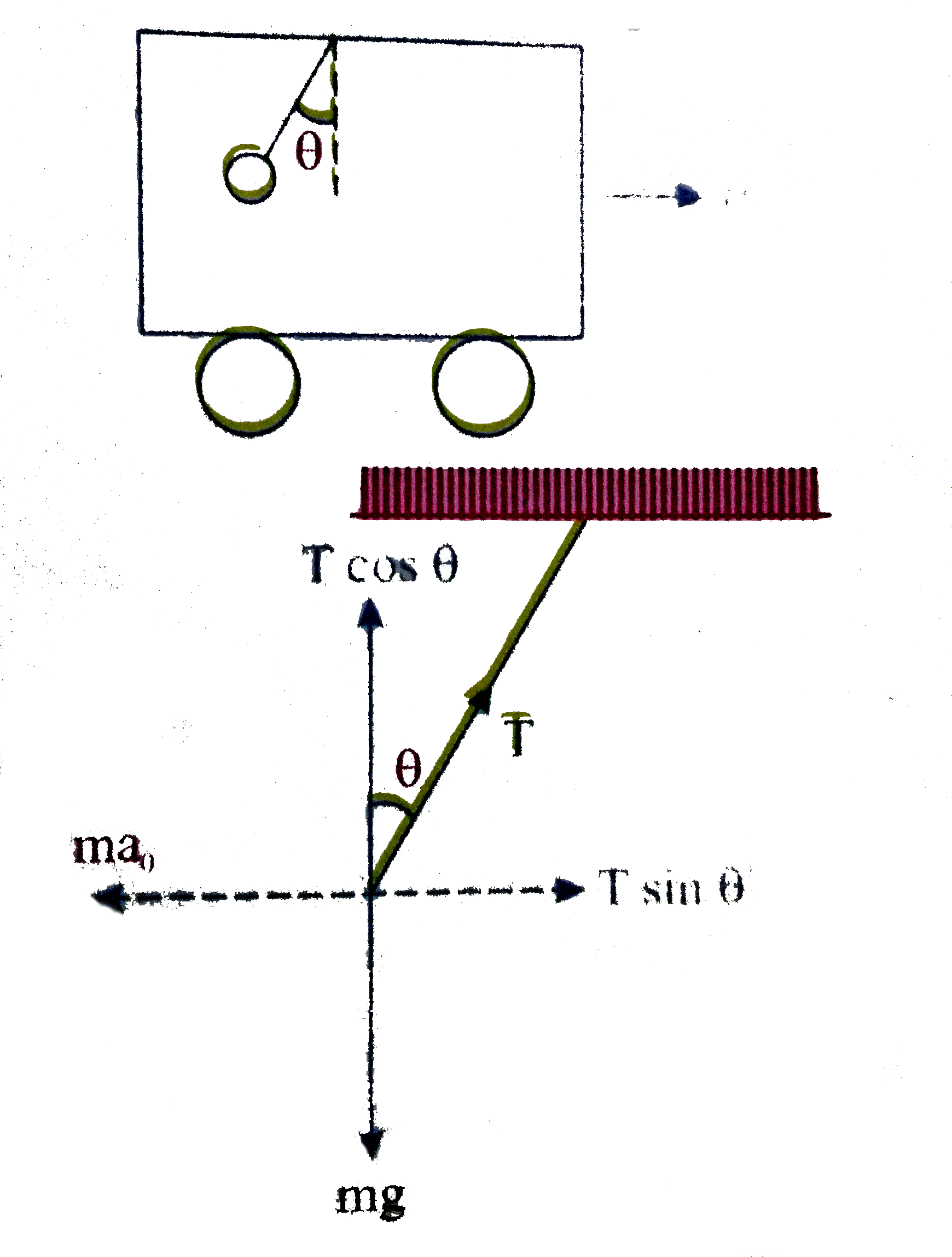 .
.
View Solution
