ϕ is the angle of the incline when a block of mass m just starts slipping down. The distance covered by the block if thrown up the incline with an initial speed v0 is :
v204gsinϕ
4v20gsinϕ
v20sinϕ4g
4v20sinϕg
The correct Answer is:1
To solve the problem of how far a block of mass m will travel up an incline when thrown with an initial speed v0 before it starts slipping back down, we can follow these steps:
1. Identify Forces Acting on the Block:
- The gravitational force acting on the block can be resolved into two components:
- Perpendicular to the incline: mgcosϕ
- Parallel to the incline (down the slope): mgsinϕ
2. Determine the Frictional Force:
- The frictional force (f) acting on the block when it is moving up the incline is given by:
f=μmgcosϕ
- Here, μ is the coefficient of friction.
3. Calculate the Net Force:
- The net force (Fnet) acting on the block when it is thrown up the incline can be expressed as:
Fnet=−mgsinϕ−μmgcosϕ
- The negative sign indicates that both the gravitational force and the frictional force act in the direction opposite to the motion.
4. Determine the Acceleration:
- Using Newton's second law, we can express the acceleration (a) of the block:
ma=−mgsinϕ−μmgcosϕ
- Dividing through by m:
a=−gsinϕ−μgcosϕ
5. Use Kinematic Equation:
- We can use the kinematic equation to find the distance s the block travels up the incline before coming to rest:
v2f=v20+2as
- Here, vf=0 (final velocity when the block stops), v0 is the initial velocity, and a is the acceleration we found earlier.
- Plugging in the values:
0=v20+2(−gsinϕ−μgcosϕ)s
- Rearranging gives:
v20=2(gsinϕ+μgcosϕ)s
6. Solve for Distance s:
- Finally, we can solve for s:
s=v202(gsinϕ+μgcosϕ)
Final Answer:
The distance covered by the block when thrown up the incline with an initial speed v0 is:
s=v202(gsinϕ+μgcosϕ)
- The gravitational force acting on the block can be resolved into two components:
- Perpendicular to the incline: mgcosϕ
- Parallel to the incline (down the slope): mgsinϕ
- The frictional force (f) acting on the block when it is moving up the incline is given by:
f=μmgcosϕ
- Here, μ is the coefficient of friction.
- The net force (Fnet) acting on the block when it is thrown up the incline can be expressed as:
Fnet=−mgsinϕ−μmgcosϕ
- The negative sign indicates that both the gravitational force and the frictional force act in the direction opposite to the motion.
- Using Newton's second law, we can express the acceleration (a) of the block:
ma=−mgsinϕ−μmgcosϕ
- Dividing through by m:
a=−gsinϕ−μgcosϕ
- We can use the kinematic equation to find the distance s the block travels up the incline before coming to rest:
v2f=v20+2as
- Here, vf=0 (final velocity when the block stops), v0 is the initial velocity, and a is the acceleration we found earlier.
- Plugging in the values:
0=v20+2(−gsinϕ−μgcosϕ)s
- Rearranging gives:
v20=2(gsinϕ+μgcosϕ)s
- Finally, we can solve for s:
s=v202(gsinϕ+μgcosϕ)
The distance covered by the block when thrown up the incline with an initial speed v0 is:
s=v202(gsinϕ+μgcosϕ)
Similar Questions
The maximum range of a projectile is 500m. If the particle is thrown up a plane is inclined at an angle of 30∘ with the same speed, the distance (in meter) covered by it along the inclined plane will be:
View SolutionFigure shows a spring fixed at the bottom end of an incline of inclination 370. A small block of mass 2 kg starts slipping down the incline from a point 4.8 m away from the spring. The block compresses the spring by 20 cm, stops momentarily and then rebounds through a distance of 1 m up the incline. Find a. the frictioin coefficient between the plane and the block and b. the spring constant of the spring. Take g=10ms2.
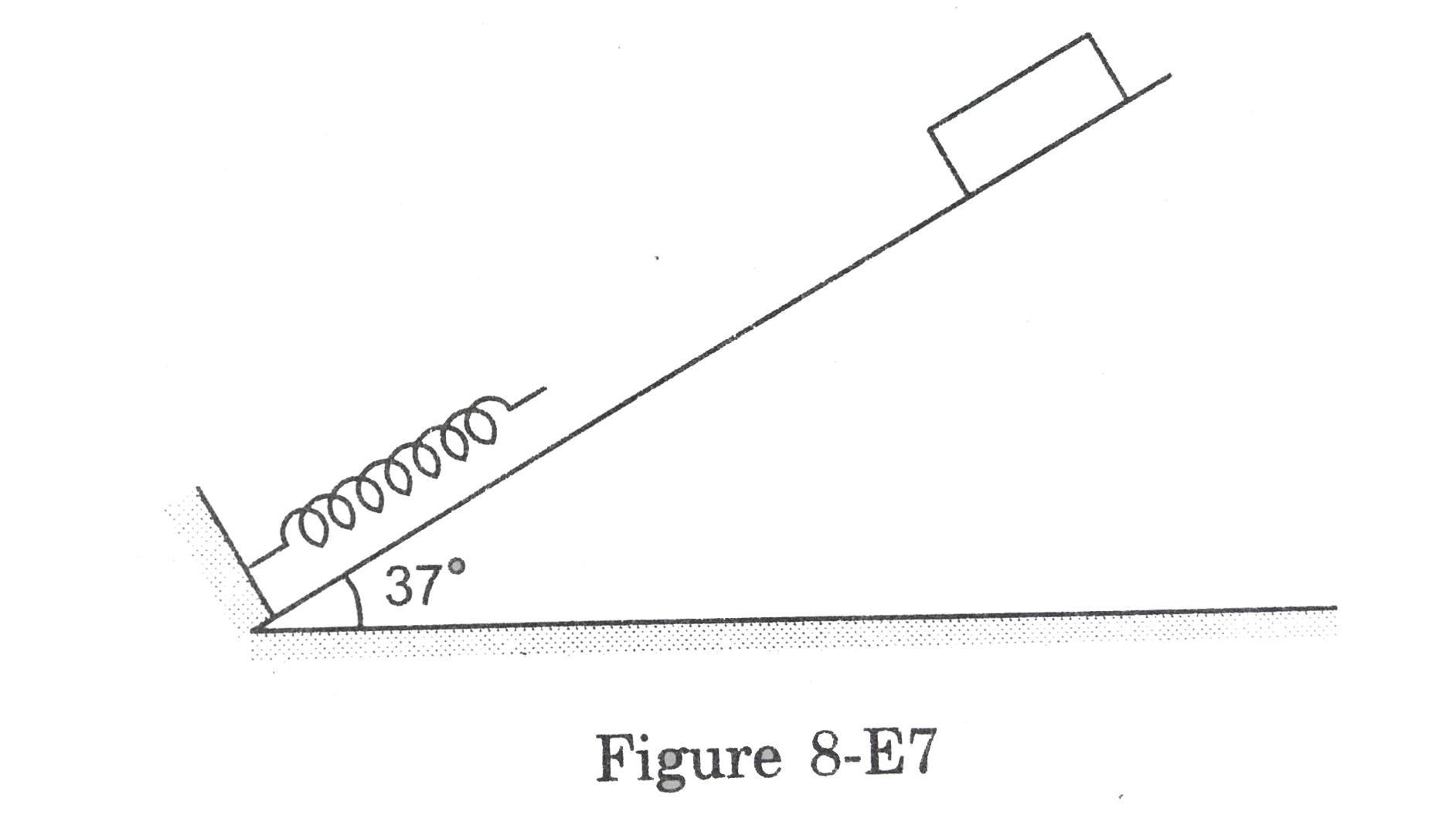
View Solution
Knowledge Check
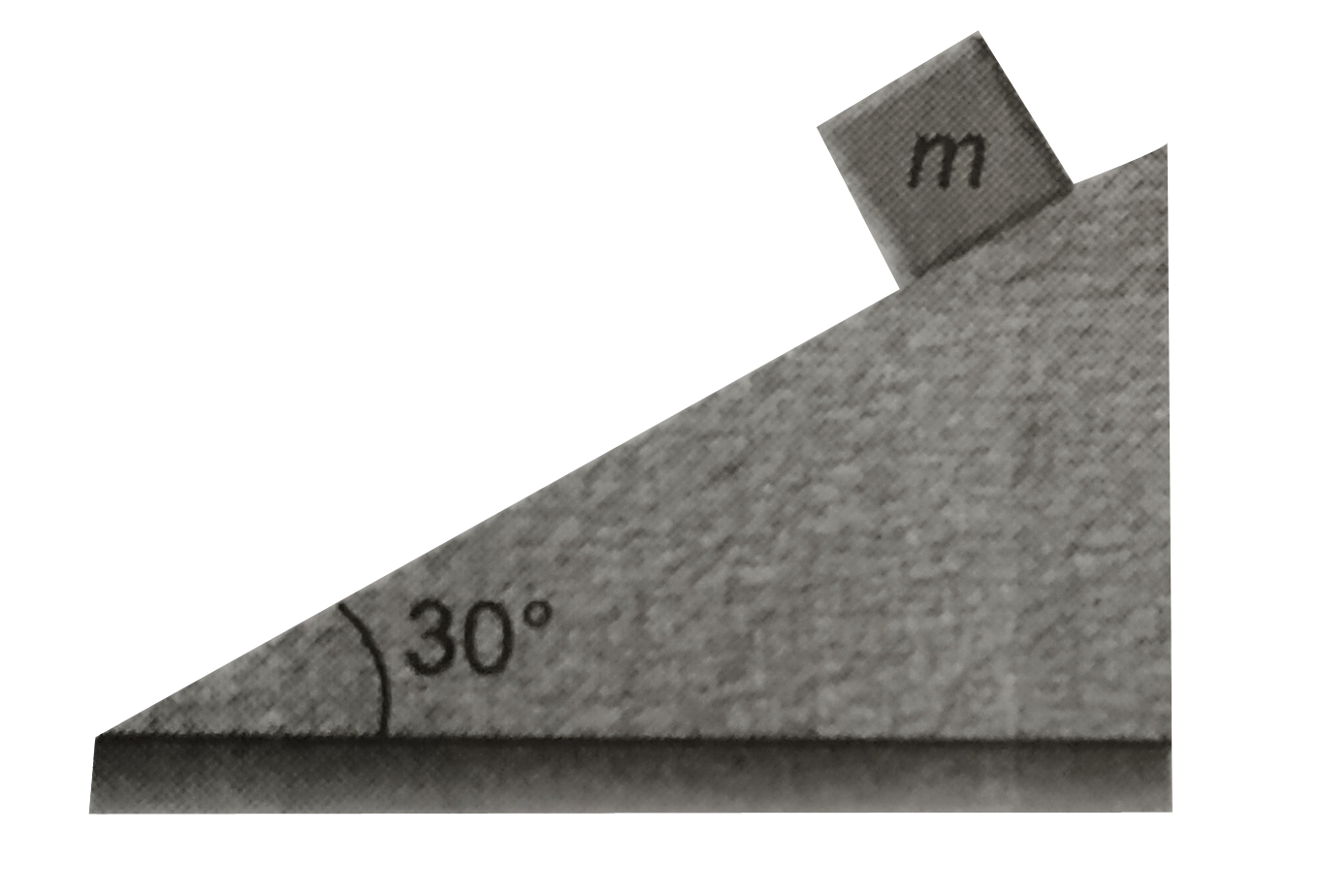
A man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
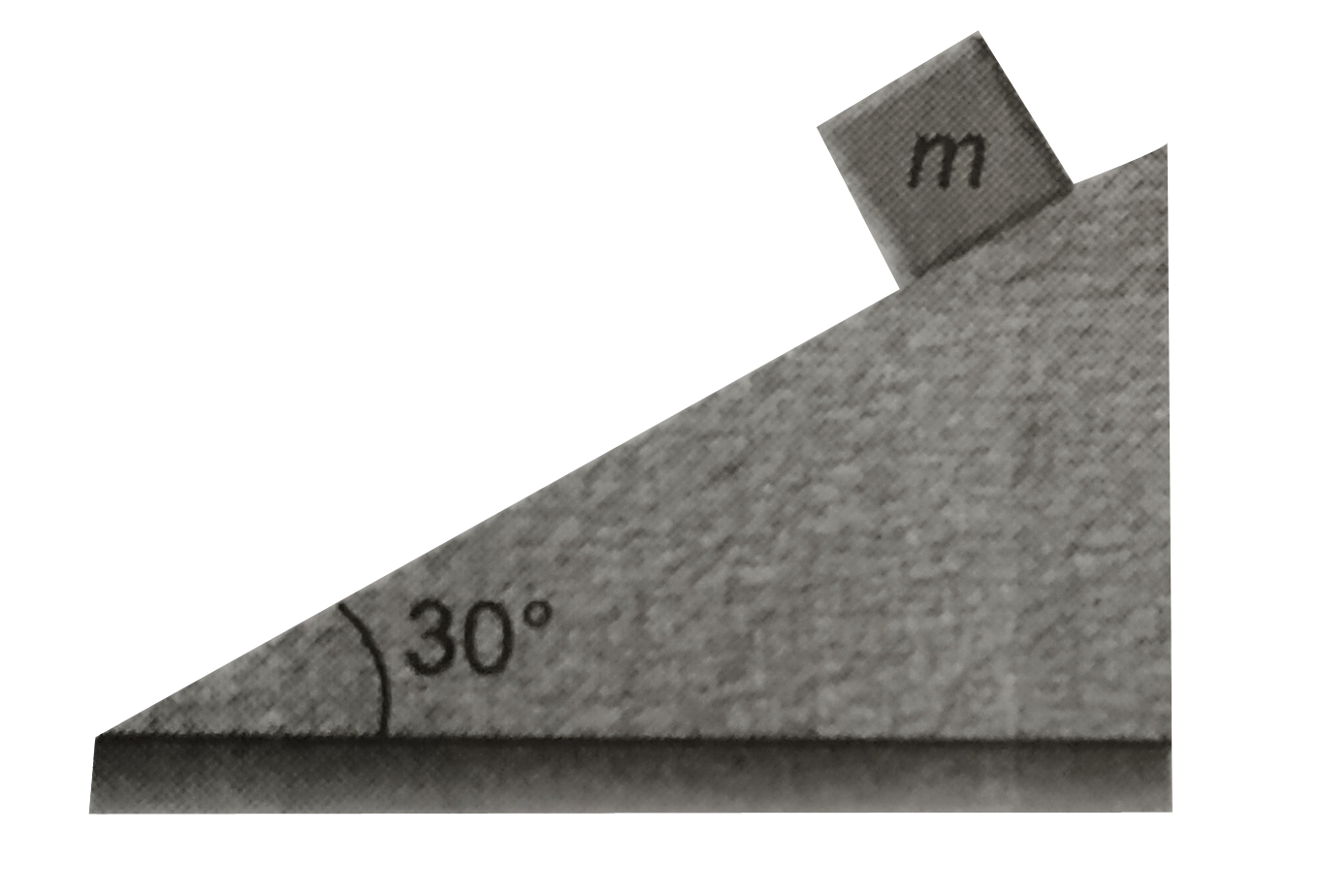
What minimum force is required to move it up the incline with constant speed?A23mgBmg2C7mg6D5mg6A man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
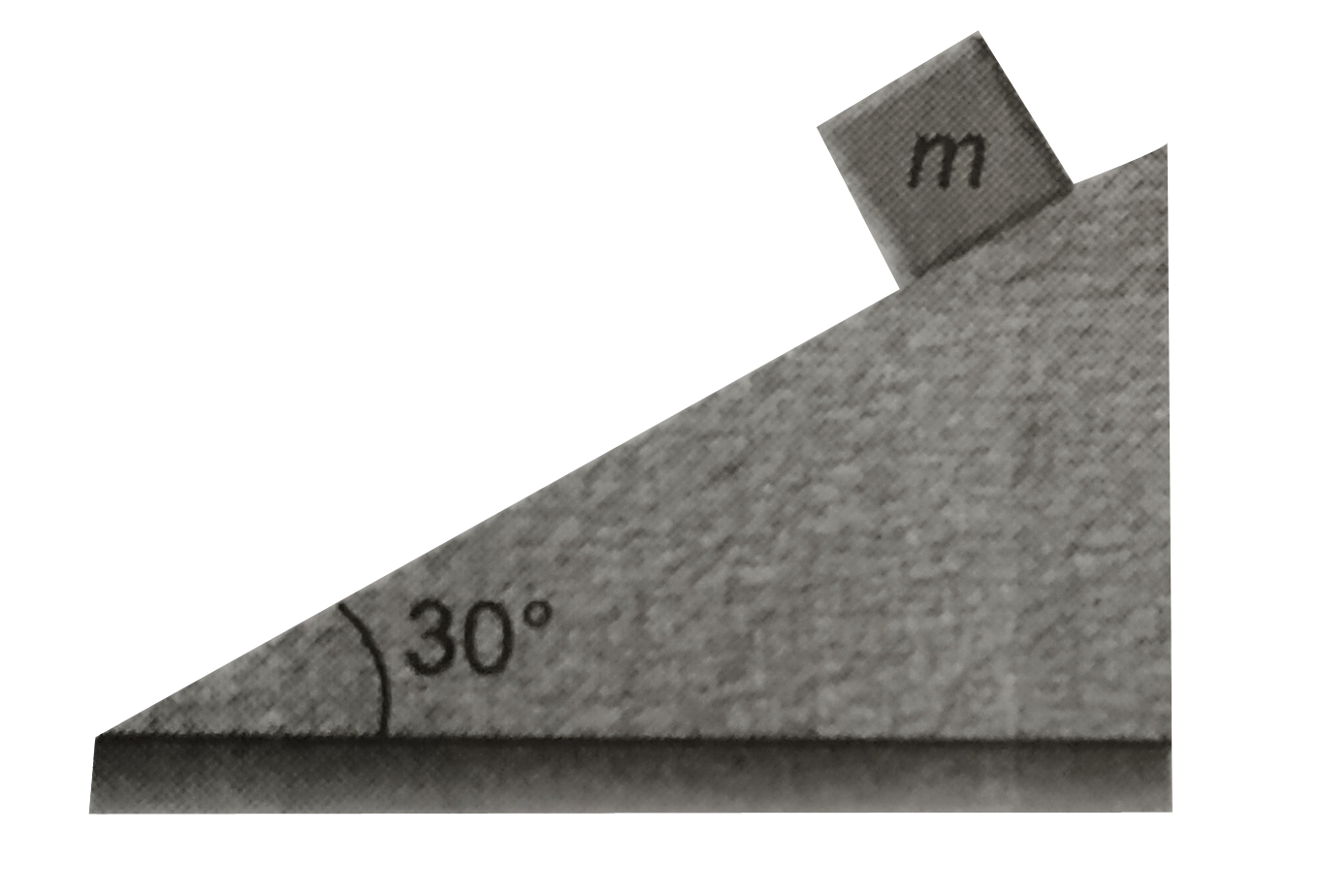
If the man continues pushing the block by force F its acceleration would beAg6Bg4Cg2Dg3A block can slides down an inclined plane of slope and θ, with constant velocity. If it is projected up the same plane with an initial speed v0, then
AThe distance moved up the incline before coming to rest v202gsinθBThe distance moved up the incline before coming to rest v204gsinθCBlock will remain at rest after reaching maximum heightDBlock will moving down with uniform velocity after reaching maximum height
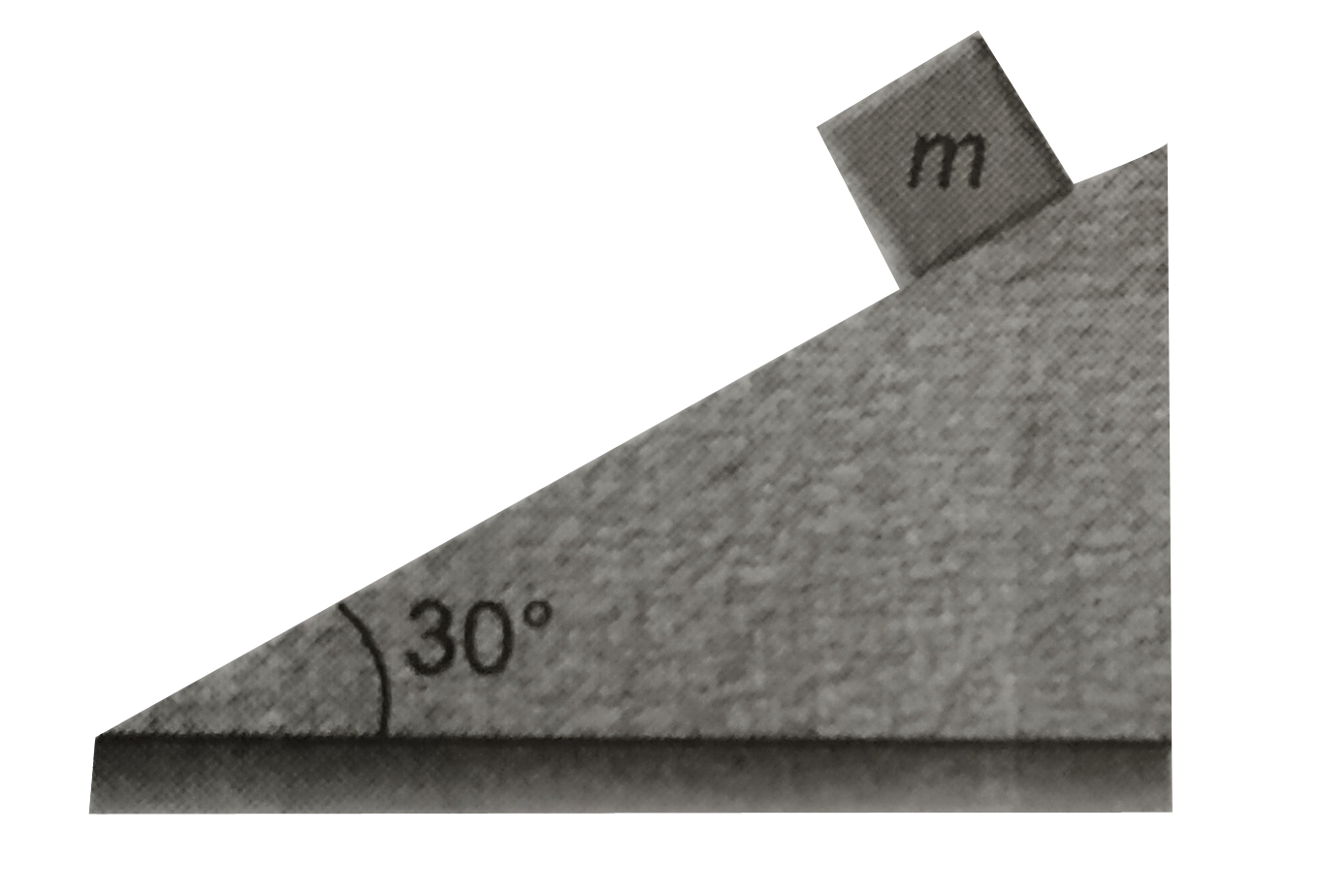
A man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
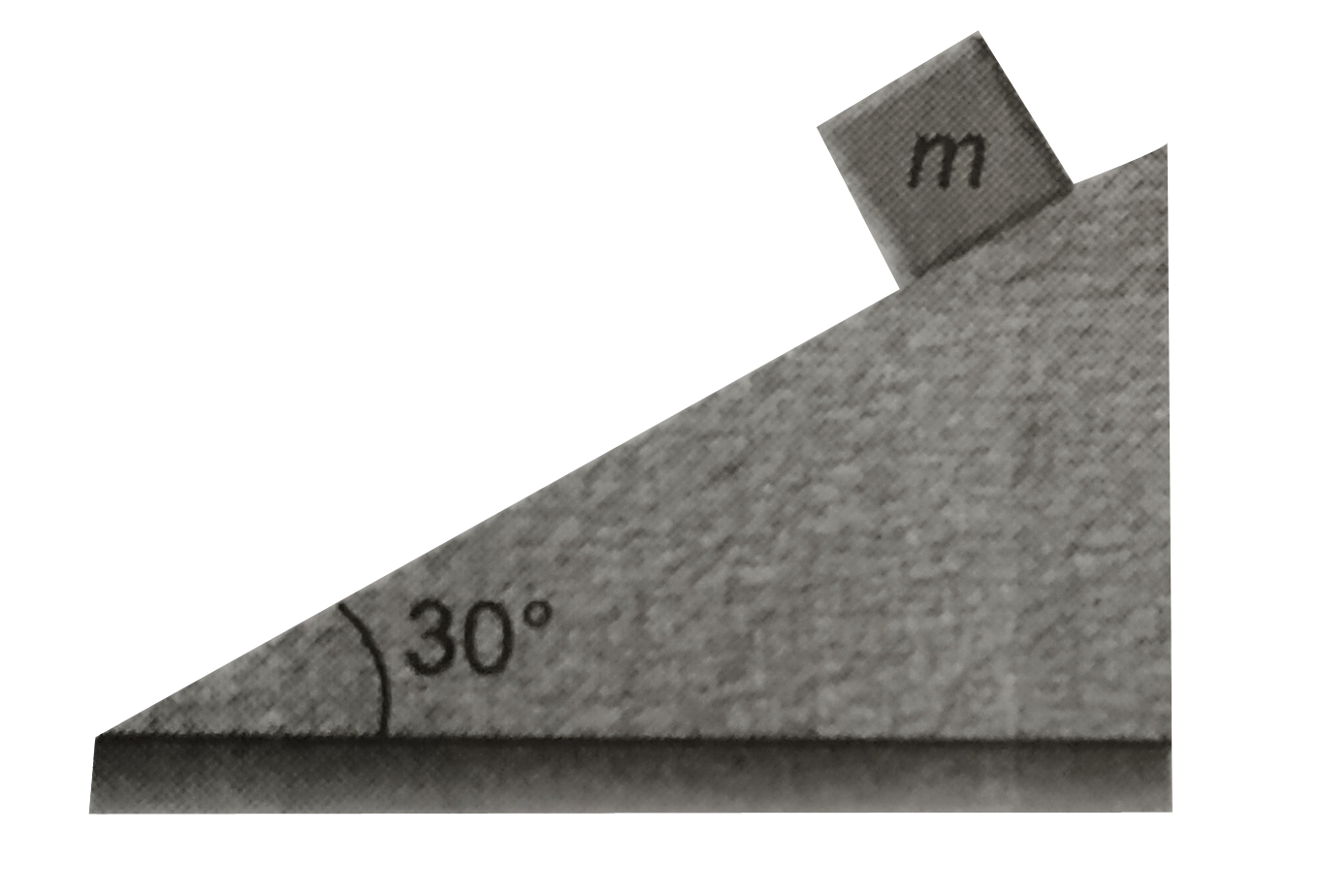
What is the value of F?
View SolutionA man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
What is the value of μs, the coefficient of static friction?
View SolutionA man wants to slide down a block of mass m which is kept on a fixed inclined plane of inclination 30∘ as shown in the figure .Initially the block is not sliding .
To just start sliding the man pushed the block down the inclined with a force F. Now , the block starts accelerating. To move it downwards with conatant speed the man starts pulling the block with same force. Surface are such that ratio of maximum static friction to kinetic friction is 2 Now, answer the following questions.
If the man wants to move the block up the inclined , what minimum force is required to start the motion?
View SolutionA block is at rest on an inclined plane making an angle α with the horizontal . As the angle α of the incline is increased the block starts slipping when the angle of inclination becomes θ. The coefficient of static friction between the block and the surface of the inclined plane is or A body starts sliding down at an angle θ to the horizontal. Then the coefficient of friction is equal to
View SolutionA block is at rest on an inclined plane making an angle α with the horizontal. As the angle of the incline is increased the block start slipping when the angle of inclination becomes θ then coefficient of friction is equal to
View Solution
