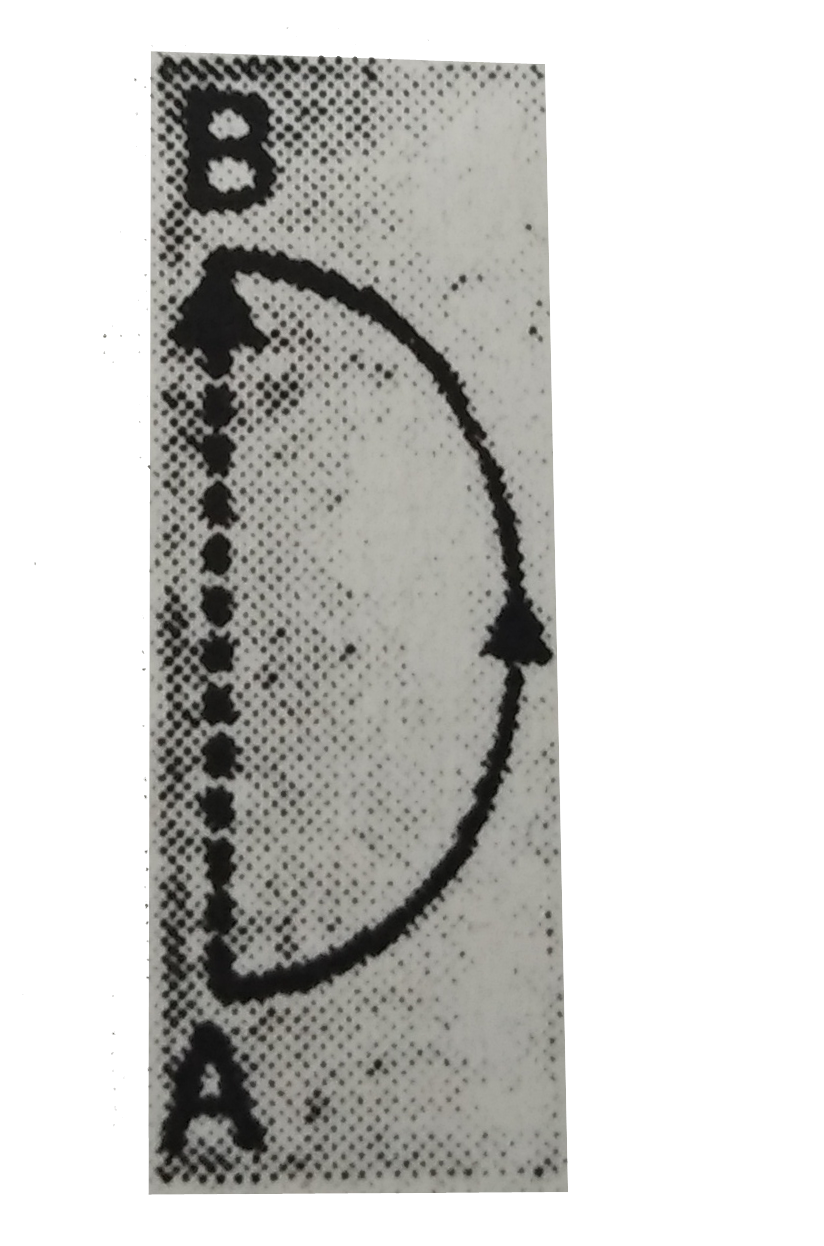
An old man moves on a semi-circular track of radius 7 km during a morning walk. If he starts at one end of the truck and reaches at the other end, the distance and displacement of the person are :
20 km, 7 km
22km, 14km
14km, 7km
All of these
The correct Answer is:B
To solve the problem of the old man walking on a semi-circular track of radius 7 km, we need to calculate both the distance traveled and the displacement. Here’s a step-by-step solution:
Step 1: Understand the Track
The old man is walking on a semi-circular track, which means he starts at one end of the semi-circle and walks to the other end.
Step 2: Calculate the Distance
The distance traveled by the old man is the length of the semi-circular path. The formula for the circumference of a full circle is given by:
Circumference=2πr
Since he is walking on a semi-circle, the distance he travels is half of the circumference:
Distance=12×2πr=πr
Given that the radius r=7 km, we can substitute this value into the equation:
Distance=π×7
Using π≈227:
Distance=227×7=22 km
Step 3: Calculate the Displacement
Displacement is defined as the shortest distance from the initial position to the final position. In this case, the shortest path from point A (starting point) to point B (ending point) is a straight line across the diameter of the semi-circle.
The diameter d can be calculated as:
d=2r
Substituting the radius:
d=2×7=14 km
Thus, the displacement is 14 km.
Final Results
- Distance traveled: 22 km
- Displacement: 14 km
The old man is walking on a semi-circular track, which means he starts at one end of the semi-circle and walks to the other end.
The distance traveled by the old man is the length of the semi-circular path. The formula for the circumference of a full circle is given by:
Circumference=2πr
Since he is walking on a semi-circle, the distance he travels is half of the circumference:
Distance=12×2πr=πr
Given that the radius r=7 km, we can substitute this value into the equation:
Distance=π×7
Using π≈227:
Distance=227×7=22 km
Displacement is defined as the shortest distance from the initial position to the final position. In this case, the shortest path from point A (starting point) to point B (ending point) is a straight line across the diameter of the semi-circle.
d=2r
Substituting the radius:
d=2×7=14 km
Thus, the displacement is 14 km.
- Distance traveled: 22 km
- Displacement: 14 km
Similar Questions
An old person moves on a semi circulasr track of radius 40.0 m during a moirning walk. If he starts at one end of the track and reaches at the other end, find the distance covered and the displacement of the person.
View SolutionA person moves on a semi - circular track of radius radius 42 m during a morning walk. He starts at one end of the track and reaches the other end. Find the distance covered and the displacment of the person .
View Solution
Knowledge Check
An old person moves on a semi circulasr track of radius 40.0 m during a moirning walk. If he starts at one end of the track and reaches at the other end, find the distance covered and the displacement of the person.
A126 m, 80 mB80 m, 126 mC80 m, 252 mD252 m, 80 mA boy of mass m is standing at one end of a boat of mass M and length l. The boy walks to the other end, the displacement of the
ACM is zeroBboat is mlM+mCboy is mlM+mDboat is mMlA boy completes one round of a circular track of radius 20 m in 50 seconds. The displacement at the end of 4 minute 10 second will be
A40 mB20 mC80πmDZero
A person moves on a semicircular track of radius 40 m. If he starts at one end of the track and reaches the other end, find the distance covered and magnitude of displacement of the person.
View SolutionMukesh walks around a circular track of radius 14 m with a speed of 4 km/hr. If he takes 20 rounds of the track, for how long does he walk?
View SolutionA person moves on a circular track of radius R = 10 m in anticlockwise sense, along ACB such that ∠AOB=120∘. Find distance traversed and magnitude of displacement.
View SolutionAn athlete is running on a circular track PQRS of radius 100 m shown below
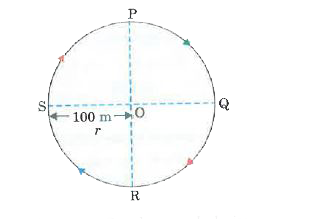
He starts from point P and moves clockwise.
(i) Find the distance travelled by him and displacement when he
(a) reaches Q (b) moves from Q to S (c ) reaches P again after one revolution
(ii) If he completes one revolution in 5 minutes find his
(a) average speed (b) average velocity
View SolutionA glass marble moves from one end of a semiciecular arc of radius R to the other end of the arc, The ratio of distance travelled by the marble to its displacement is
View Solution
