Let A, B, C, D be points on a vertical line such that AB = BC = CD. If a body is released from position A, the times of descent through AB, BC and CD are in the ratio.
:√2−1:√3−√2
1:√2:√3
1:2:3
1:4:9
The correct Answer is:A
Step by step video & text solution for Let A, B, C, D be points on a vertical line such that AB = BC = CD. If a body is released from position A, the times of descent through AB, BC and CD are in the ratio. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If A, B, C and D are four numbers such that A:B=2:3,B:C=4:5,C:D=5:8, then A:D is equal to
A1:3B3:1C2:3D3:2If AB =112cm, BC=16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is
A90∘B120∘C60∘D150∘Let D be the mid-point of a straight line AB and let C be a point different from D such that AC=BC, then.
AAC⊥ABB∠BDC=90∘C∠BDC is acuteD∠BNDC>90∘
In Fig. 2.15, points A,B,C,D and E are collinear such that AB = BC = CD= DE. Then
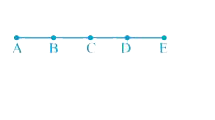
AE = ________ × AB.
View SolutionIn Fig. 2.15, points A,B,C,D and E are collinear such that AB = BC = CD= DE. Then
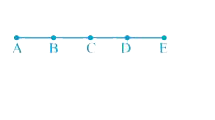
AD = AB + ________
View SolutionIn Fig. 2.15, points A,B,C,D and E are collinear such that AB = BC = CD= DE. Then
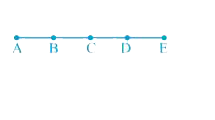
AD = AC + ___________
View SolutionLet D be the mid-point of a straight line AB and let C be a point different from D such that AC=BC, then.
View SolutionAb Bc Cd De Ef Fg G?
View Solution