Which of the following graphs has a positive , decreasing slope ?
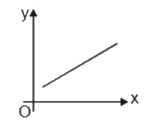
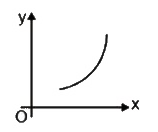
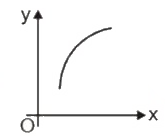
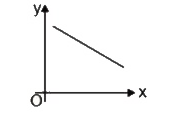
The correct Answer is:C
To determine which graph has a positive, decreasing slope, we need to analyze the properties of the slope in relation to the angle it forms with the x-axis.
1. Understanding Slope: The slope of a graph is defined as the tangent of the angle (θ) that the graph makes with the x-axis. Mathematically, slope (m) = tan(θ).
2. Condition for Positive Slope: For the slope to be positive, the angle θ must be between 0° and 90°. This means that as long as the graph is rising, the slope will be positive.
3. Condition for Decreasing Slope: A decreasing slope means that as we move along the graph, the angle θ is decreasing. This implies that the tangent of the angle (and hence the slope) is getting smaller.
4. Analyzing the Options:
- Option 1: If the slope is constant, then θ does not change, meaning the slope is not decreasing.
- Option 2: If the slope is increasing (θ is increasing), then the slope cannot be decreasing.
- Option 3: If we draw tangents at different points on this graph and observe that the angle θ is decreasing as we move along the graph, then the slope is positive and decreasing.
- Option 4: If the slope is negative, then it does not satisfy the condition of being positive.
5. Conclusion: Based on the analysis, the graph that has a positive and decreasing slope is Option 3.
- Option 1: If the slope is constant, then θ does not change, meaning the slope is not decreasing.
- Option 2: If the slope is increasing (θ is increasing), then the slope cannot be decreasing.
- Option 3: If we draw tangents at different points on this graph and observe that the angle θ is decreasing as we move along the graph, then the slope is positive and decreasing.
- Option 4: If the slope is negative, then it does not satisfy the condition of being positive.
Similar Questions
Knowledge Check
Which of the following compounds has a positive enthalpy of solution?
ALiFBLiClCLiBrDLiIWhich of the following compounds has a positive enthalpy of solution?
ALiFBLiClCLiBrDLiIIn Elingham diagrams of ΔG de formation Vs T, which of the following graphs has negative slope?
AC→COBFe→Fe2O3CM>oMgODAll of these
Which of the following graphs are graphs of a function?

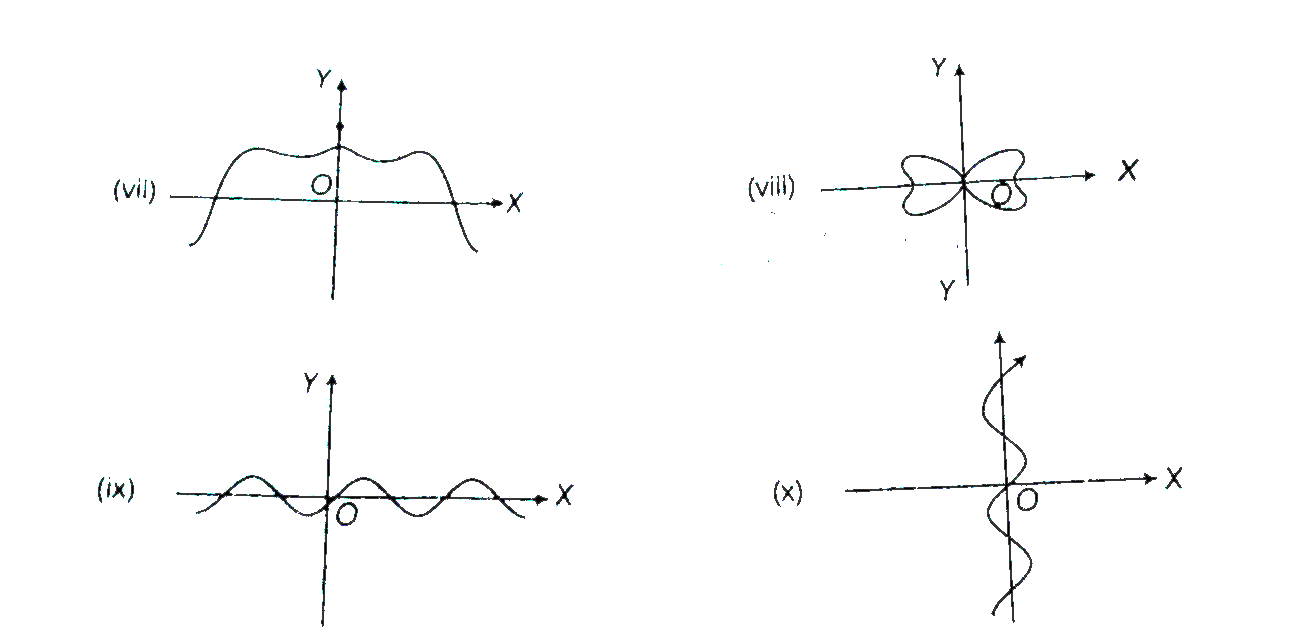
View SolutionWhich of the following graphs represents the position time-graph of a particle moving with negative velocity?
View SolutionWhich one of the following has △S∘ positive?
View SolutionWhich among the following has carbon as the positive electrode?
View SolutionWhich among the following has carbon as the positive electrode?
View Solution
