Question

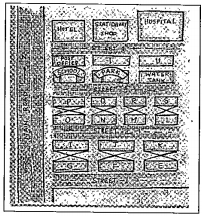
(Street plan): A city has two main roads which cross each other at the centre of the city. These two roads are along the North-South direction and East_West direction. All the other streets of the city run parallel to these roads and are 200m apart. There are 5 streets in each direaction. Using ${\displaystyle 1cm=200cm,}$ draw a model of teh city on your note book. Represent the roads/streets by single lines.
There are many cross-streets in your model. A particular cross-street is made by two streets, one running in the North-South directin and another in the East-West direction. Each cross street is rreferred to in the following manner, If the ${\displaystyle 2^{nd}}$ steeet running in teh North-South direction ${\displaystyle 5^{th}}$ in the East-West direction meet at some crossing, then we will call this cross-street (2,5). Using this convection, find:
How many cross-streets can be referred to as (3,4)

Answer

There is only one cross-street, which can be referred as (3,4)