If coal gives off 7000 kcal/ kg when it is burnt, how much coal will be needed to heat a house that requires 4.2×107 kcal for the whole winter? Assume that an additional 30 percent of the heat is lost up the chimney.
The correct Answer is:7.8×103kg
To solve the problem, we need to calculate the amount of coal required to heat a house that needs 4.2x107 kcal for the winter, considering that 30% of the heat is lost through the chimney.
1. Determine the total heat required considering the heat loss:
- The house requires 4.2x107 kcal.
- Since 30% of the heat is lost, we need to find the total heat that must be produced to account for this loss.
- The heat lost is calculated as:
\(
\text{Heat lost} = 0.3 \times (4.2 \times 10^7) = 1.26 \times 10^7 \text{ kcal}
\)
- Therefore, the total heat required is:
\(
\text{Total heat required} = \text{Required heat} + \text{Heat lost} = 4.2 \times 10^7 + 1.26 \times 10^7 = 5.46 \times 10^7 \text{ kcal}
\)
2. Calculate the amount of coal needed:
- We know that 1 kg of coal produces 7000 kcal.
- To find the amount of coal needed, we divide the total heat required by the heat produced per kg of coal:
\(
\text{Amount of coal required} = \frac{\text{Total heat required}}{\text{Heat produced per kg}} = \frac{5.46 \times 10^7}{7000}
\)
3. Perform the division:
- First, simplify the division:
\(
\frac{5.46 \times 10^7}{7000} = \frac{5.46 \times 10^7}{7 \times 10^3} = \frac{5.46}{7} \times 10^{7-3} = \frac{5.46}{7} \times 10^4
\)
- Now, calculate \( \frac{5.46}{7} \):
\(
\frac{5.46}{7} \approx 0.78
\)
- Therefore, the amount of coal required is:
\(
0.78 \times 10^4 \text{ kg} = 7.8 \times 10^3 \text{ kg}
\)
Final Answer:
The amount of coal required is approximately 7.8 x 10^3 kg.
- The house requires 4.2x107 kcal.
- Since 30% of the heat is lost, we need to find the total heat that must be produced to account for this loss.
- The heat lost is calculated as:
\(
\text{Heat lost} = 0.3 \times (4.2 \times 10^7) = 1.26 \times 10^7 \text{ kcal}
\)
- Therefore, the total heat required is:
\(
\text{Total heat required} = \text{Required heat} + \text{Heat lost} = 4.2 \times 10^7 + 1.26 \times 10^7 = 5.46 \times 10^7 \text{ kcal}
\)
- We know that 1 kg of coal produces 7000 kcal.
- To find the amount of coal needed, we divide the total heat required by the heat produced per kg of coal:
\(
\text{Amount of coal required} = \frac{\text{Total heat required}}{\text{Heat produced per kg}} = \frac{5.46 \times 10^7}{7000}
\)
- First, simplify the division:
\(
\frac{5.46 \times 10^7}{7000} = \frac{5.46 \times 10^7}{7 \times 10^3} = \frac{5.46}{7} \times 10^{7-3} = \frac{5.46}{7} \times 10^4
\)
- Now, calculate \( \frac{5.46}{7} \):
\(
\frac{5.46}{7} \approx 0.78
\)
- Therefore, the amount of coal required is:
\(
0.78 \times 10^4 \text{ kg} = 7.8 \times 10^3 \text{ kg}
\)
The amount of coal required is approximately 7.8 x 10^3 kg.
Topper's Solved these Questions
- HEAT AND THERMAL EXPANSIONBook:PHYSICS GALAXY - ASHISH ARORAChapter:HEAT AND THERMAL EXPANSIONExercise:ADVANCE MCQs WITH ONE OR MORE OPTIONIS CORRECT
Explore 24 Videos
- GEOMETRICAL OPTICSBook:PHYSICS GALAXY - ASHISH ARORAChapter:GEOMETRICAL OPTICSExercise:Unsolved Numerical Problems
Explore 107 Videos
- HEAT TRANSFER Book:PHYSICS GALAXY - ASHISH ARORAChapter:HEAT TRANSFER Exercise:Unsolved Numerical Problems for Preparation of NSEP,INPhO&IPhO
Explore 66 Videos
Similar Questions
5.00 kg of liquid water is heated to 100.0∘C in a closed system At this temperature , the density of liquid water is 958 kg/m3 . The pressure is maintained at atmospheric pressure of 1.01×105 Pa. A moveable piston of negligible weight rests on the surface of the water. The water is then converted to steam by adding an additional amount of heat to the system. When all of the water is converted, the final volume of the steam is 8.50m3. The latent heat of vaporization of water is 2.26×106 J/kg.
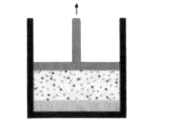
How much heat is added to the system in the isothermal process of converting all of the water into steam?
View SolutionA meteorite has mass of 500 kg and is composed of a metal. The temperature of the meteor is −20∘C and its speed is 10 km/hr when it is at large distance from a planet. The meteorite crashes into the planet and its entire kinetic energy gets converted into heat. This heat is equally shared between the planet and the meteorite. Assume that the heating of meteorite is uniform and the average specific heat capacity of the metal, for its solid, liquid and vapour phase, is 1200Jkg−1.∘C−1. The latent heat of fusion and vaporization of the metal are Lf=4×105Jkg−1 and Lv=1.1×107Jkg−1 respectively. The melting point and boiling points are 380.∘C and 2380.∘C respectively. Find the temperature of the meteorite material immediately after the impact.
Take: G=6.6×1011Nm2kg−2, mass of planet M=6×1024kg, radius of planet R = 6600 km
View Solution
Knowledge Check
Heat of combustion of methane is -800 kJ. How much heat is required for combustion of 4×10−4 kg of methane ?
A-15 kJB−18kJC-20 kJD-22 kJA person trying to lose weight by burning fat filts a mass of 10kg upto a being of 1m1000 time . Assume that the potential energy lost each time be lower the mass is dissipated . How much far will be use up considering the work done only when the weight is lifted up ? Far supplies 3.8×107J of energy per kg wich is canverted to mechanical energy with x20% efficiency rate Take =9.8ms−2
A9.89×10−3kgB12.89×10−3kgC2.45×10−3kgD6.45×10−3kg
A thermocol vessel contains 0.5kg of distilled water at 30∘C. A metal coil of area 5×10−3m2, number of turns 100, mass 0.06kg and resistance 1.6Ω is lying horizontally at the bottom of the vessel. A uniform time-varying magnetic field is set up to pass vertically through the coil at time t=0. The field is first increased from zero to 0.8T at a constant rate between 0 and 0.2s and then decreased to zero at the same rate between 0.2and0.4s. the cycle is repeated 12000 times. Make sketches of the current through the coil and the power dissipated in the coil as function of time for the first two cycles. Clearly indicate the magnitude of the quantities on the axes. Assumes that no heat is lost to the vessel or the surroundings. Determine the final tempreture of water under thermal equilibrium. Specific heat of metal =500jkg−1K−1 and the specific heat of water =4200jkg−1K−1. Neglect the inductance of coil.
View SolutionDuring exercise, a person may give offf 180 kcal of heat in 30 min by evaporation of water from the skin. How much water has been lost? Given that latent heat of vaporization of water is 540 cal/gm.
View SolutionA sample of air weighing 1.18g occupies 1.0×103cm3 when kept at 300Kand1.0×105 pa. When 2.0cal of heat is added to it constant volume, its temperature increases by 1∘C. Calculate the amount if heat needed to increases the temperature of air by 1∘C at constant pressure if the mechanical equivalent of heat si 4.2×10−1 . Assume that air behaves as an ideal gas.
View Solution5.00 kg of liquid water is heated to 100.0∘C in a closed system At this temperature , the density of liquid water is 958 kg/m3 . The pressure is maintained at atmospheric pressure of 1.01×105 Pa. A moveable piston of negligible weight rests on the surface of the water. The water is then converted to steam by adding an additional amount of heat to the system. When all of the water is converted, the final volume of the steam is 8.50m3. The latent heat of vaporization of water is 2.26×106 J/kg.
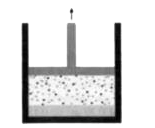
How much work is done by this closed system during this isothermal process?
View SolutionQuantity of heat gained by 100 kg of iron in raising its temperature by 10∘C is 11×104cal. If a heater can supply heat at the rate of 1000 J s−1, how much time does it take to heat an iron block of mass 2.5 kg when the mecury level in a Fahrenheit thermometer rises by 45 divisions.
View SolutionOn a winter day when the atmospheric temperature drops to −10∘C, ice form on the surface of a lake.
(a) Calculate the rate of increases of thickness of the ice when 10cm of ice is already formed.
(b) Calculate the total time taken in forming 10cm of ice. Assume that the temperature of the entire water reaches 0∘C before the ice starts froming. Density of water =1000kg/m3, latent heat of fusion of ice =3.36×105J/kg and thermal conductivity of ice =1.7W/m−∘C. Neglect the expansion of water on freezing
View Solution