A pole has to be erected at a point on the boundary of a circular park of diameter 13 metres in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 metres. Is it the possible to do so? If yes, at what distances from the two gates should the pole be erected?
Diameter,AB=17m
AC−BC=7
∠ACB=90o
using pythagoras theorem,
AC2+BC2=AB2..(1)
AC−BC=7..(2)
Subtituting AC from (2) in(1), we get
(7−BC)2+BC2=172
BC2+7BC−120=0
(BC−8)(BC+15)=0
BC=−15,8
BC is a diameter and cannot be negative
so BC=8m
AC=7+BC=15m
Topper's Solved these Questions
Similar Questions
A pole has to be erected at a point on the boundary of a circular park of diameter 13 metres in such a way that the differences of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 metres. Is it possible to do so? If yes, at what distances from the two gates should the pole be erected?
View Solution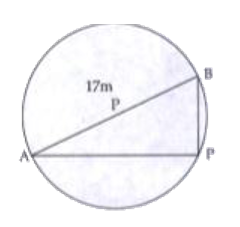
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
What is the length of (AP+BP) ?
View Solution
Knowledge Check
The locus of a point which moves so that the difference of the squares of its distances from two given points is constant, is a
Astraight lineBplaneCsphereDnone of theseThe locus of a point which moves so that the difference of the squares of its distance from two given points is constant, is a
Astraight lineBplaneCsphereDNone of theseA point P is moving in a plane such that the difference of its distances from two fixed points in the same plane is a constant. The path traced by the point P is a/an
ACircleBparabolaCEllipseDHyperbola

Aniket is studying in X standard. He created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
Find the area of triangle ABP.
View Solution
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
Find the distance between pole and gate B.
View Solution
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
Find the distance between pole and gate A.
View Solution
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gets A and B on the boundary is 7m.
Find a quadratic equations in variable x for above situation.
View SolutionA point P moves in such a way that the ratio of its distance from two coplanar points is always a fixed number (≠1) . Then, identify the locus of the point.
View Solution
