An organic compound (A) decomposes according to two parallel first order mechanism.
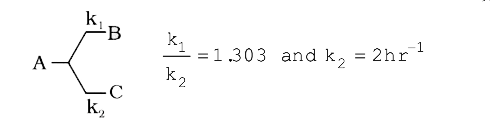 k1k2=1.303andk2=2hr−1
k1k2=1.303andk2=2hr−1
Calculate the ratio of concentration of C to A, if an experiment is allowed to start with only A for one hour?
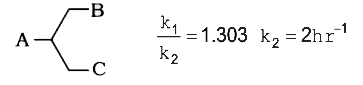
k1k2=1.303k2=2hr−1
sol. [B][C]=k1k2=1.303 where At= concentration after 1hr.
−d[A]dt−[k1+k2][A]
(k1+k2)=2.303tlog10[[At]+[B]+[C][At]]
2.303k2=2.3031log10[1+2.303[C][At]]
k2=log10[1+2.303[C]At]
102=1+2.303[C]At
So 2.303[C][At]=99, [C][At]=992.303
So [C][At]=42.987+43.
Similar Questions
A certain organic compound A decomposes by two parallel first order mechanisms.
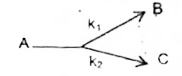
If k1:k2=1:9 and k1=1.3×10−5s−1. Calculate the concentration ratio of C to A if an experiment is started with only A and allowed to run for one hour.
View SolutionCalculate the equilibrium concentration ratio of C to A, if 2.0 mol each of A and B were allowed to come to equilibrium at 300 K.
View Solution
Knowledge Check
For the first-order reaction (C=C0e−kt1) and Tav=k−11. After two average lives concentration of the reactant is reduced to :
A25%B75%C100e%D100e2%For the two step reaction in which both the steps are first order
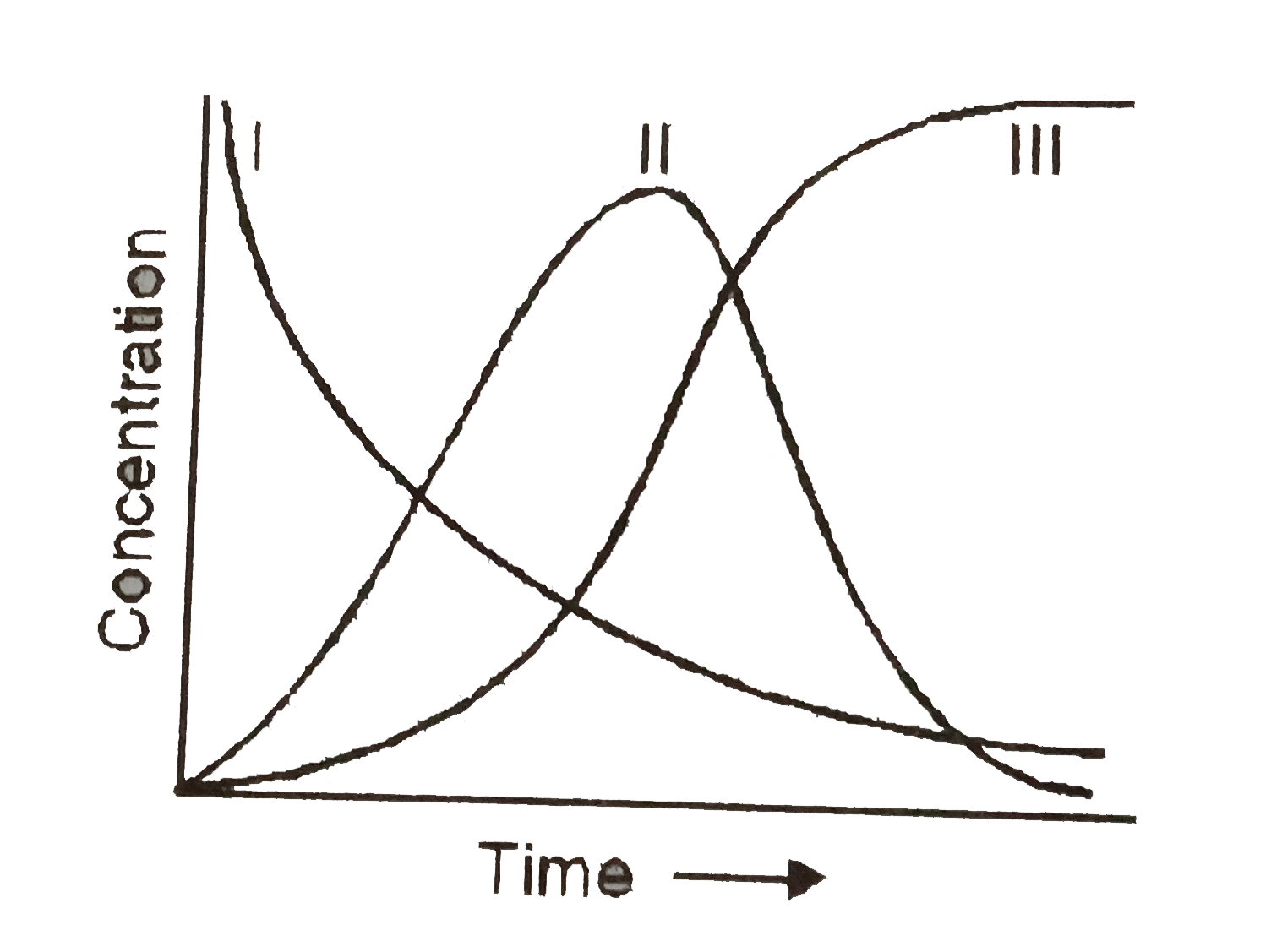
Rk1−−→I
Ik2−−→P
the rate of change of concentration of I is given by the plotAIBIICIIIDNoneFor the consecutive unimolecular first order reaction Ak1−−→Rk2−−→S, the concentration of component A, CA at any time t is given by
AC=CA0e(k1+k2)tBCA=CA0e−(k1+k2)tCCA=CA0e−k1tDCA=CA0ek1t
A substance undergoes first order decomposition. The decomposition follows two parallel first order reactions as
 , k1=1.26×10−4 sec−1andk2=3.6×10−5 sec−1 Calculate the % distribution of B & C.
, k1=1.26×10−4 sec−1andk2=3.6×10−5 sec−1 Calculate the % distribution of B & C.
View SolutionA substance undergoes first order decomposition. The decomposition follows two parallel first order reactions as :
k1=1.26×10−4s−1,k2=3.8×10−5s−1
The percentage distribution of B and C are :
View SolutionFor a reaction, A⇔B equilibrium constant is 1.66 and kforward=0.166hr−1.
Calculate the time (in hour) when concentration of B is 80% of its equilibrium concentration. (Given : In 25=3.20)
View SolutionThe substance undergoes first order decomposition. The decomposition follows two parallel first order reactions as :
K1=1.26×10−4sec−1andK2=3.8×10−5sec−1 The percentage distribution of B and C
View SolutionThe substance undergoes first order decomposition.The decomposition follows two parallel first order reactons as :

K1=10−2sec−1andK2=10−2sec−1
If the corresponding activation energies of parallel reaction are 100 and 120 kJ mol−1 then the net activation energy of A is/are:
View Solution