For the reaction, Products, and at different time interval, [A] values are
At 20 min, rate of reaction in M m will be
To solve the problem, we need to determine the rate of reaction at 20 minutes for the reaction A→Products. We are given the concentration of A at different time intervals, and we can use this information to calculate the rate of reaction.
1. Identify the Concentration Values:
From the data provided:
- At t=0 min, (A)=20M
- At t=5 min, (A)=18M
- At t=10 min, (A)=16M
- At t=15 min, (A)=14M
We can calculate the concentration at t=20 min. Since the concentration decreases consistently by 2 M every 5 minutes, we can predict:
- At t=20 min, (A)=20M−8M=12M
2. Calculate the Change in Concentration:
We need to find the change in concentration from t=0 min to t=20 min:
Δ(A)=(A)t=20−(A)t=0=12M−20M=−8M
3. Calculate the Time Interval:
The time interval from t=0 min to t=20 min is:
Δt=20min−0min=20min
4. Determine the Rate of Reaction:
The rate of reaction is given by the formula:
−d(A)dt=Δ(A)Δt
Substituting the values we calculated:
−d(A)dt=−8M20min=−0.4M/min
Since we are interested in the rate (which is a positive quantity), we take the absolute value:
Rate of reaction=0.4M/min
Final Answer:
The rate of reaction at 20 minutes is 0.4M/min.
From the data provided:
- At t=0 min, (A)=20M
- At t=5 min, (A)=18M
- At t=10 min, (A)=16M
- At t=15 min, (A)=14M
- At t=20 min, (A)=20M−8M=12M
We need to find the change in concentration from t=0 min to t=20 min:
Δ(A)=(A)t=20−(A)t=0=12M−20M=−8M
The time interval from t=0 min to t=20 min is:
Δt=20min−0min=20min
The rate of reaction is given by the formula:
−d(A)dt=Δ(A)Δt
Substituting the values we calculated:
−d(A)dt=−8M20min=−0.4M/min
Since we are interested in the rate (which is a positive quantity), we take the absolute value:
Rate of reaction=0.4M/min
The rate of reaction at 20 minutes is 0.4M/min.
Similar Questions
Knowledge Check
- Question 1 - Select One
For the reaction A→ Product, −d[A]dt=k and at different time interval, [A] values are :
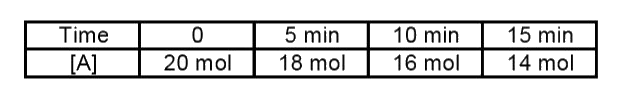
At 20 minute, rate will be :A12 mol/minB10 mol/minC8 mol/minD0.4 mol/min - Question 2 - Select One
For the reaction A→ Product, −d[A]dt=k and at different time interval, [A] values are :

At 20 minute, rate will be :A12 mol/minB10 mol/minC8 mol/minD0.4 mol/min - Question 3 - Select One
For the rection 3A → Products the value of k=1×10−3 L/(mol-min) the value of −d[A]dt in mol/L-sec when [A] = 2 M is :
A6.67×10−3B1.2×10−2C2×10−4D4×10−3
For the reaction A→ prouducts, the following data is given for a particular run.
time(min.):0515251[A](M−1):1248
Determine the order of the reaction.View SolutionGiven X → product (1st order reaction)
conc.(mol/lit)0.010.0025time(min)040
Initial rate of reaction (in mol/l/min)View SolutionThe rate constant of a reaction is 0.0693−1min. Starting with 10mol, the rate of the reaction after 10min is
View SolutionThe rate constant of a reaction is 0.0693−1min. Starting with 10mol, the rate of the reaction after 10min is
View SolutionFor the reaction ,
Ak−−→B+C
Following data are obtained

What will be the rate of reaction (in molL−1−1min, when conc of 'A' is 3M.View Solution
