Let a total charge 2Q be distributed in a sphere of radius R, with the charge density given by , ρ(r)=kr, where r is the distance from the centre. Two charge A and B, of –Q each, are placed on diametrically opposite points, at equal distance, a from the centre. If A and B do not experience any force, then:
a=2−1/4R
a=R√3
a=8−1/4R
a=3R21/4
The correct Answer is:C
Consider force on A due to B
|F|=Q24π∈0(2a)2=Q216π∈0a2
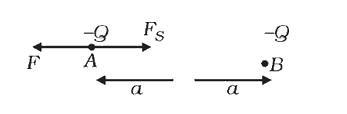
Since A is in equilibrium, force on it due to changed sphere, Fs=F (magnitude)
So, electric field at distance a from centre
E=Q16π∈0a2
If r>R , result for electric field is E=2Q4π∈0r2
So, assuming a>R, E=Q16π∈0a2=2Q4π∈0r2 ⇒ r=2√2a ⇒ a=v2√2
But the result was only valid for r>R ⇒ The point is inside the sphere and a<R
Let electric field inside the sphere at distance r from centre be E, then by gauss law
E(4πr2)=1∈0r∫0kx(4πx2)dx ⇒ E=kr24∈0
Also R∫0kx(4πx2)dx=2Q ⇒ k=2QπR4 ⇒ E=Qr22π∈0R4=Qa22π∈0R4
Comparing with E=Q16π∈0a2 ⇒ a=8−1/4R
Similar Questions
A sphere of radius R carries charge density p proportional to the square of the distance from the centre such that p=Cr2, where C is a positive constant. At a distance R/2 from the centre, the magnitude of the electric field is :
View SolutionCharge is distributed within a sphere of radius R with a volume charge density p(r)=Ar2e−2r/a, where A and a are constants. If Q is the total charge of this charge distribution, the radius R is :
View Solution
Knowledge Check
A sphere of radius R has a charge density σ. The electric intensity at a point at a distance r from its centre is
AE=σR2∈0kr2BE=σ∈0kCE=R2DE=∈0kr2σ2Let a total charge 2Q be distributed in a sphere of radius R, with the charge density given by , ρ(r)=kr, where r is the distance from the centre. Two charge A and B, of –Q each, are placed on diametrically opposite points, at equal distance, a from the centre. If A and B do not experience any force, then:
Aa=R/2Ba=RCa=2RDa=3R/4Charge density of a sphere of radius R is ρ=ρ0r where r is distance from centre of sphere.Total charge of sphere will be
 AρπeR2B2ρ0πeR2C3ρ0πeR2D4ρ0πeR2
AρπeR2B2ρ0πeR2C3ρ0πeR2D4ρ0πeR2
A solid sphere of radius R has total charge Q. The charge is distributed in spherically symmetric manner in the sphere. The charge density is zero at the centre and increases linearly with distance from the centre. (a) Find the charge density at distance r from the centre of the sphere. (b) Find the magnitude of electric field at a point ‘P’ inside the sphere at distance r1 from the centre.
View SolutionThe volume charge density inside of sphere of radius R is given by ρ=ρ0(1−rR) ,where ρ0 is constant and r distance from centre.
(a) Find total charge Q inside sphere.
Electric field outside sphere at distance r from centre.
(b) Electric field outside sphere at distance r from centre.
(c) Electric field inside sphere at distance r from centre.
(d) At what distance from centre electric field is maximum and its value?
(e ) Sketch E versus r graph.
View SolutionCharge Q is distributed on two concentric spheres of radius r and R resp. if charge density of both spheres is same then electric potential at centre will be
View SolutionA sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the centre. What is the ratio of the magnitude of the electric field at a distance 2 R from the centre to the magnitude of the electric field at a distance of R/2 from the centre?
View SolutionA spherical shell of radius R is given charge 3q on its surface and a point charge q is placed at distance R/2 from its centre C. Also there is a charge 2q placed outside the shell at a distance of 2R as shown. Then

View Solution
