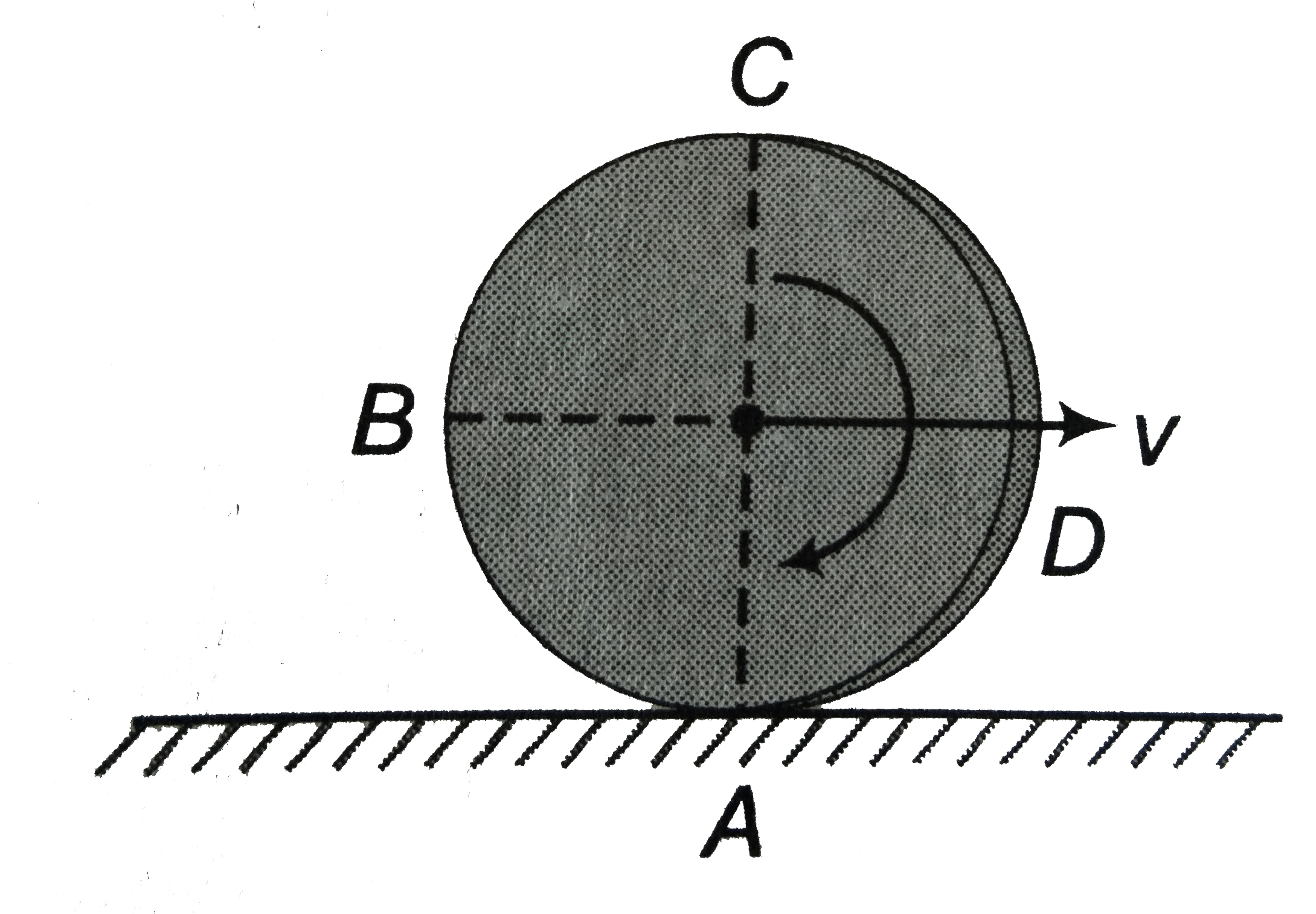
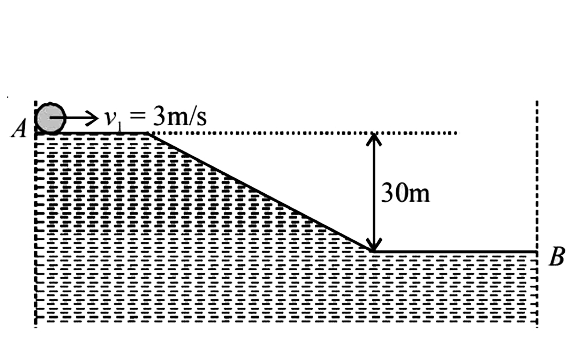
Two identical uniform discs roll without slipping on tow different sufaces AB and CD (see figure) starting at A and C with linear speeds v1andv2 respectively, and always remain in contact with the surfaces. If they reach B and D with the same linear speed v1=3m/sthenv2∈m/sis(g=10m/s2)

Kinetic energy of a purely rolling disc =12mV2+12(m2)v2=34mV2
Using conservation of energy on both discs and equating their final energies.
34mV21+mg.30=34mV22+mg.27,3mg=34m(V22−V21)
4g=V22−9,V22=49,V2=7.
Similar Questions
Knowledge Check
A symmetrical body of mass M and radius R is rolling without slipping on a horizontal surface with linear speed v. Then its angular speed is
Av/RBContinuously increasingCDependent on mass MDIndependent of radius (R)Two identical photo-cathodes receive light of frequencies v1 and v2. If the velocities of the photoelectrons (of mass m) coming out are v1 and v2 respectively, then
Av1−v2=[(2hm)(v1−v2))]1/2Bv21−v22=2hm(v1−v2)Cv1−v2=[(2hm)(v1−v2))]1/2Dv21−v22=2hm(v1−v2)Two identical cylinders roll from rest on two identical planes of slant lengths s and 2s but of the same height h. Then, the velocities, v1 and v2 acquired by the cylinders when they reach the bottom of the incline are related as.
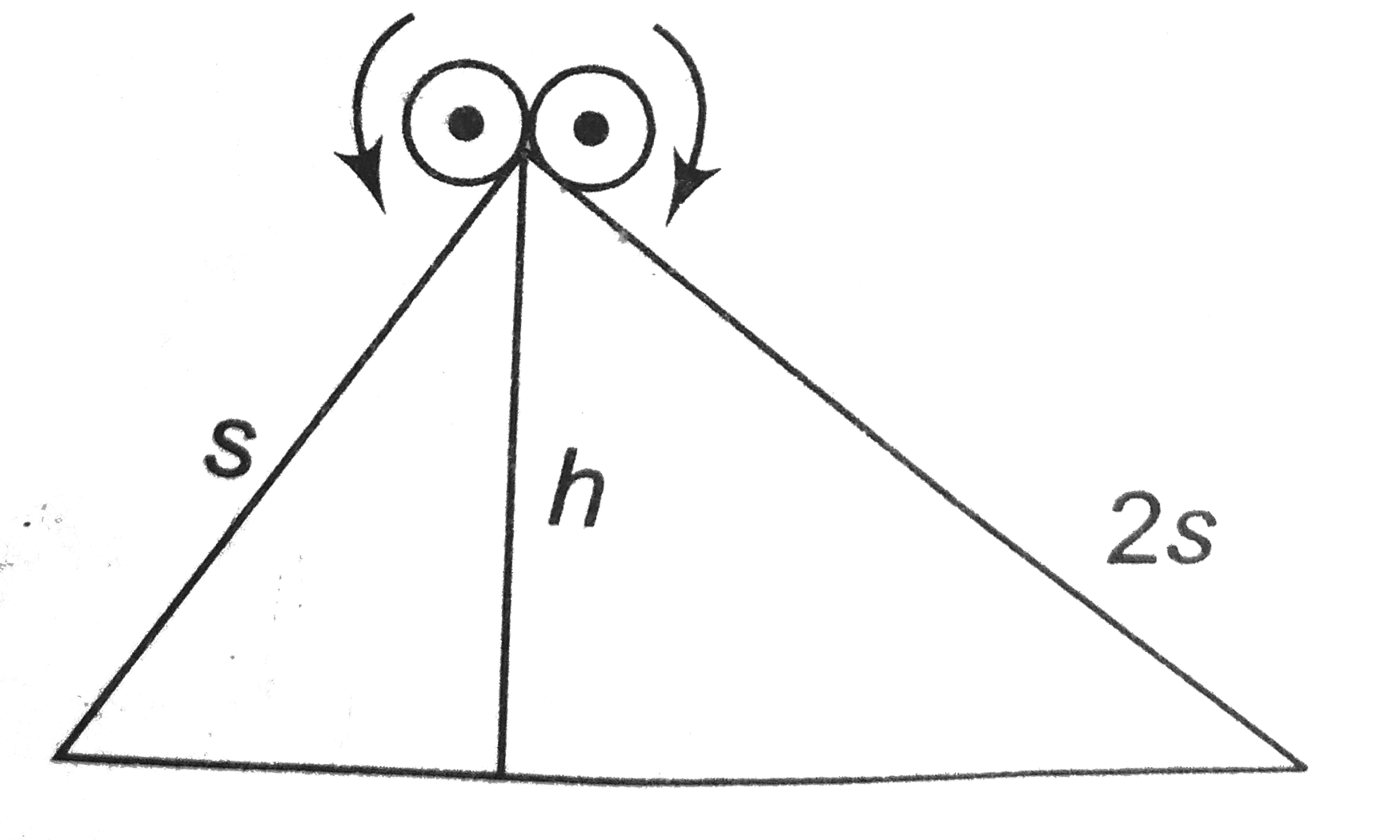 .Av1=v2Bv1=2v2C2v1=v2DNone of the above.
.Av1=v2Bv1=2v2C2v1=v2DNone of the above.
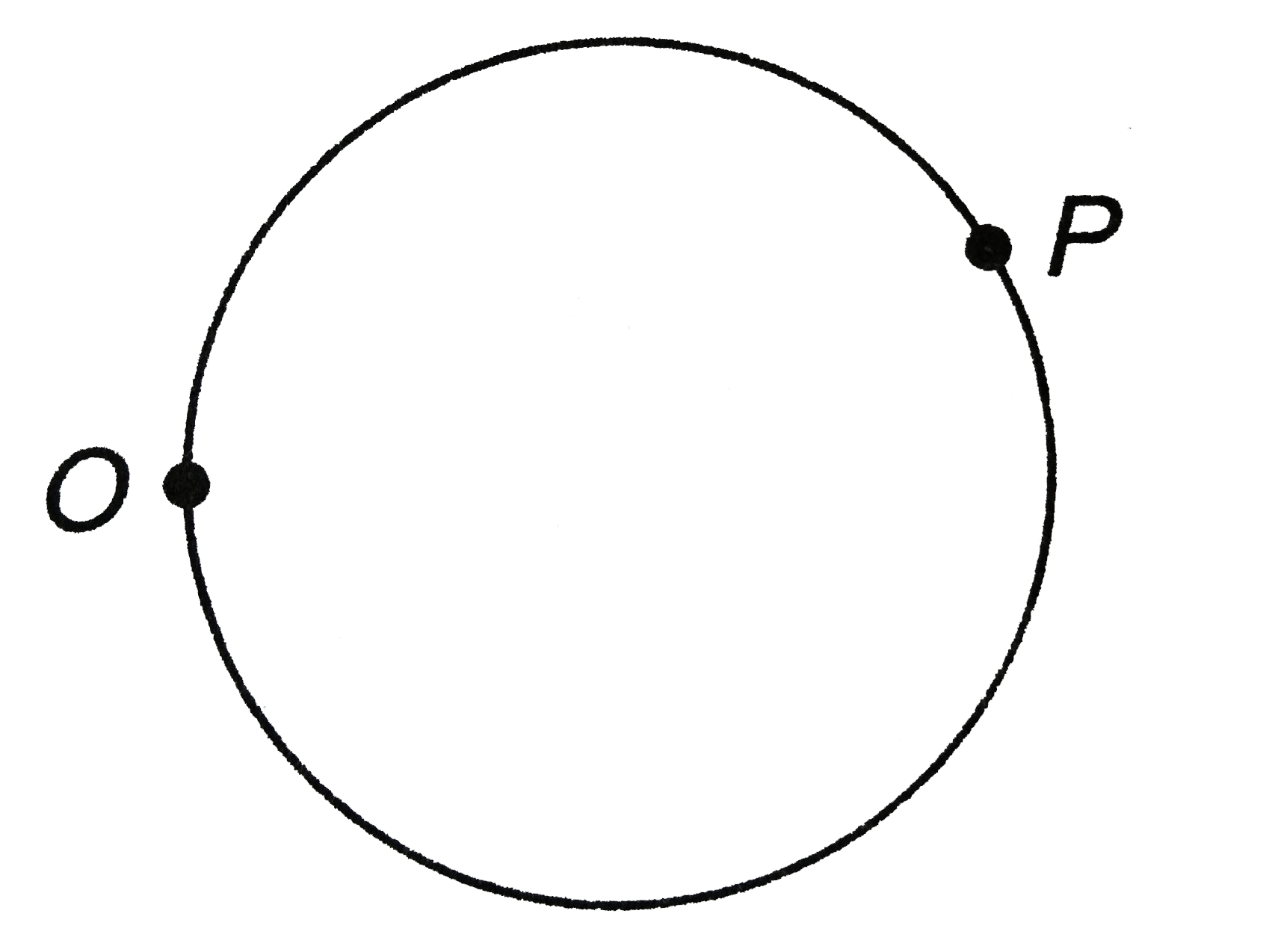
Particle P shown in figure is moving in a circle of radius R=10 cm with linear speed v=2m/s Find the angular speed of particle about point O.
View SolutionWhat will be the reading of spring balance in the figure shown in following situations ( g=10m/s2 )
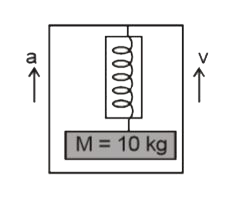
a=2m/s2,v=0
View SolutionA wheel of radius R=0.1m is rolling without slipping on a horizontal surface as shown in the firgure. Centre of the wheel moves with a constant speed √3m/s. The speed of the point P with respect to ground is
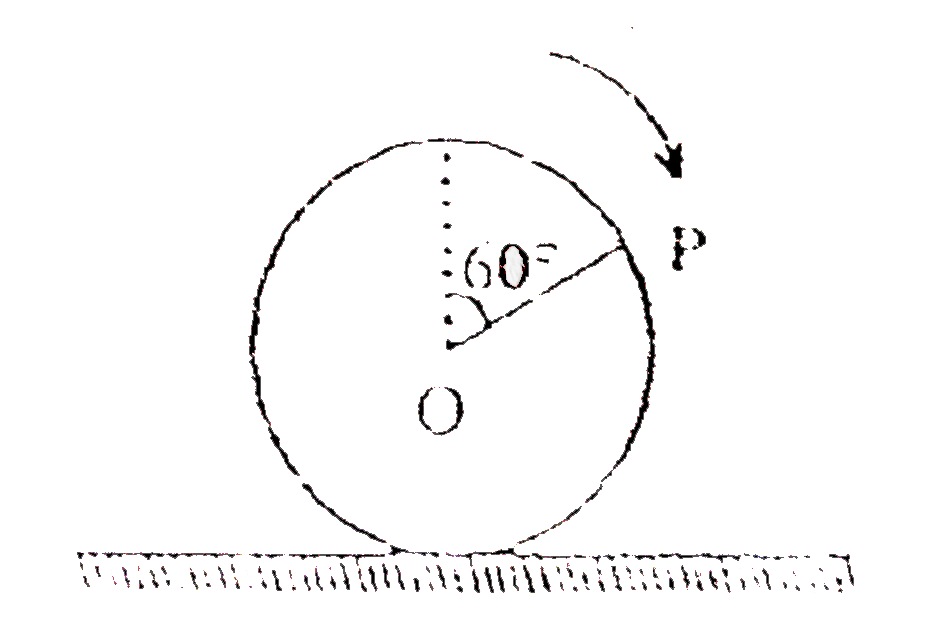
View SolutionA small sphere of mass 1 kg is rolling without slipping with linear speed
v=√2007m/s

It leaves the inclined plane at point C.
Find the linear speed at point C.
View SolutionA circular disc of mass 2 kg and radius 10 cm rolls without slipping with a speed 2 m/s. The total kinetic energy of disc is
View Solution