The figure shows a system consisting of (i) a ring the outer radius 3R rolling clockwise without slipping on a horizontal surface with angular speed $ω$ and (ii) an inner disc of radius 2R rotating anti clockwise with angular speed $ω / 2 .$ The ring and disc are separted. The point P on the inner disc is at a distance R from the origin, where OP makes an angle of $30^{\circ}$ with the horizontal. Then with respect to the horizontal surface,

The point O has a linear velocity $3 R ω ˆ i$

The point P has a linear velocity $\frac{11}{4} R ω ˆ i + \frac{\sqrt{3}}{4} R ω ˆ k$

the point P has a liner velocity $\frac{13}{4} R ω ˆ i + \frac{\sqrt{3}}{4} R ω ˆ k$

The point P has a linear velocity $(3 - \frac{\sqrt{3}}{4}) R ω ˆ i + \frac{1}{4} R ω ˆ k$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B

Updated on:21/07/2023

A ring and a disc of same mass roll without slipping along a horizontal surface with same velocity. If the $K . E .$ of ring is $8 J$ , then that of disc is
A $2 J$
B $4 J$
C $6 J$
D $16 J$
A thin circular ring of mass per unit length p and radius r is rotating at an angular speed $ω$ as shown in figure. The tension in the ring is

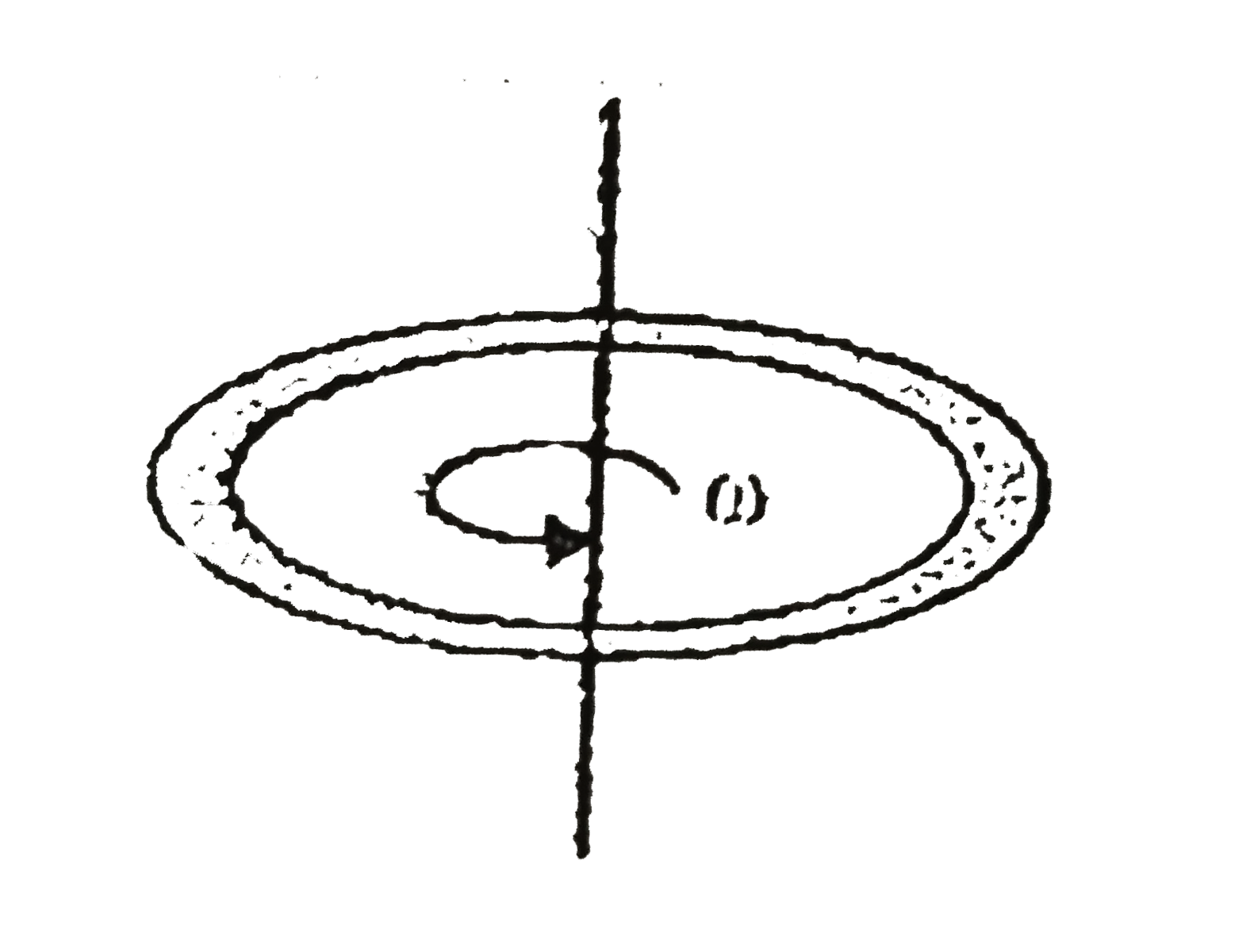A $p ω^{2} r^{2} / 2$
B $p ω^{2} r^{2}$
C $p ω^{2} r^{2} / 3$
DNone
A rough disc of mass $m$ rotates freely with an angular velocity $ω$ . If another rough disc of mass $\frac{m}{2}$ and same radius but spinning in opposite sense with angular speed $ω$ is kept on the first disc. Then:
Athe final angular speed of the dise is $\frac{ω}{3}$
Bthe net work does by friction is zero
Cthe friction does a positive work on the lighter disc
Dthe net work done by friction is $\frac{- m R^{2} ω^{2}}{3}$