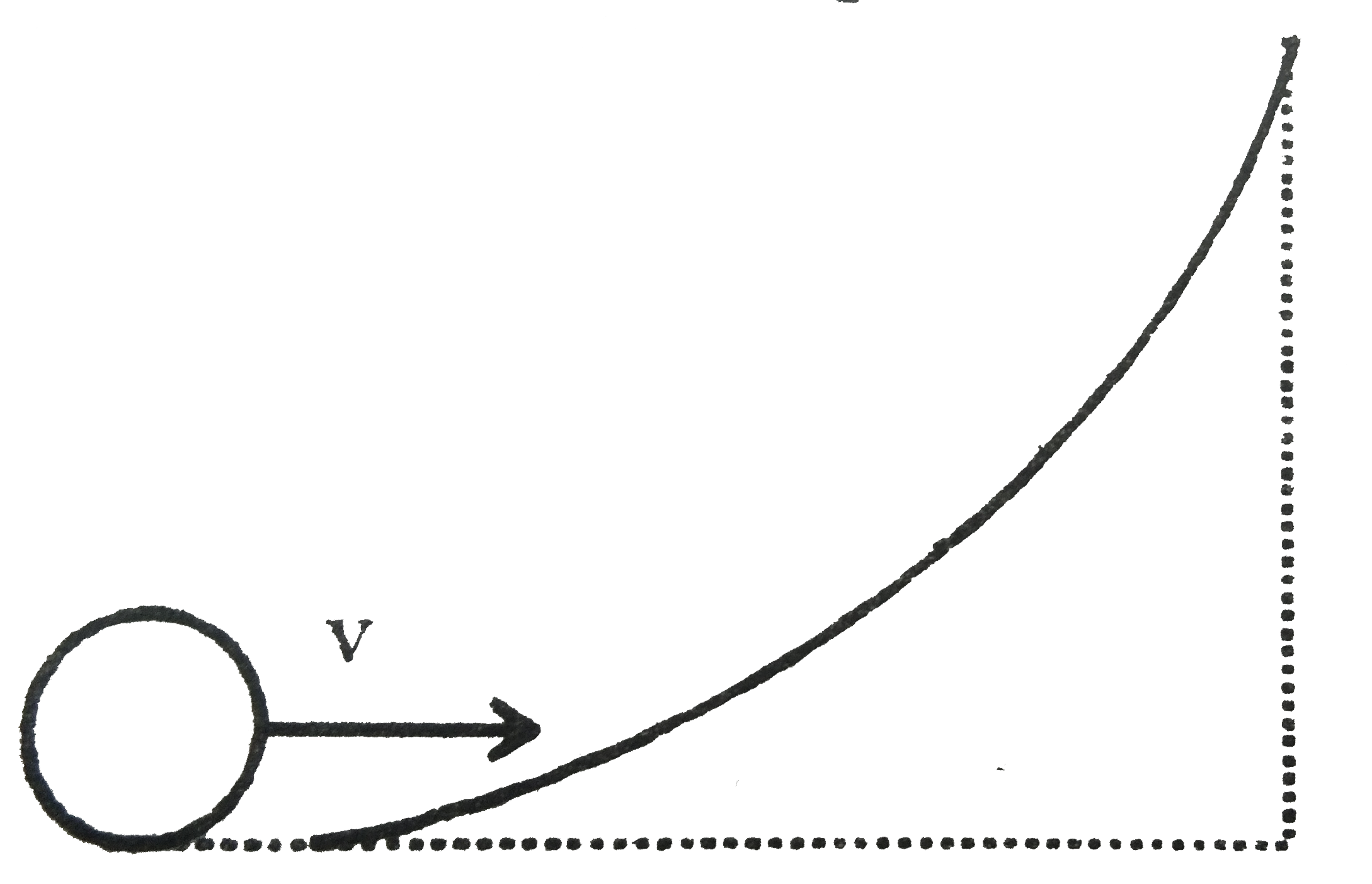
A small object of uniform density rolls up a curved surface with an initial velocity v. it reaches up to a maximum height of (3v^2)/(4g)
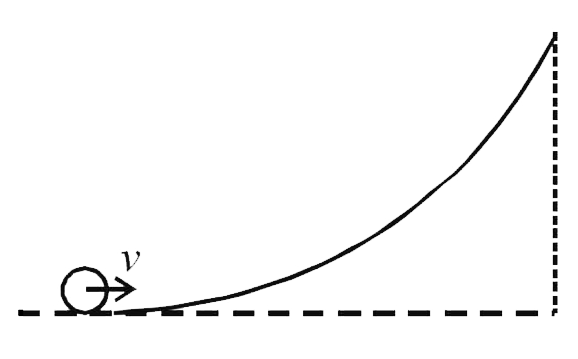
with respect to the initial position. The object is
solid sphere
hollow sphere
disc
ring
The correct Answer is:C
Let I be the moment of inertia of the body. Then
KE=12mV2+12Iω2 or K.E.=12mv2+12V2R2 or (ω=VR)
According to energy conservation, loss in kE = gain in PE
or 12(m+IR2)V2=mgh=mg(3v2)4g
Solving this, we get I=12mR2 i.e., the solid body is a disc.
Similar Questions
A small object of uniform density rolls up a curved surface with an initial velocity v. It reaches up to a maximum height of 5v2 / 6g with respect to the initial position. The object is- О. o ring O solid sphere O hollow sphere o disc
View SolutionWhen a body thrown vertically up with an initial velocity vo ,then maximum height reached by the body is
View Solution
Knowledge Check
Two discs A and B are mounted coaxially on a vertical axle. The discs have moments of inertia I and 2I respectively about the common axis. Disc A is imparted an initial angular velocity 2ω using the entire potential energy of a spring compressed by a distance x1. Disc B is imparted an angular velocity ω by a spring having the same spring constant and compressed by a distance x2. Both the discs rotate in the clockwise direction.
A small object of uniform density rolls up a curved surface with an initial velocitiy v. It reaches upto a maximum height of 3v24g with respect to the initial position. The object is:AringBsolid sphereChollow sphereDdiscA small object of uniform density rolls up a curved surface with an initial velocity v'. It reaches upto to maximum height of 3v24g with respect to the initial position. The object is
AringBsolid sphereChollow sphereDdiscA small object of uniform density rolls up a curved surface with an initial velocity 'v' . It reaches upto a maximum height of 3v24g with respect to the initial position . The object is
Ahollow sphereBdiscCringDsolid sphere
For a body thrown vertically up with an initial velocity u ,the maximum height reached by body from the ground is
View SolutionA small object of uniform density rolls up a curved surface with an initial velocity 'w'. It reaches up to a maximum height of 3u24g with respect to initial position. The object is:
View SolutionA small object of uniform density rolls up a curved surface with an initial velocity v. It reached upto maximum height of 3v2/4g with respect to the initial position. The object is -
 .
.
View SolutionAn object of radius R and mass M is rolling horizontally without slipping with speed v . It then rolls up the hill to a maximum height h=3v24g . The moment of inertia of the object is ( g = acceleration due to gravity)
View SolutionWhen a ball is thrown up vertically with velocity v0, it reaches a maximum height of h. If one wishes to triple the maximum height then the ball should be thrown with velocity
View Solution