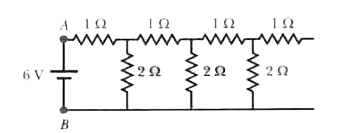
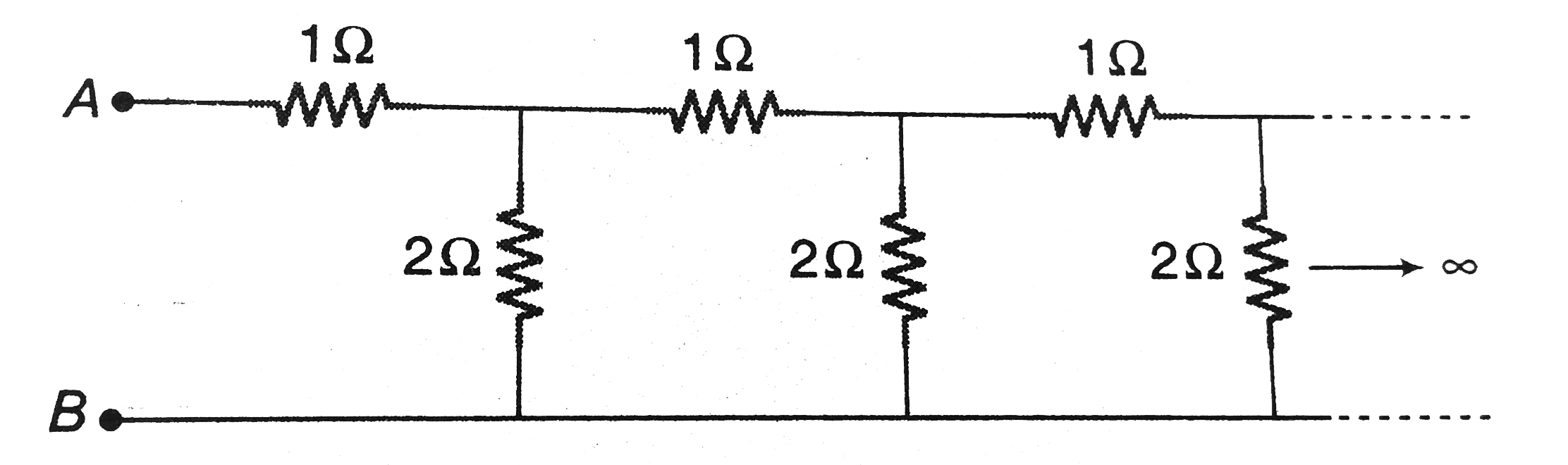
An infinite ladder is constructed with 1(Ω)and 2(Ω)resistor as shown in figure.(a)Find the effective resistance between the point A and B. (b) Find the current that passes through the (2Ω) resistor nearest to the battery.

Video Solution
Text Solution
(i) 2Ω (ii) 1.5 A
(i) Let RAB=x Then we can break one chain and connect a resistance of magnitude in place of it.Thus, the circuit remains as shown in figure Now, are in parallel. So, their combined resistance is 2x2+xorRAB=1+2x2+x
But RAB is assumed as x. Therefore x=1+2x2+x
Solving this equation ,we get x=2Ω
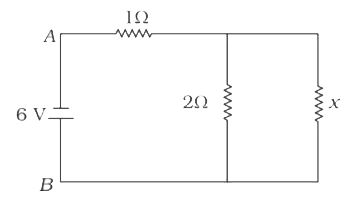
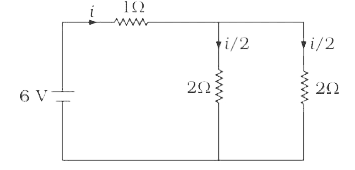
(ii) Net resistance of circuit R=1+2×22+2=2Ω
∴ Current through battery i=62=3A
The current is equally distributed in 2Ωand2Ω resistances , Therefore the desired current is i2or1.5A.
|
Similar Questions
Knowledge Check
In the figure shown the current through 2Ω resistor is
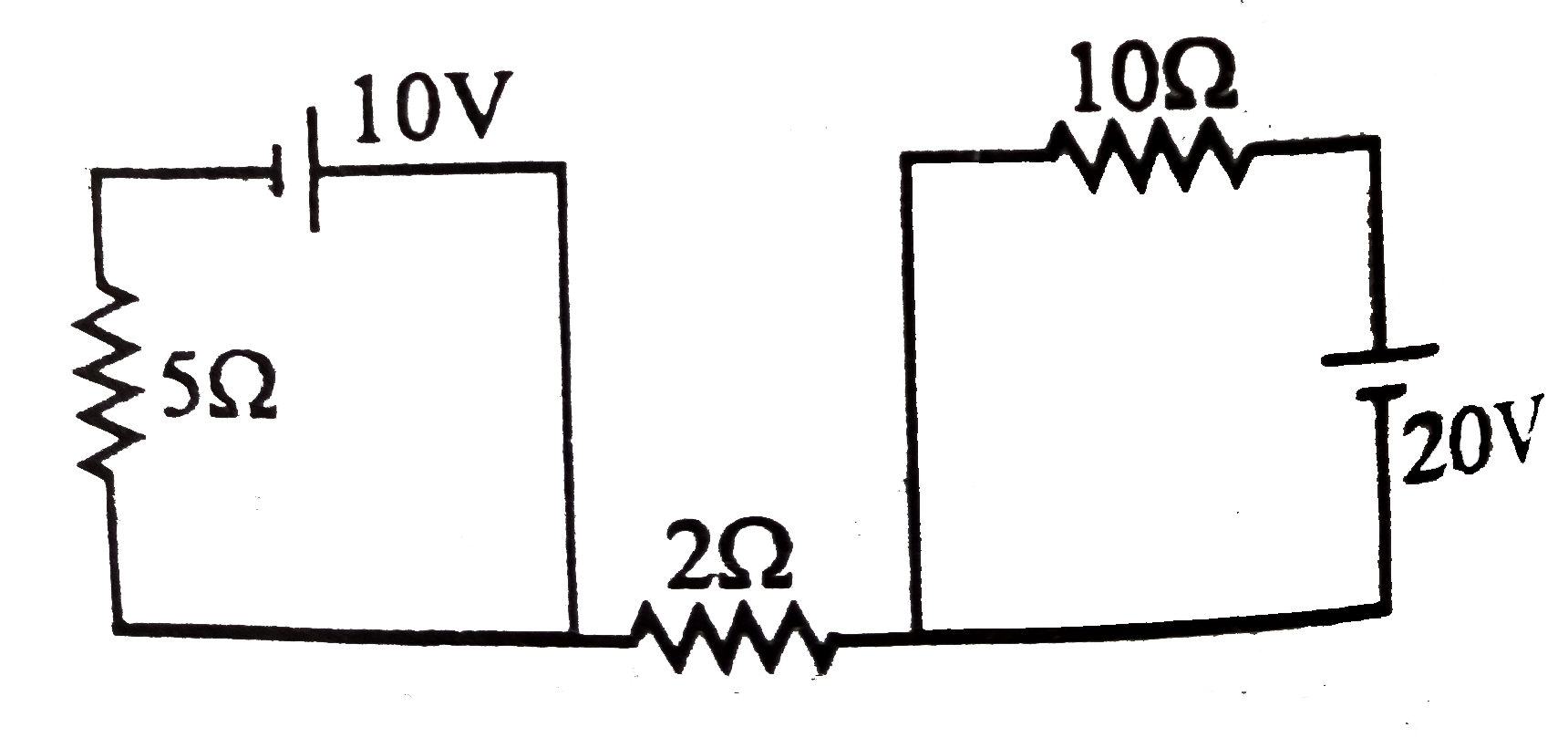 A2AB0AC4AD6A
A2AB0AC4AD6AIn the circuit shown in figure potential difference between point A and B is 16V. Find the current passing through 2Ω resistance.
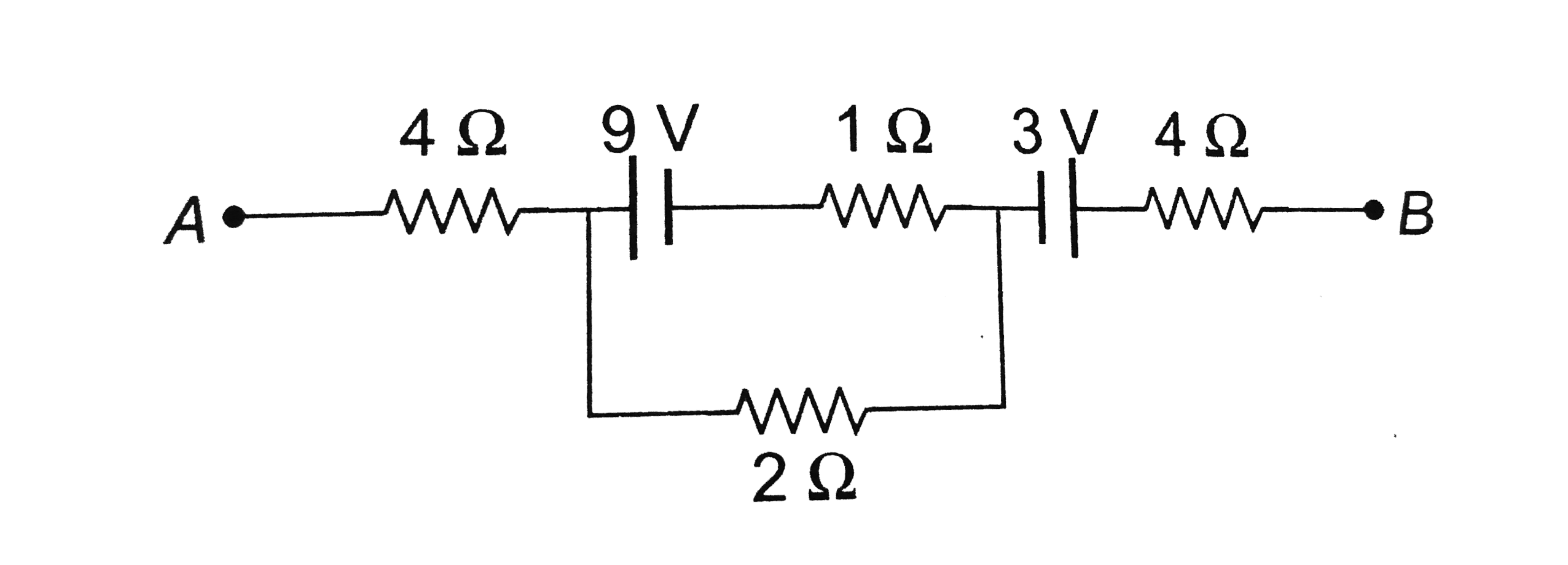 A1.5AB3.5AC1.0AD2.5A
A1.5AB3.5AC1.0AD2.5A
Find the current through the 10(Ω)resistor shown in figure.
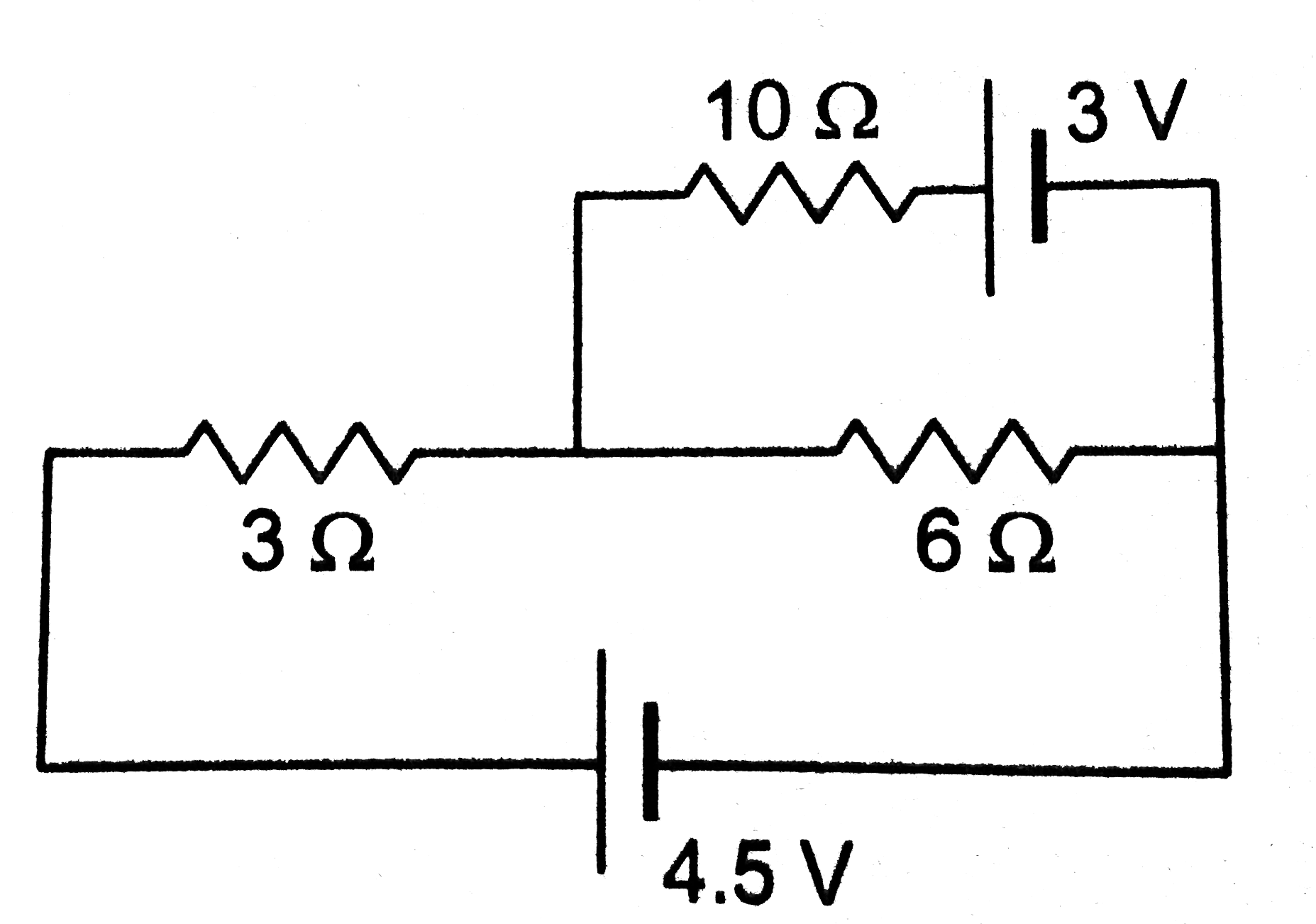
View SolutionFind the current through the 10Ω resistor shown in figure .
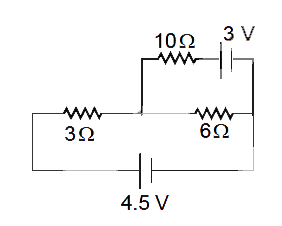
View Solution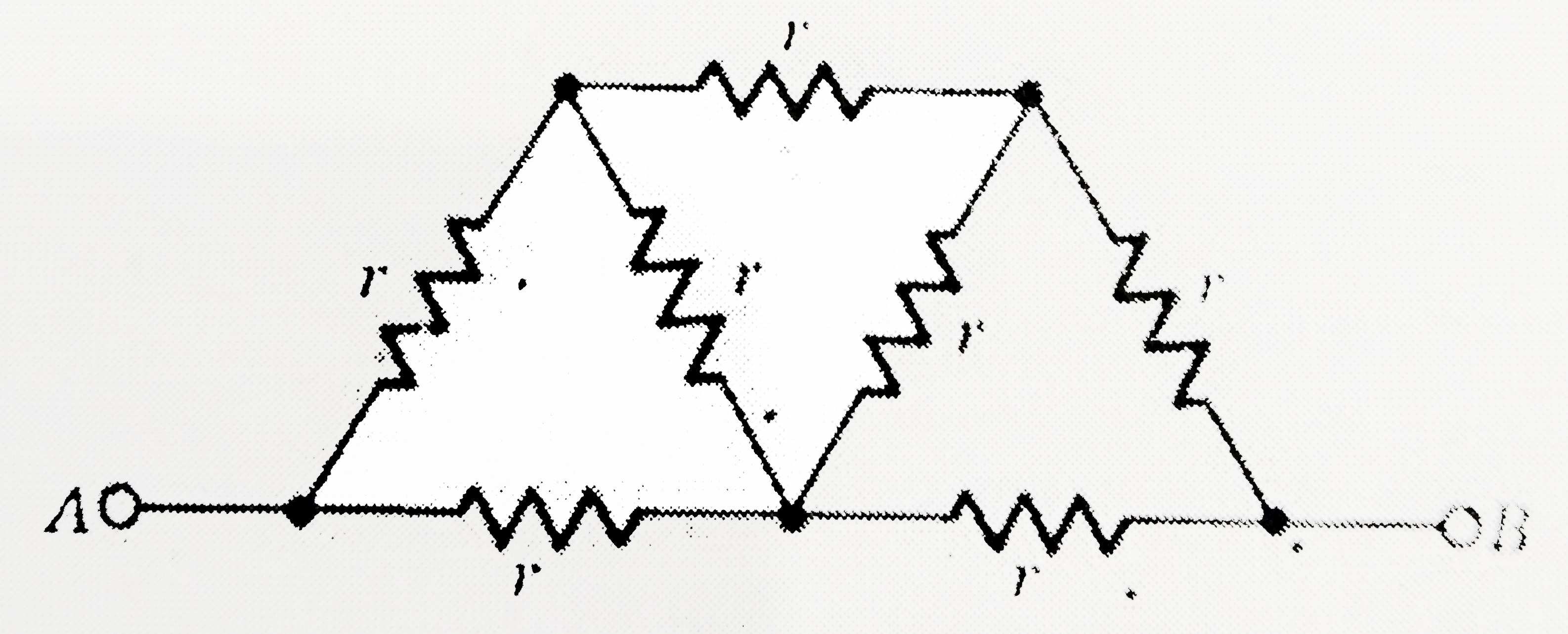
In the network as shown in figure. Each resistance r is of 2Ω find the effective resistance between points A and B.
View SolutionFind the current through 12Ω resistor in
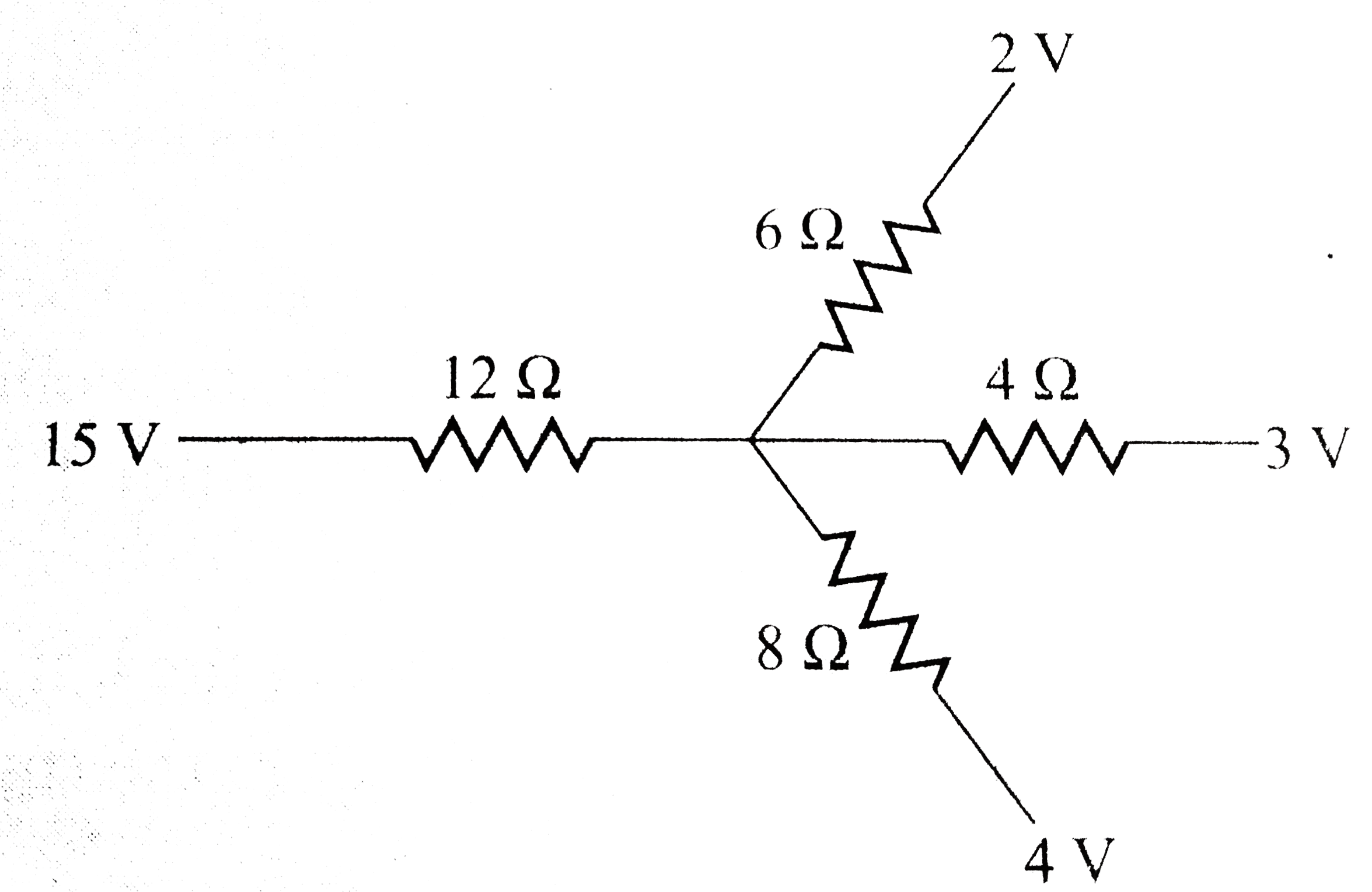
View SolutionFind the current through 23Ω resistance in the figure shown.
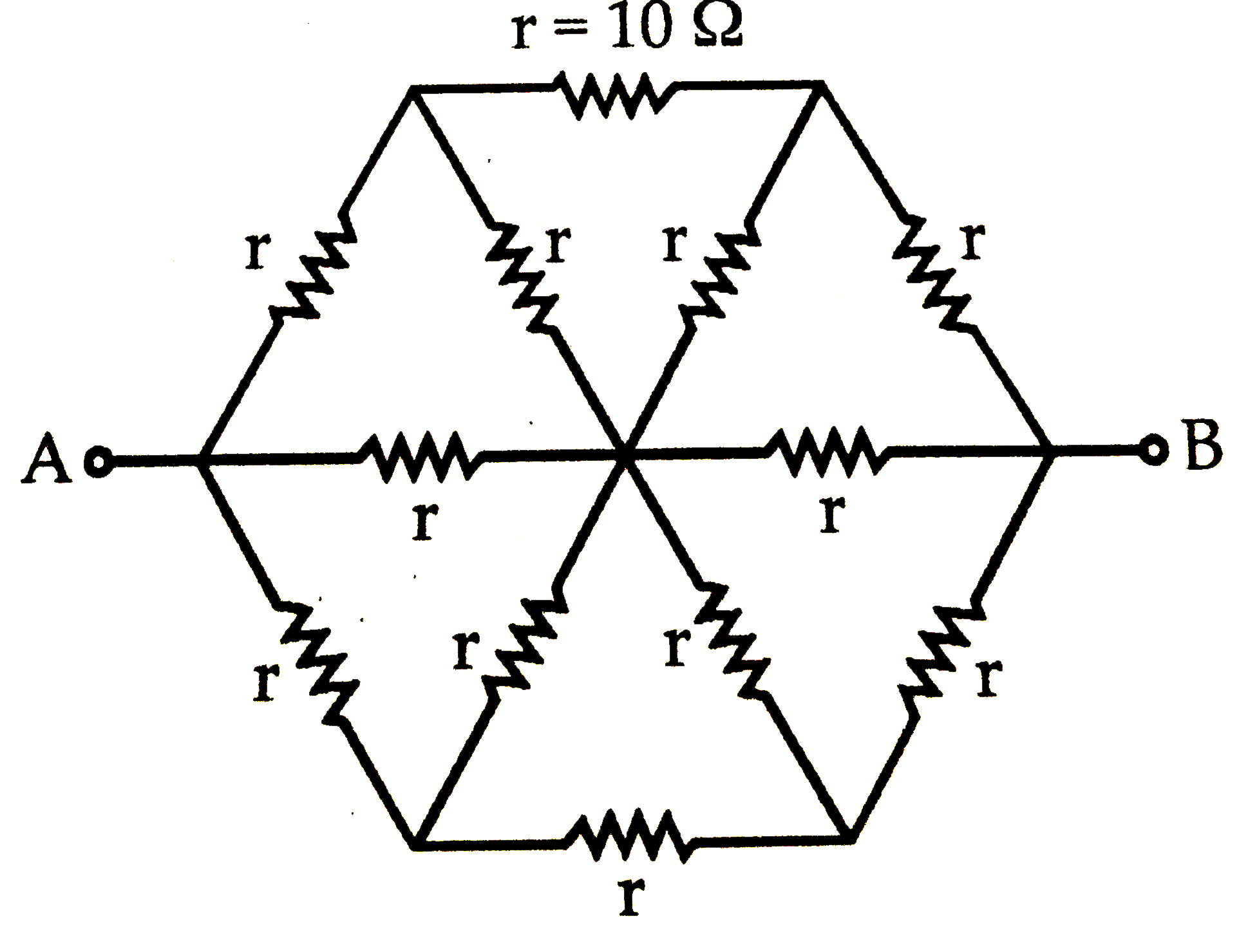
View Solution12 resistors each of 10Ω are connected as shown in the figure. The effective resistance between A and B is
View Solution