1<2x<2
Quantity AQuantity Bx5x7
Quantity A is greater.
Quantity B is greater.
The two quantities are equal.
The relationship cannot be determined from the infromation given.
The correct Answer is:A
To solve the inequality 1<2x<2 and compare the quantities x5 and x7, we can follow these steps:
Step 1: Solve the inequality
We start with the inequality:
1<2x<2
We can break this down into two parts.
1. From 1<2x:
12<x
2. From 2x<2:
x<1
Combining these results, we have:
12<x<1
Step 2: Choose a value for x
To compare x5 and x7, we can choose a value of x that lies within the interval (12,1). A suitable choice is:
x=34
Step 3: Calculate x5 and x7
Now we calculate x5 and x7 using x=34.
1. Calculate x5:
x5=(34)5=3545=2431024
2. Calculate x7:
x7=(34)7=3747=218716384
Step 4: Compare x5 and x7
We know that for 0<x<1, xn decreases as n increases. Therefore, since 5<7:
x5>x7
Conclusion
Thus, we conclude that:
Quantity A (x5)> Quantity B (x7)
Final Answer
The relationship is:
Quantity A>Quantity B
---
We start with the inequality:
1<2x<2
We can break this down into two parts.
12<x
x<1
12<x<1
To compare x5 and x7, we can choose a value of x that lies within the interval (12,1). A suitable choice is:
x=34
Now we calculate x5 and x7 using x=34.
x5=(34)5=3545=2431024
x7=(34)7=3747=218716384
We know that for 0<x<1, xn decreases as n increases. Therefore, since 5<7:
x5>x7
Thus, we conclude that:
Quantity A (x5)> Quantity B (x7)
The relationship is:
Quantity A>Quantity B
Similar Questions
Knowledge Check
Quantity AQuantity Bx22x
AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.Quantity AQuantity Bx3x−4
AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.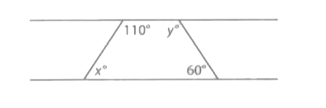
Quantity AQuantity By−x50AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.