x2+x−42=0
Quantity AQuantity B|x+1|5
Quantity A is greater.
Quantity B is greater.
The two quantities are equal.
The relationship cannot be determined from the infromation given.
The correct Answer is:A
To solve the equation x2+x−42=0 and compare the quantities |x+1| and 5, we will follow these steps:
Step 1: Solve the quadratic equation
The given equation is:
x2+x−42=0
We can factor this quadratic equation. We need to find two numbers that multiply to −42 (the product of A and C) and add up to 1 (the coefficient of B). The numbers that satisfy this are 7 and −6.
Step 2: Factor the equation
Using the numbers found, we can rewrite the equation:
x2+7x−6x−42=0
Now, we can group the terms:
(x2+7x)+(−6x−42)=0
Factoring by grouping gives us:
x(x+7)−6(x+7)=0
Factoring out the common term (x+7):
(x+7)(x−6)=0
Step 3: Find the roots
Setting each factor to zero gives us:
x+7=0⇒x=−7
x−6=0⇒x=6
Thus, the solutions to the equation are x=−7 and x=6.
Step 4: Calculate Quantity A
Now we need to evaluate |x+1| for both values of x.
1. For x=6:
|x+1|=|6+1|=|7|=7
2. For x=−7:
|x+1|=|−7+1|=|−6|=6
Step 5: Compare Quantity A and Quantity B
Now we compare the results with Quantity B, which is 5:
- When x=6, |x+1|=7 which is greater than 5.
- When x=−7, |x+1|=6 which is also greater than 5.
Conclusion
In both cases, |x+1|>5. Therefore, we conclude that:
Quantity A is greater than Quantity B.
Final Answer
The correct option is that Quantity A is greater than Quantity B.
---
The given equation is:
x2+x−42=0
We can factor this quadratic equation. We need to find two numbers that multiply to −42 (the product of A and C) and add up to 1 (the coefficient of B). The numbers that satisfy this are 7 and −6.
Using the numbers found, we can rewrite the equation:
x2+7x−6x−42=0
Now, we can group the terms:
(x2+7x)+(−6x−42)=0
Factoring by grouping gives us:
x(x+7)−6(x+7)=0
Factoring out the common term (x+7):
(x+7)(x−6)=0
Setting each factor to zero gives us:
x+7=0⇒x=−7
x−6=0⇒x=6
Thus, the solutions to the equation are x=−7 and x=6.
Now we need to evaluate |x+1| for both values of x.
|x+1|=|6+1|=|7|=7
|x+1|=|−7+1|=|−6|=6
Now we compare the results with Quantity B, which is 5:
- When x=6, |x+1|=7 which is greater than 5.
- When x=−7, |x+1|=6 which is also greater than 5.
In both cases, |x+1|>5. Therefore, we conclude that:
Quantity A is greater than Quantity B.
The correct option is that Quantity A is greater than Quantity B.
Similar Questions
Knowledge Check
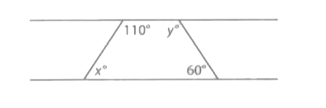
Quantity AQuantity By−x50AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.Quantity AQuantity Bx3x−4
AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.Quantity AQuantity Bx22x
AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.