xy>0
Quantity AQuantity B(x+y)2(x−y)2
Video Solution
Text Solution
The correct Answer is:Qunatity A is greater
To solve the problem, we need to compare the two quantities given:
- Quantity A: (x+y)2
- Quantity B: (x−y)2
Given that xy>0, this implies that both x and y are either both positive or both negative.
1. Expand Quantity A:
(x+y)2=x2+2xy+y2
2. Expand Quantity B:
(x−y)2=x2−2xy+y2
3. Compare the Two Quantities:
We need to compare:
x2+2xy+y2(Quantity A)
and
x2−2xy+y2(Quantity B)
4. Subtract Quantity B from Quantity A:
(x+y)2−(x−y)2=(x2+2xy+y2)−(x2−2xy+y2)
Simplifying this gives:
=2xy+2xy=4xy
5. Analyze the Result:
Since xy>0, it follows that 4xy>0. This means:
(x+y)2>(x−y)2
6. Conclusion:
Therefore, Quantity A is greater than Quantity B:
Quantity A>Quantity B
Final Answer:
Quantity A is greater than Quantity B.
---
- Quantity B: (x−y)2
(x+y)2=x2+2xy+y2
(x−y)2=x2−2xy+y2
We need to compare:
x2+2xy+y2(Quantity A)
and
x2−2xy+y2(Quantity B)
(x+y)2−(x−y)2=(x2+2xy+y2)−(x2−2xy+y2)
Simplifying this gives:
=2xy+2xy=4xy
Since xy>0, it follows that 4xy>0. This means:
(x+y)2>(x−y)2
Therefore, Quantity A is greater than Quantity B:
Quantity A>Quantity B
Quantity A is greater than Quantity B.
|
Updated on:7/8/2024
Similar Questions
Knowledge Check
- Question 1 - Select One
Quantity AQuantity Bx22x
AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given. - Question 2 - Select One
xy>0
Quantity AQuantity Bx+y0AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given. - Question 3 - Select One
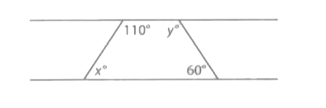
Quantity AQuantity By−x50AQuantity A is greater.BQuantity B is greater.CThe two quantities are equal.DThe relationship cannot be determined from the infromation given.