The Ksp for the following dissociation is 1.6×10−5
PbCl2(s)→Pb2+(aq)+2Cl−(aq) Which of the following choices is correct for a mixture of 300 mL 0.134 M Pb(NO3)2 and 100 mL 0.4 M NaCl?
Q>Ksp
Q=Ksp
Not enough data provided
Q<Ksp
The correct Answer is:A
To solve the problem, we need to determine whether a precipitate of lead(II) chloride (PbCl₂) will form when mixing the given solutions. We will follow these steps:
Step 1: Calculate the moles of Pb²⁺ and Cl⁻ ions in the mixture.
1. Calculate moles of Pb²⁺ from Pb(NO₃)₂:
- Volume = 300 mL = 0.300 L
- Molarity = 0.134 M
- Moles of Pb²⁺ = Molarity × Volume = 0.134 mol/L × 0.300 L = 0.0402 moles
2. Calculate moles of Cl⁻ from NaCl:
- Volume = 100 mL = 0.100 L
- Molarity = 0.4 M
- Moles of Cl⁻ = Molarity × Volume = 0.4 mol/L × 0.100 L = 0.040 moles
Step 2: Calculate the total volume of the mixture.
- Total Volume = Volume of Pb(NO₃)₂ + Volume of NaCl = 300 mL + 100 mL = 400 mL = 0.400 L
Step 3: Calculate the concentrations of Pb²⁺ and Cl⁻ in the mixture.
1. Concentration of Pb²⁺:
Concentration of Pb2+=Moles of Pb2+Total Volume=0.0402 moles0.400 L=0.1005 M
2. Concentration of Cl⁻:
Concentration of Cl−=Moles of Cl−Total Volume=0.040 moles0.400 L=0.100 M
Step 4: Calculate the ionic product (Q) for PbCl₂.
The dissociation of PbCl₂ can be represented as:
PbCl2(s)⇌Pb2+(aq)+2Cl−(aq)
The ionic product (Q) is given by:
Q=(Pb2+)(Cl−)2
Substituting the concentrations:
Q=(0.1005)(0.100)2=(0.1005)(0.01)=0.001005=1.005×10−3
Step 5: Compare Q with Ksp.
Given Ksp for PbCl₂ is 1.6×10−5.
- Since Q=1.005×10−3 and Ksp=1.6×10−5:
Q>Ksp
Step 6: Conclusion.
Since Q is greater than Ksp, a precipitate of PbCl₂ will form.
Final Answer:
A precipitate of PbCl₂ will form.
---
- Volume = 300 mL = 0.300 L
- Molarity = 0.134 M
- Moles of Pb²⁺ = Molarity × Volume = 0.134 mol/L × 0.300 L = 0.0402 moles
- Volume = 100 mL = 0.100 L
- Molarity = 0.4 M
- Moles of Cl⁻ = Molarity × Volume = 0.4 mol/L × 0.100 L = 0.040 moles
Concentration of Pb2+=Moles of Pb2+Total Volume=0.0402 moles0.400 L=0.1005 M
Concentration of Cl−=Moles of Cl−Total Volume=0.040 moles0.400 L=0.100 M
PbCl2(s)⇌Pb2+(aq)+2Cl−(aq)
Q=(Pb2+)(Cl−)2
Substituting the concentrations:
Q=(0.1005)(0.100)2=(0.1005)(0.01)=0.001005=1.005×10−3
Q>Ksp
A precipitate of PbCl₂ will form.
Similar Questions
Knowledge Check
The Ksp for the following dissociation is 2.2×10−2
PbCl2(s)fPb2+(aq)+2Cl−(aq)
Which of the following choices is correct for a mixture of 350mL 0.225M Pb(NO3)2 and 200 mL 0.6 M NaCl ?AQ>KspBQ=KspCNot enough data providedDQ<Ksp[Cl−] in a mixture of 200mL of 0.01 M HCl and 100 mL of 0.01 M BaCl2 is
A0.01M NaHCO−3B0.0133MC0.03MD0.02MKsp of PbCl2=1.6×10−5 On mixing 300mL,0.134MPb(NO3)2(aq.)+100mL,0.4MNaCl(aq.)
AQ>KspBQ<KspCQ=KspDRelation does not exit
How many mL of 2.0 M Pb(NO3)2 , contains 600 mg Pb2+.
View SolutionThe normality of mixture obtained by mixing 100 ml of 0.2 M H2SO4+100 ml 0.2 M NaOH is
View Solution500 ml, 1 M NaCl(aq) solution is mixed with 1000 ml, 1 M AgNO3(aq) solution. Which of the following option is correct for resultant solution?
View SolutionWhat is the maximum mass of PbI2 that can be precipitated by mixing 25.0 mL of 0.100 M Pb(NO3)2 with 35.0 mL of 0.100 M NaI?
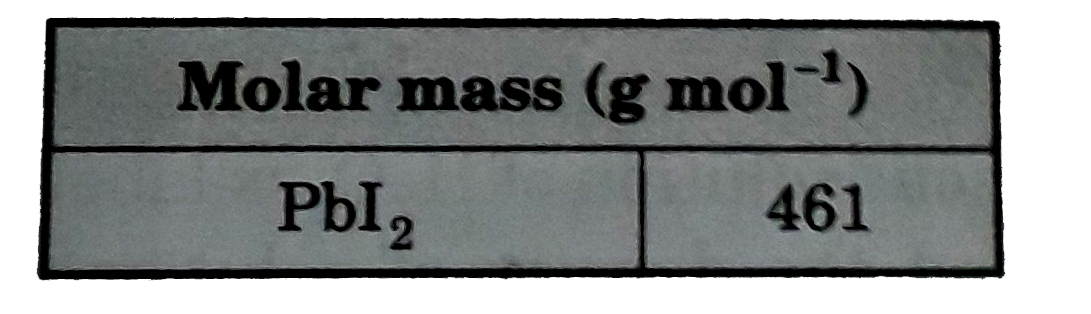
View SolutionWhat is the final [Na+] in a solution prepared by mixing 70.0mL of 3.00 M Na2SO4 with 30.0 mL of 1.00 M NaCl?
View Solution

